Skills Review for Linear Approximations and Differentials

Learning Outcomes

  • Evaluate trigonometric functions using the unit circle

In the Linear Approximations and Differentials section, we will sometimes be asked to evaluate the derivatives of trigonometric functions at specific values. Here we will review how to evaluate trigonometric functions at various angle measures.

Evaluate Trigonometric Functions

(also in Module 1, Skills Review for Trigonometric Functions and Module 3, Skills Review for Derivatives of Trigonometric Functions) – but slightly different content below

The unit circle tells us the value of cosine and sine at any of the given angle measures seen below. The first coordinate in each ordered pair is the value of cosine at the given angle measure, while the second coordinate in each ordered pair is the value of sine at the given angle measure. You have learned that all trigonometric functions can be written in terms of sine and cosine. Thus, if you can evaluate sine and cosine at various angle values, you can also evaluate the other trigonometric functions at various angle values. Take time to learn the [latex]\left(x,y\right)[/latex] coordinates of all of the major angles in the first quadrant of the unit circle.

Remember, every angle in quadrant two, three, or four has a reference angle that lies in quadrant one. The quadrant of the original angle only affects the sign (positive or negative) of a trigonometric function’s value at a given angle.

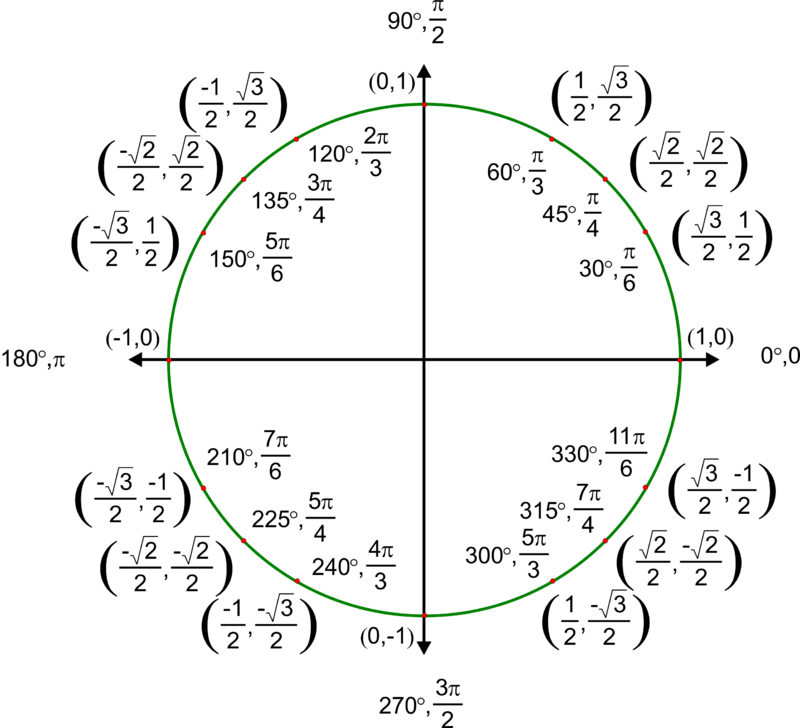
f-d-43392176e093fa07f39e1f3687226d4d751b809be928c16abac5dcb3+IMAGE+IMAGE

A General Note: Evaluating Tangent, Secant, Cosecant, and Cotangent Functions

If [latex]\theta[/latex] is an angle measure, then, using the unit circle,

[latex]\begin{gathered}\tan \theta=\frac{sin \theta}{cos \theta}\\ \sec \theta=\frac{1}{cos \theta}\\ \csc t=\frac{1}{sin \theta}\\ \cot \theta=\frac{cos \theta}{sin \theta}\end{gathered}[/latex]

Example: Using the Unit Circle to Find the Value of Trigonometric Functions

Find [latex]\sin \theta,\cos \theta,\tan \theta,\sec \theta,\csc \theta[/latex], and [latex]\cot \theta[/latex] when [latex]\theta=\frac{\pi }{3}[/latex].

Try It

Below are the values of all six trigonometric functions evaluated at common angle measures from Quadrant I.

Angle [latex]0[/latex] [latex]\frac{\pi }{6},\text{ or }{30}^{\circ}[/latex] [latex]\frac{\pi }{4},\text{ or } {45}^{\circ }[/latex] [latex]\frac{\pi }{3},\text{ or }{60}^{\circ }[/latex] [latex]\frac{\pi }{2},\text{ or }{90}^{\circ }[/latex]
Cosine 1 [latex]\frac{\sqrt{3}}{2}[/latex] [latex]\frac{\sqrt{2}}{2}[/latex] [latex]\frac{1}{2}[/latex] 0
Sine 0 [latex]\frac{1}{2}[/latex] [latex]\frac{\sqrt{2}}{2}[/latex] [latex]\frac{\sqrt{3}}{2}[/latex] 1
Tangent 0 [latex]\frac{\sqrt{3}}{3}[/latex] 1 [latex]\sqrt{3}[/latex] Undefined
Secant 1 [latex]\frac{2\sqrt{3}}{3}[/latex] [latex]\sqrt{2}[/latex] 2 Undefined
Cosecant Undefined 2 [latex]\sqrt{2}[/latex] [latex]\frac{2\sqrt{3}}{3}[/latex] 1
Cotangent Undefined [latex]\sqrt{3}[/latex] 1 [latex]\frac{\sqrt{3}}{3}[/latex] 0