Learning Outcomes
- Describe the velocity and acceleration vectors of a particle moving in space.
Our starting point is using vector-valued functions to represent the position of an object as a function of time. All of the following material can be applied either to curves in the plane or to space curves. For example, when we look at the orbit of the planets, the curves defining these orbits all lie in a plane because they are elliptical. However, a particle traveling along a helix moves on a curve in three dimensions.
Definition
Let [latex]{\bf{r}}\,(t)[/latex] be a twice-differentiable vector-valued function of the parameter [latex]t[/latex] that represents the position of an object as a function of time. The velocity vector [latex]{\bf{v}}\,(t)[/latex] of the object is given by
[latex]\text{Velocity}={\bf{v}}\,(t)={\bf{r}}'\,(t).[/latex]
The acceleration vector [latex]{\bf{a}}\,(t)[/latex] is defined to be
[latex]\text{Acceleration}={\bf{a}}\,(t)={\bf{v}}'\,(t)={\bf{r}}''\,(t).[/latex]
The speed is defined to be
[latex]\text{Speed}=v\,(t)=\left\Vert{\bf{v}}\,(t)\right\Vert=\left\Vert{\bf{r}}'\,(t)\right\Vert=\frac{ds}{dt}.[/latex]
Since [latex]{\bf{r}}\,(t)[/latex] can be in either two or three dimensions, these vector-valued functions can have either two or three components. In two dimensions, we define [latex]{\bf{r}}\,(t)=x\,(t)\,{\bf{i}}+y\,(t)\,{\bf{j}}[/latex] and in three dimensions [latex]{\bf{r}}\,(t)=x\,(t)\,{\bf{i}}+y\,(t)\,{\bf{j}}+z\,(t)\,{\bf{k}}[/latex]. Then the velocity, acceleration, and speed can be written as shown in the following table.
| Quantity | Two Dimensions | Three Dimensions |
| Position | [latex]\large{{\bf{r}}\,(t)=x\,(t)\,{\bf{i}}+y\,(t)\,{\bf{j}}}[/latex] | [latex]\large{{\bf{r}}\,(t)=x\,(t)\,{\bf{i}}+y\,(t)\,{\bf{j}}+z\,(t)\,{\bf{k}}}[/latex] |
| Velocity | [latex]\large{{\bf{v}}\,(t)=x'\,(t)\,{\bf{i}}+y'\,(t)\,{\bf{j}}}[/latex] | [latex]\large{{\bf{v}}\,(t)=x'\,(t)\,{\bf{i}}+y'\,(t)\,{\bf{j}}+z'\,(t)\,{\bf{k}}}[/latex] |
| Acceleration | [latex]\large{{\bf{a}}\,(t)=x''\,(t)\,{\bf{i}}+y''\,(t)\,{\bf{j}}}[/latex] | [latex]\large{{\bf{a}}\,(t)=x''\,(t)\,{\bf{i}}+y''\,(t)\,{\bf{j}}+z''\,(t)\,{\bf{k}}}[/latex] |
| Speed | [latex]\large{v\,(t)=\sqrt{(x'\,(t))^{2}+(y'\,(t))^{2}}}[/latex] | [latex]\large{v\,(t)=\sqrt{(x'\,(t))^{2}+(y'\,(t))^{2}+(z'\,(t))^{2}}}[/latex] |
Example: Studying Motion Along a PArabola
A particle moves in a parabolic path defined by the vector-valued function [latex]{\bf{r}}\,(t)=t^{2}\,{\bf{i}}+\sqrt{5-t^{2}}\,{\bf{j}}[/latex], where [latex]t[/latex] measures time in seconds.
try it
A particle moves in a path defined by the vector-valued function [latex]{\bf{r}}\,(t)=(t^{2}-3t)\,{\bf{i}}+(2t-4)\,{\bf{j}}+(t+2)\,{\bf{k}}[/latex], where [latex]t[/latex] measures time in seconds and where distance is measured in feet. Find the velocity, acceleration, and speed as functions of time.
Watch the following video to see the worked solution to the above Try It
To gain a better understanding of the velocity and acceleration vectors, imagine you are driving along a curvy road. If you do not turn the steering wheel, you would continue in a straight line and run off the road. The speed at which you are traveling when you run off the road, coupled with the direction, gives a vector representing your velocity, as illustrated in the following figure.
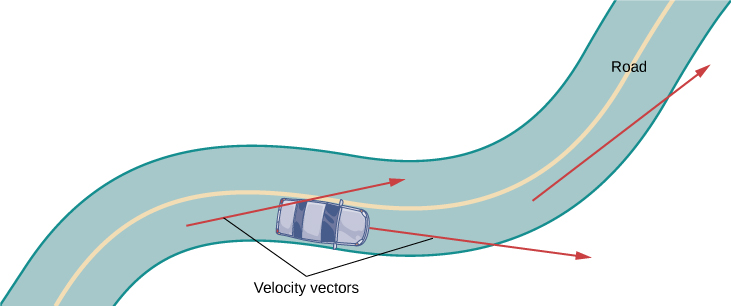
Figure 2. At each point along a road traveled by a car, the velocity vector of the car is tangent to the path traveled by the car.
However, the fact that you must turn the steering wheel to stay on the road indicates that your velocity is always changing (even if your speed is not) because your direction is constantly changing to keep you on the road. As you turn to the right, your acceleration vector also points to the right. As you turn to the left, your acceleration vector points to the left. This indicates that your velocity and acceleration vectors are constantly changing, regardless of whether your actual speed varies (Figure 3).

Figure 3. The dashed line represents the trajectory of an object (a car, for example). The acceleration vector points toward the inside of the turn at all times.
Candela Citations
- CP 3.14. Authored by: Ryan Melton. License: CC BY: Attribution
- Calculus Volume 3. Authored by: Gilbert Strang, Edwin (Jed) Herman. Provided by: OpenStax. Located at: https://openstax.org/books/calculus-volume-3/pages/1-introduction. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike. License Terms: Access for free at https://openstax.org/books/calculus-volume-3/pages/1-introduction

