Learning Objectives
- Use a double integral to calculate the area of a region, volume under a surface, or average value of a function over a plane region.
Double integrals are very useful for finding the area of a region bounded by curves of functions. We describe this situation in more detail in the next section. However, if the region is a rectangular shape, we can find its area by integrating the constant function [latex]f(x,y) = 1[/latex] over the region [latex]R[/latex].
definition
The area of the region [latex]R[/latex] is given by [latex]A(R) = \underset{R}{\displaystyle\iint}1dA[/latex].
This definition makes sense because using [latex]f(x,y) = 1[/latex] and evaluating the integral make it a product of length and width. Let’s check this formula with an example and see how this works.
Example: finding area using a double integral
Find the area of the region [latex]{R} = {\left \{ (x,y) \mid 0 \leq x \leq 3,0 \leq y \leq 2 \right \}}[/latex] by using a double integral, that is, by integrating [latex]1[/latex] over the region [latex]R[/latex].
We have already seen how double integrals can be used to find the volume of a solid bounded above by a function [latex]f(x,y)[/latex] over a region [latex]R[/latex] provided [latex]f(x,y)\geq 0[/latex] for all [latex](x,y)[/latex] in [latex]R[/latex]. Here is another example to illustrate this concept.
Example: Volume of an elliptic paraboloid
Find the volume [latex]V[/latex] of the solid [latex]S[/latex] that is bounded by the elliptic paraboloid [latex]2x^2+y^2+z=27[/latex], the planes [latex]x = 3[/latex] and [latex]y = 3[/latex], and the three coordinate planes.
try it
Find the volume of the solid bounded above by the graph of [latex]f(x,y) = xy\sin(x^2y)[/latex] and below by the [latex]xy[/latex]-plane on the rectangular region [latex]R = [0,1] \times [0, \pi][/latex].
Watch the following video to see the worked solution to the above Try It
Try It
Recall that we defined the average value of a function of one variable on an interval [latex][a,b][/latex] as
[latex]\large{{f_{\text{ave}}} = {\dfrac{1}{b-a}}\displaystyle\int_a^b{f(x)dx}}[/latex].
Similarly, we can define the average value of a function of two variables over a region [latex]R[/latex]. The main difference is that we divide by an area instead of the width of an interval.
definition
The average value of a function of two variables over a region [latex]R[/latex] is
[latex]\large{{f_{\text{ave}}} = {\dfrac{1}{\text{Area }R}} \underset{R}{\displaystyle\iint}{f(x,y)dA}}[/latex]
In the next example we find the average value of a function over a rectangular region. This is a good example of obtaining useful information for an integration by making individual measurements over a grid, instead of trying to find an algebraic expression for a function.
Example: calculating average storm rainfall
The weather map in Figure 2 shows an unusually moist storm system associated with the remnants of Hurricane Karl, which dumped 4–8 inches (100–200 mm) of rain in some parts of the Midwest on September 22–23, 2010. The area of rainfall measured 300 miles east to west and 250 miles north to south. Estimate the average rainfall over the entire area in those two days.

Figure 2. Effects of Hurricane Karl, which dumped 4–8 inches (100–200 mm) of rain in some parts of southwest Wisconsin, southern Minnesota, and southeast South Dakota over a span of 300 miles east to west and 250 miles north to south.
try it
A contour map is shown for a function [latex]f(x,y)[/latex] on the rectangle [latex]{R} = {[-3,6]} {\times} {[-1,4]}[/latex].
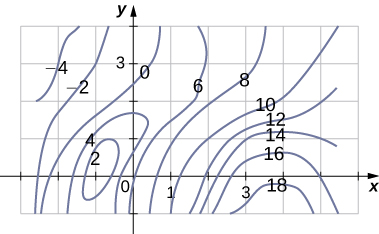
Figure 4.
- Use the midpoint rule with [latex]m = 3[/latex] and [latex]n = 2[/latex] to estimate the value of [latex]\underset{R}{\displaystyle\iint}{f(x,y)dA}[/latex].
- Estimate the average value of the function [latex]f(x,y)[/latex].
Candela Citations
- CP 5.5. Authored by: Ryan Melton. License: CC BY: Attribution
- Calculus Volume 3. Authored by: Gilbert Strang, Edwin (Jed) Herman. Provided by: OpenStax. Located at: https://openstax.org/books/calculus-volume-3/pages/1-introduction. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike. License Terms: Access for free at https://openstax.org/books/calculus-volume-3/pages/1-introduction


