1. Draw an angle in standard position. Label the vertex, initial side, and terminal side.
2. Explain why there are an infinite number of angles that are coterminal to a certain angle.
3. State what a positive or negative angle signifies, and explain how to draw each.
4. How does radian measure of an angle compare to the degree measure? Include an explanation of 1 radian in your paragraph.
5. Explain the differences between linear speed and angular speed when describing motion along a circular path.
For the following exercises, draw an angle in standard position with the given measure.
6. 30°
7. 300°
8. −80°
9. 135°
10. −150°
11. [latex]\frac{2\pi }{3}[/latex]
12. [latex]\frac{7\pi }{4}[/latex]
13. [latex]\frac{5\pi }{6}[/latex]
14. [latex]\frac{\pi }{2}[/latex]
15. [latex]-\frac{\pi }{10}[/latex]
16. 415°
17. −120°
18. −315°
19. [latex]\frac{22\pi }{3}[/latex]
20. [latex]-\frac{\pi }{6}[/latex]
21. [latex]-\frac{4\pi }{3}[/latex]
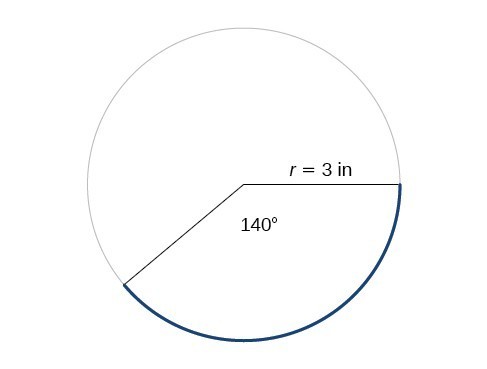
Figure 24
For the following exercises, refer to Figure 24. Round to two decimal places.
22. Find the arc length.
23. Find the area of the sector.
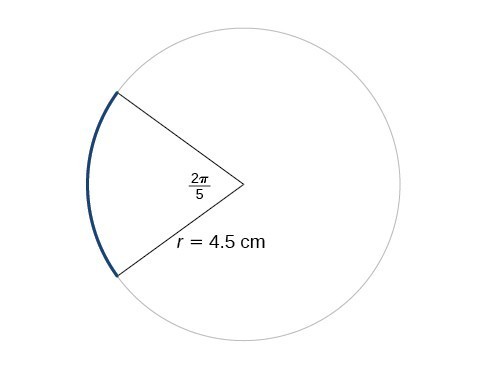
Figure 25
For the following exercises, refer to Figure 25. Round to two decimal places.
24. Find the arc length.
25. Find the area of the sector.
For the following exercises, convert angles in radians to degrees.
26. [latex]\frac{3\pi }{4}[/latex] radians
27. [latex]\frac{\pi }{9}[/latex] radians
28. [latex]-\frac{5\pi }{4}[/latex] radians
29. [latex]\frac{\pi }{3}[/latex] radians
30. [latex]-\frac{7\pi }{3}[/latex] radians
31. [latex]-\frac{5\pi }{12}[/latex] radians
32. [latex]\frac{11\pi }{6}[/latex] radians
For the following exercises, convert angles in degrees to radians.
33. 90°
34. 100°
35. −540°
36. −120°
37. 180°
38. −315°
39. 150°
For the following exercises, use to given information to find the length of a circular arc. Round to two decimal places.
40. Find the length of the arc of a circle of radius 12 inches subtended by a central angle of [latex]\frac{\pi }{4}[/latex] radians.
41. Find the length of the arc of a circle of radius 5.02 miles subtended by the central angle of [latex]\frac{\pi }{3}[/latex].
42. Find the length of the arc of a circle of diameter 14 meters subtended by the central angle of [latex]\frac{5\pi }{6}[/latex].
43. Find the length of the arc of a circle of radius 10 centimeters subtended by the central angle of 50°.
44. Find the length of the arc of a circle of radius 5 inches subtended by the central angle of 220°.
45. Find the length of the arc of a circle of diameter 12 meters subtended by the central angle is 63°.
For the following exercises, use the given information to find the area of the sector. Round to four decimal places.
46. A sector of a circle has a central angle of 45° and a radius 6 cm.
47. A sector of a circle has a central angle of 30° and a radius of 20 cm.
48. A sector of a circle with diameter 10 feet and an angle of [latex]\frac{\pi }{2}[/latex] radians.
49. A sector of a circle with radius of 0.7 inches and an angle of [latex]\pi[/latex] radians.
For the following exercises, find the angle between 0° and 360° that is coterminal to the given angle.
50. −40°
51. −110°
52. 700°
53. 1400°
For the following exercises, find the angle between 0 and [latex]2\pi[/latex] in radians that is coterminal to the given angle.
54. [latex]-\frac{\pi }{9}[/latex]
55. [latex]\frac{10\pi }{3}[/latex]
56. [latex]\frac{13\pi }{6}[/latex]
57. [latex]\frac{44\pi }{9}[/latex]
58. A truck with 32-inch diameter wheels is traveling at 60 mi/h. Find the angular speed of the wheels in rad/min. How many revolutions per minute do the wheels make?
59. A bicycle with 24-inch diameter wheels is traveling at 15 mi/h. Find the angular speed of the wheels in rad/min. How many revolutions per minute do the wheels make?
60. A wheel of radius 8 inches is rotating 15°/s. What is the linear speed [latex]v[/latex], the angular speed in RPM, and the angular speed in rad/s?
61. A wheel of radius 14 inches is rotating 0.5 rad/s. What is the linear speed [latex]v[/latex], the angular speed in RPM, and the angular speed in deg/s?
62. A CD has diameter of 120 millimeters. When playing audio, the angular speed varies to keep the linear speed constant where the disc is being read. When reading along the outer edge of the disc, the angular speed is about 200 RPM (revolutions per minute). Find the linear speed.
63. When being burned in a writable CD-R drive, the angular speed of a CD is often much faster than when playing audio, but the angular speed still varies to keep the linear speed constant where the disc is being written. When writing along the outer edge of the disc, the angular speed of one drive is about 4800 RPM (revolutions per minute). Find the linear speed if the CD has diameter of 120 millimeters.
64. A person is standing on the equator of Earth (radius 3960 miles). What are his linear and angular speeds?
65. Find the distance along an arc on the surface of Earth that subtends a central angle of 5 minutes [latex]\left(1\text{ minute}=\frac{1}{60}\text{ degree}\right)[/latex] . The radius of Earth is 3960 miles.
66. Find the distance along an arc on the surface of Earth that subtends a central angle of 7 minutes [latex]\left(1\text{ minute}=\frac{1}{60}\text{ degree}\right)[/latex]. The radius of Earth is [latex]3960[/latex] miles.
67. Consider a clock with an hour hand and minute hand. What is the measure of the angle the minute hand traces in [latex]20[/latex] minutes?
68. Two cities have the same longitude. The latitude of city A is 9.00 degrees north and the latitude of city B is 30.00 degree north. Assume the radius of the earth is 3960 miles. Find the distance between the two cities.
69. A city is located at 40 degrees north latitude. Assume the radius of the earth is 3960 miles and the earth rotates once every 24 hours. Find the linear speed of a person who resides in this city.
70. A city is located at 75 degrees north latitude. Assume the radius of the earth is 3960 miles and the earth rotates once every 24 hours. Find the linear speed of a person who resides in this city.
71. Find the linear speed of the moon if the average distance between the earth and moon is 239,000 miles, assuming the orbit of the moon is circular and requires about 28 days. Express answer in miles per hour.
72. A bicycle has wheels 28 inches in diameter. A tachometer determines that the wheels are rotating at 180 RPM (revolutions per minute). Find the speed the bicycle is travelling down the road.
73. A car travels 3 miles. Its tires make 2640 revolutions. What is the radius of a tire in inches?
74. A wheel on a tractor has a 24-inch diameter. How many revolutions does the wheel make if the tractor travels 4 miles?
Candela Citations
- Precalculus. Authored by: OpenStax College. Provided by: OpenStax. Located at: http://cnx.org/contents/fd53eae1-fa23-47c7-bb1b-972349835c3c@5.175:1/Preface. License: CC BY: Attribution