Visualizing Quantitative (Numerical) Data

Quantitative, or numerical, data can also be summarized into frequency tables.

Example

A teacher records scores on a 20-point quiz for the 30 students in his class. The scores are:

19 20 18 18 17 18 19 17 20 18 20 16 20 15 17 12 18 19 18 19 17 20 18 16 15 18 20 5 0 0

These scores could be summarized into a frequency table by grouping like values:

Score	Frequency
0	2
5	1
12	1
15	2
16	2
17	4
18	8
19	4
20	6

Using the table from the first example, it would be possible to create a standard bar chart from this summary, like we did for categorical data:

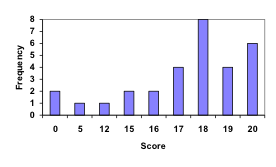
Bar graph. Vertical measures Frequency, in increments of 1 from 0-8. Horizontal measures Score, in irregular increments from 0-20. 18 is the highest frequency score, at 8, while 5 and 12 are the lowest frequency scores, at 1 each.
However, since the scores are numerical values, this chart doesn’t really make sense; the first and second bars are five values apart, while the later bars are only one value apart. It would be more correct to treat the horizontal axis as a number line. This type of graph is called a histogram.

Histogram

A histogram is like a bar graph, but where the horizontal axis is a number line.

example

For the values above, a histogram would look like:

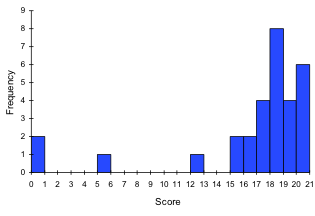
Bar graph. Vertical measures Frequency, in increments of 1 from 0-9. Horizontal measures Score, in increments of 1 from 0-21. This includes several empty scores, denoting 0 frequency, while keeping data of earlier bar graph on this page.

Notice that in the histogram, a bar represents values on the horizontal axis from that on the left hand-side of the bar up to, but not including, the value on the right hand side of the bar. Some people choose to have bars start at ½ values to avoid this ambiguity.

This video demonstrates the creation of the histogram from this data.

Unfortunately, not a lot of common software packages can correctly graph a histogram. About the best you can do in Excel or Word is a bar graph with no gap between the bars and spacing added to simulate a numerical horizontal axis.

If we have a large number of widely varying data values, creating a frequency table that lists every possible value as a category would lead to an exceptionally long frequency table, and probably would not reveal any patterns. For this reason, it is common with quantitative data to group data into class intervals.

Class Intervals

Class intervals are groupings of the data. In general, we define class intervals so that

each interval is equal in size. For example, if the first class contains values from 120-129, the second class should include values from 130-139.
we have somewhere between 5 and 20 classes, typically, depending upon the number of data we’re working with.

example

Suppose that we have collected weights from 100 male subjects as part of a nutrition study. For our weight data, we have values ranging from a low of 121 pounds to a high of 263 pounds, giving a total span of 263-121 = 142. We could create 7 intervals with a width of around 20, 14 intervals with a width of around 10, or somewhere in between. Oftentimes we have to experiment with a few possibilities to find something that represents the data well. Let us try using an interval width of 15. We could start at 121, or at 120 since it is a nice round number.

Interval	Frequency
120 – 134	4
135 – 149	14
150 – 164	16
165 – 179	28
180 – 194	12
195 – 209	8
210 – 224	7
225 – 239	6
240 – 254	2
255 – 269	3

A histogram of this data would look like:

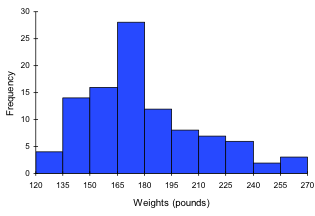
Bar graph. Vertical measures Frequency, in increments of 5 from 0-30. Horizontal measures Weights (pounds), in increments of 15 from 120-270. Measurements are noted between the 15-pound ranges, so that everyone weighing between 165-180, for instance, falls in the same category of measurement.