Finding the Volume and Surface Area of a Cylinder

Learning Outcomes

Find the volume and surface area of a cylinder

If you have ever seen a can of soda, you know what a cylinder looks like. A cylinder is a solid figure with two parallel circles of the same size at the top and bottom. The top and bottom of a cylinder are called the bases. The height [latex]h[/latex] of a cylinder is the distance between the two bases. For all the cylinders we will work with here, the sides and the height, [latex]h[/latex] , will be perpendicular to the bases.

A cylinder has two circular bases of equal size. The height is the distance between the bases.

An image of a cylinder is shown. There is a red arrow pointing to the radius of the top labeling it r, radius. There is a red arrow pointing to the height of the cylinder labeling it h, height.
Rectangular solids and cylinders are somewhat similar because they both have two bases and a height. The formula for the volume of a rectangular solid, [latex]V=Bh[/latex] , can also be used to find the volume of a cylinder.

For the rectangular solid, the area of the base, [latex]B[/latex] , is the area of the rectangular base, length × width. For a cylinder, the area of the base, [latex]B[/latex], is the area of its circular base, [latex]\pi {r}^{2}[/latex]. The image below compares how the formula [latex]V=Bh[/latex] is used for rectangular solids and cylinders.

Seeing how a cylinder is similar to a rectangular solid may make it easier to understand the formula for the volume of a cylinder.

To understand the formula for the surface area of a cylinder, think of a can of vegetables. It has three surfaces: the top, the bottom, and the piece that forms the sides of the can. If you carefully cut the label off the side of the can and unroll it, you will see that it is a rectangle. See the image below.

By cutting and unrolling the label of a can of vegetables, we can see that the surface of a cylinder is a rectangle. The length of the rectangle is the circumference of the cylinder’s base, and the width is the height of the cylinder.

A cylindrical can of green beans is shown. The height is labeled h. Beside this are pictures of circles for the top and bottom of the can and a rectangle for the other portion of the can. Above the circles is C equals 2 times pi times r. The top of the rectangle says l equals 2 times pi times r. The left side of the rectangle is labeled h, the right side is labeled w.
The distance around the edge of the can is the circumference of the cylinder’s base it is also the length [latex]L[/latex] of the rectangular label. The height of the cylinder is the width [latex]W[/latex] of the rectangular label. So the area of the label can be represented as

The top line says A equals l times red w. Below the l is 2 times pi times r. Below the w is a red h.
To find the total surface area of the cylinder, we add the areas of the two circles to the area of the rectangle.

A rectangle is shown with circles coming off the top and bottom.
The surface area of a cylinder with radius [latex]r[/latex] and height [latex]h[/latex], is

[latex]S=2\pi {r}^{2}+2\pi rh[/latex]

Volume and Surface Area of a Cylinder

For a cylinder with radius [latex]r[/latex] and height [latex]h:[/latex]

A cylinder is shown. The height is labeled h and the radius of the top is labeled r. Beside it is Volume: V equals pi times r squared times h or V equals capital B times h. Below this is Surface Area: S equals 2 times pi times r squared plus 2 times pi times r times h.

example

A cylinder has height [latex]5[/latex] centimeters and radius [latex]3[/latex] centimeters. Find the 1. volume and 2. surface area.

Solution

Step 1. Read the problem. Draw the figure and label

it with the given information.

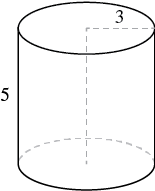1.
Step 2. Identify what you are looking for.	the volume of the cylinder
Step 3. Name. Choose a variable to represent it.	let V = volume
Step 4. Translate. Write the appropriate formula. Substitute. (Use [latex]3.14[/latex] for [latex]\pi[/latex] )	[latex]V=\pi {r}^{2}h[/latex] [latex]V\approx \left(3.14\right){3}^{2}\cdot 5[/latex]
Step 5. Solve.	[latex]V\approx 141.3[/latex]
Step 6. Check: We leave it to you to check your calculations.
Step 7. Answer the question.	The volume is approximately [latex]141.3[/latex] cubic inches.

2.
Step 2. Identify what you are looking for.	the surface area of the cylinder
Step 3. Name. Choose a variable to represent it.	let S = surface area
Step 4. Translate. Write the appropriate formula. Substitute. (Use [latex]3.14[/latex] for [latex]\pi[/latex] )	[latex]S=2\pi {r}^{2}+2\pi rh[/latex] [latex]S\approx 2\left(3.14\right){3}^{2}+2\left(3.14\right)\left(3\right)5[/latex]
Step 5. Solve.	[latex]S\approx 150.72[/latex]
Step 6. Check: We leave it to you to check your calculations.
Step 7. Answer the question.	The surface area is approximately [latex]150.72[/latex] square inches.

try it

example

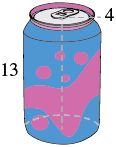
Find the 1. volume and 2. surface area of a can of soda. The radius of the base is [latex]4[/latex] centimeters and the height is [latex]13[/latex] centimeters. Assume the can is shaped exactly like a cylinder.

Show Solution