Using the Properties of Triangles to Solve Problems

Learning Outcomes

  • Find the area, base and height of a triangle
  • Find the length of one side of a triangle given the perimeter and two other lengths

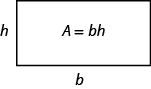
We now know how to find the area of a rectangle. We can use this fact to help us visualize the formula for the area of a triangle. In the rectangle below, we’ve labeled the length [latex]b[/latex] and the width [latex]h[/latex], so it’s area is [latex]bh[/latex].

The area of a rectangle is the base, [latex]b[/latex], times the height, [latex]h[/latex].

A rectangle is shown. The side is labeled h and the bottom is labeled b. The center says A equals bh.
We can divide this rectangle into two congruent triangles (see the image below). Triangles that are congruent have identical side lengths and angles, and so their areas are equal. The area of each triangle is one-half the area of the rectangle, or [latex]\Large\frac{1}{2}\normalsize bh[/latex]. This example helps us see why the formula for the area of a triangle is [latex]A=\Large\frac{1}{2}\normalsize bh[/latex].

A rectangle can be divided into two triangles of equal area. The area of each triangle is one-half the area of the rectangle.

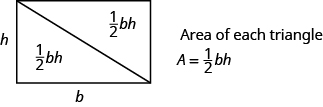
A rectangle is shown. A diagonal line is drawn from the upper left corner to the bottom right corner. The side of the rectangle is labeled h and the bottom is labeled b. Each triangle says one-half bh. To the right of the rectangle, it says
The formula for the area of a triangle is [latex]A=\Large\frac{1}{2}\normalsize bh[/latex], where [latex]b[/latex] is the base and [latex]h[/latex] is the height.

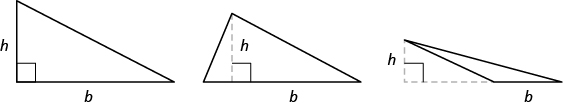
To find the area of the triangle, you need to know its base and height. The base is the length of one side of the triangle, usually the side at the bottom. The height is the length of the line that connects the base to the opposite vertex, and makes a [latex]\text{90}^ \circ[/latex] angle with the base. The image below shows three triangles with the base and height of each marked.

The height [latex]h[/latex] of a triangle is the length of a line segment that connects the the base to the opposite vertex and makes a [latex]\text{90}^ \circ[/latex] angle with the base.

Three triangles are shown. The triangle on the left is a right triangle. The bottom is labeled b and the side is labeled h. The middle triangle is an acute triangle. The bottom is labeled b. There is a dotted line from the top vertex to the base of the triangle, forming a right angle with the base. That line is labeled h. The triangle on the right is an obtuse triangle. The bottom of the triangle is labeled b. The base has a dotted line extended out and forms a right angle with a dotted line to the top of the triangle. The vertical line is labeled h.

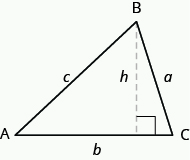
Triangle Properties

For any triangle [latex]\Delta ABC[/latex], the sum of the measures of the angles is [latex]\text{180}^ \circ[/latex].

[latex]m\angle{A}+m\angle{B}+m\angle{C}=180^\circ[/latex]

The perimeter of a triangle is the sum of the lengths of the sides.

[latex]P=a+b+c[/latex]

The area of a triangle is one-half the base, [latex]b[/latex], times the height, [latex]h[/latex].

[latex]A={\Large\frac{1}{2}}bh[/latex]

A triangle is shown. The vertices are labeled A, B, and C. The sides are labeled a, b, and c. There is a vertical dotted line from vertex B at the top of the triangle to the base of the triangle, meeting the base at a right angle. The dotted line is labeled h.

 

example

Find the area of a triangle whose base is [latex]11[/latex] inches and whose height is [latex]8[/latex] inches.

Solution

Step 1. Read the problem. Draw the figure and label it with the given information. .
Step 2. Identify what you are looking for. the area of the triangle
Step 3. Name. Choose a variable to represent it. let A = area of the triangle
Step 4.Translate.

Write the appropriate formula.

Substitute.

 .
Step 5. Solve the equation. [latex]A=44[/latex] square inches.
Step 6. Check:

[latex]A={ \Large\frac{1}{2}}bh[/latex]

[latex]44\stackrel{?}{=}{ \Large\frac{1}{2}}(11)8[/latex]

[latex]44=44\quad\checkmark[/latex]
Step 7. Answer the question. The area is [latex]44[/latex] square inches.

 

try it

The following video provides another example of how to use the area formula for triangles.

example

The perimeter of a triangular garden is [latex]24[/latex] feet. The lengths of two sides are [latex]4[/latex] feet and [latex]9[/latex] feet. How long is the third side?

 

try it

 

example

The area of a triangular church window is [latex]90[/latex] square meters. The base of the window is [latex]15[/latex] meters. What is the window’s height?

 

try it

In our next video, we show another example of how to find the height of a triangle given it’s area.

Isosceles and Equilateral Triangles

Besides the right triangle, some other triangles have special names. A triangle with two sides of equal length is called an isosceles triangle. A triangle that has three sides of equal length is called an equilateral triangle. The image below shows both types of triangles.

In an isosceles triangle, two sides have the same length, and the third side is the base. In an equilateral triangle, all three sides have the same length.

Two triangles are shown. All three sides of the triangle on the left are labeled s. It is labeled

Isosceles and Equilateral Triangles

An isosceles triangle has two sides the same length.
An equilateral triangle has three sides of equal length.

 

example

The perimeter of an equilateral triangle is [latex]93[/latex] inches. Find the length of each side.

 

try it

 

example

Arianna has [latex]156[/latex] inches of beading to use as trim around a scarf. The scarf will be an isosceles triangle with a base of [latex]60[/latex] inches. How long can she make the two equal sides?

 

try it