Section 1.1 Solutions

1. Yes, if the point is on the [latex]x[/latex]– or [latex]y[/latex]-axis.

3. A [latex]y[/latex]-intercept is where the graph crosses the [latex]y[/latex]-axis [latex](x=0)[/latex].

5. [latex]x[/latex]–[latex]int=(2,0)[/latex]; [latex]y[/latex]–[latex]int=(0,6)[/latex]

7. [latex]x[/latex]–[latex]int=(2,0)[/latex]; [latex]y[/latex]–[latex]int=(0,-3)[/latex]

9. [latex]x[/latex]–[latex]int=(3,0)[/latex]; [latex]y[/latex]–[latex]int=\left(0,\frac{9}{8}\right)[/latex]

11. [latex]y=4-2x[/latex]

13. [latex]y=\frac{5}{3}-\frac{2}{3}x[/latex]

15. [latex]y=2x-\frac{4}{5}[/latex]

17. [latex]\sqrt{74}[/latex]

19. [latex]6[/latex]

21. [latex]d\approx 62.97[/latex]

23. [latex]\left(3,-\frac{3}{2}\right)[/latex]

25. [latex](2,-1)[/latex]

27. [latex](0,0)[/latex]

29. [latex]0[/latex]

31. Not collinear

 

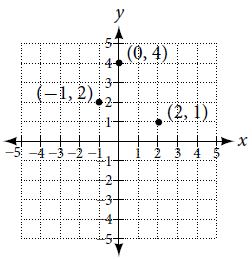
33 [latex]A=(-3,2),\text{ }B=(1,3),\text{ }C=(4,0)[/latex]

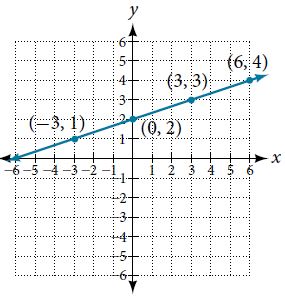
35.

x	-3	0	3	6
y	1	2	3	4

 

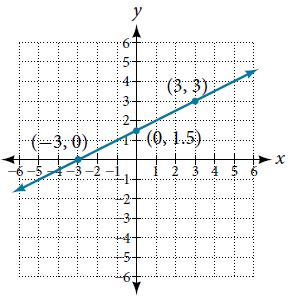
37.

x	-3	0	3
y	0	1.5	3

 

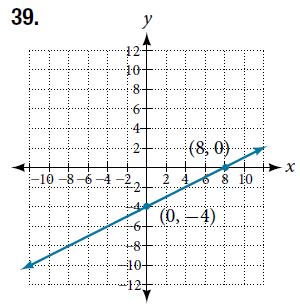
39. [latex]x[/latex]–[latex]int=(8,0)[/latex]; [latex]y[/latex]–[latex]int=(0,-4)[/latex]

 

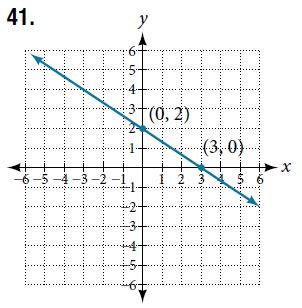
41. [latex]x[/latex]–[latex]int=(3,0)[/latex]; [latex]y[/latex]–[latex]int=(0,2)[/latex]

 

43. [latex]d=8.246[/latex]

45. [latex]d=5[/latex]

47. [latex](-3,4)[/latex]

49. [latex]\text{ }15-11.2=3.8[/latex] mi shorter

51. [latex]\text{ }6.042[/latex]

53. [latex]\text{ }37\text{ }mi[/latex]

Section 1.2 Solutions

1. [latex]x^{2}+y^{2}=49[/latex]

3. [latex]x^{2}+y^{2}=2[/latex]

5. [latex]{\left(x-3\right)}^{2}+{\left(y-5\right)}^{2}=1[/latex]

7. [latex]{\left(x-1.5\right)}^{2}+{\left(y+3.5\right)}^{2}=6.25[/latex]

9. [latex]{\left(x-3\right)}^{2}+{\left(y+2\right)}^{2}=64[/latex]

11. [latex]{\left(x-4\right)}^{2}+{\left(y-4\right)}^{2}=8[/latex]

13. Center: [latex](-5,-3)[/latex], Radius: [latex]1[/latex]

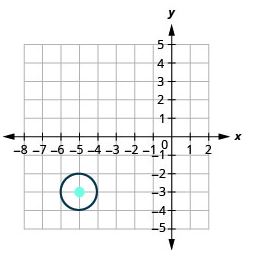
15. Center: [latex](4,-2)[/latex], Radius: [latex]4[/latex]

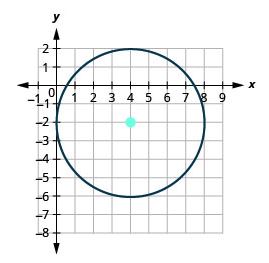
17. Center: [latex](0,-2)[/latex], Radius: [latex]5[/latex]

19. Center: [latex](1.5,2.5)[/latex], Radius: [latex]0.5[/latex]

21. Center: [latex](0,0)[/latex], Radius: [latex]8[/latex]

23. Center: [latex](0,0)[/latex], Radius: [latex]2[/latex]

25. Center: [latex](-1,-3)[/latex], Radius: [latex]1[/latex]

27. Center: [latex](2,-5)[/latex], Radius: [latex]6[/latex]

29. Center: [latex](0,-3)[/latex], Radius: [latex]2[/latex]

31. Center: [latex](-2,0)[/latex], Radius: [latex]2[/latex]

33. Answers will vary.

35. Answers will vary.

Section 1.3 Solutions

1. A relation is a set of ordered pairs. A function is a special kind of relation in which no two ordered pairs have the same first coordinate.

3. When a vertical line intersects the graph of a relation more than once, that indicates that for that input there is more than one output. At any particular input value, there can be only one output if the relation is to be a function.

5. function

7. function

9. function

11. function

13. function

15. function

17. function

19. function

21. function

23. not a function

25. [latex]f\left(-3\right)=-11\\ f\left(2\right)=-1\\ f\left(-a\right)=-2a - 5\\-f\left(a\right)=-2a+5\\f\left(a+h\right)=2a+2h - 5[/latex]

27. [latex]f\left(-3\right)=\sqrt{5}+5\\ f\left(2\right)=5\\ f\left(-a\right)=\sqrt{2+a}+5\\ -f\left(a\right)=-\sqrt{2-a}-5\\f\left(a+h\right)=\sqrt{2-a-h}+5[/latex]

29. [latex]f\left(-3\right)=2\\f\left(2\right)=1 - 3=-2\\f\left(-a\right)=|-a - 1|-|-a+1|\\ -f\left(a\right)=-|a - 1|+|a+1|\\\text{ }\text{ }f\left(a+h\right)=|a+h - 1|-|a+h+1|[/latex]

31. [latex]\frac{g\left(x\right)-g\left(a\right)}{x-a}=x+a+2,x\ne a[/latex]

33. a. [latex]f\left(-2\right)=14[/latex]; b. [latex]x=3[/latex]

35. a. [latex]f\left(5\right)=10[/latex]; b. [latex]x=-1\text{ }[/latex] or [latex]\text{ }x=4[/latex]

37. a. [latex]f\left(t\right)=6-\frac{2}{3}t[/latex]; b. [latex]f\left(-3\right)=8[/latex]; c. [latex]t=6[/latex]

39. not a function

41. function

43. function

45. function

49. function

51. a. [latex]f\left(0\right)=1[/latex]; b. [latex]f\left(x\right)=-3,x=-2\text{ }[/latex] or [latex]\text{ }x=2[/latex]

53. function

55. not a function

57. function

59. [latex]f\left(3\right)=53[/latex]

61. [latex]f\left(-2\right)=8\\ f\left(-1\right)=6\\ f\left(0\right)=4\\f\left(1\right)=2\\ f\left(2\right)=0[/latex]

63. [latex]f\left(-2\right)=49\\ f\left(-1\right)=18\\ f\left(0\right)=3\\ f\left(1\right)=4\\ f\left(2\right)=21[/latex]

65. [latex]f\left(-2\right)=-4\\ f\left(-1\right)=-\frac{3}{2}\\ f\left(0\right)=-\frac{2}{3}\\ f\left(1\right)=-\frac{1}{4}\\f\left(2\right)=0[/latex]

67. [latex]3f(1)−4g(−2)=-1[/latex]

69. [latex]13=f\left(40\right)[/latex].
[latex]f\left(5\right)=2[/latex] means that a population of 5000 will produce 2 tons of garbage each week.

71. [latex]f\left(5\right)=30[/latex] means there are 30 ducks in the lake in 1995.
[latex]f\left(10\right)=40[/latex] means there are 40 ducks in the lake in 2000.

Section 1.4 Solutions

1. Terry starts at an elevation of 3000 feet and descends 70 feet per second.

3. 3 miles per hour

5. [latex]d\left(t\right)=100 - 10t[/latex]

7. Yes.

9. No.

11. No.

13. No.

15. Increasing.

17. Decreasing.

19. Decreasing.

21. Increasing.

23. Decreasing.

25. 3

27. [latex]-\frac{1}{3}[/latex]

29. [latex]\frac{4}{5}[/latex]

31. [latex]f\left(x\right)=-\frac{1}{2}x+\frac{7}{2}[/latex]

33. [latex]y=2x+3[/latex]

35. [latex]y=-\frac{1}{3}x+\frac{22}{3}[/latex]

37. [latex]y=\frac{4}{5}x+4[/latex]

39. [latex]-\frac{5}{4}[/latex]

41. [latex]y=\frac{2}{3}x+1[/latex]

43. [latex]y=-2x+3[/latex]

45. [latex]y=3[/latex]

47. Linear, [latex]g\left(x\right)=-3x+5[/latex]

49. Linear, [latex]f\left(x\right)=5x - 5[/latex]

51. Linear, [latex]g\left(x\right)=-\frac{25}{2}x+6[/latex]

53. Linear, [latex]f\left(x\right)=10x - 24[/latex]

55. [latex]x=-\frac{16}{3}[/latex]

57. [latex]x=a[/latex]

59. [latex]y=\frac{d}{c-a}x-\frac{ad}{c-a}[/latex]

61. F

63. C

65. A

67.

69.

71.

73.

75.

77.

79.

81.

83.

85.

87. [latex]g\left(x\right)=0.75x - 5.5\text{}[/latex] 0.75 [latex]\left(0,-5.5\right)[/latex]

89. [latex]y=3[/latex]

91. [latex]x=-3[/latex]

Section 1.5 Solutions

1. The slopes are equal; y-intercepts are not equal.

3. Determine the independent variable. This is the variable upon which the output depends.

5. To determine the initial value, find the output when the input is equal to zero.

7. First, find the slope of the linear function. Then take the negative reciprocal of the slope; this is the slope of the perpendicular line. Substitute the slope of the perpendicular line and the coordinate of the given point into the equation [latex]y=mx+b[/latex] and solve for b. Then write the equation of the line in the form [latex]y=mx+b[/latex] by substituting in m and b.

9. neither parallel or perpendicular

11. perpendicular

13. parallel

15. [latex]\text{Line 1}: m=8 \text{ Line 2}: m=-6 \text{Neither}[/latex]

17. [latex]\text{Line 1}: m=-\frac{1}{2} \text{ Line 2}: m=2 \text{Perpendicular}[/latex]

19. [latex]g\left(x\right)=3x - 3[/latex]

21. [latex]p\left(t\right)=-\frac{1}{3}t+2[/latex]

23. $45 per training session.

25. The rate of change is 0.1. For every additional minute talked, the monthly charge increases by $0.1 or 10 cents. The initial value is 24. When there are no minutes talked, initially the charge is $24.

27. The slope is –400. This means for every year between 1960 and 1989, the population dropped by 400 per year in the city.

29. c

31. 2,300

33. 64,170

35. [latex]P\left(t\right)=75,000+2500t[/latex]

37. (–30, 0) Thirty years before the start of this model, the town had no citizens. (0, 75,000) Initially, the town had a population of 75,000.

39. Ten years after the model began.

41. [latex]W\left(t\right)=\text{7}.\text{5}t+0.\text{5}[/latex]

43. (–15, 0): The x-intercept is not a plausible set of data for this model because it means the baby weighed 0 pounds 15 months prior to birth. (0, 7.5): The baby weighed 7.5 pounds at birth.

45. At age 5.8 months.

47. [latex]C\left(t\right)=12,025 - 205t[/latex]

49. (58.7, 0): In roughly 59 years, the number of people inflicted with the common cold would be 0. (0, 12,025): Initially there were 12,025 people afflicted by the common cold.

51. 2064

53. [latex]y=-2t+180[/latex]

55. In 2070, the company’s profit will be zero.

57. [latex]y=30t - 300[/latex]

59. (10, 0) In 1990, the profit earned zero profit.

61. Hawaii

63. During the year 1933

65. $105,620

67.

a. 696 people
b. 4 years
c. 174 people per year
d. 305 people
e. [latex]P\left(t\right)=305+174t[/latex]
f. 2219 people

69.

a. [latex]C\left(x\right)=0.15x+10[/latex]
b. The flat monthly fee is $10 and there is an additional $0.15 fee for each additional minute used
c. $113.05

71.

a. [latex]P\left(t\right)=190t+4360[/latex]
b. 6640 moose

73.

a. [latex]R\left(t\right)=16 - 2.1t[/latex]
b. 5.5 billion cubic feet
c. During the year 2017

75. More than 133 minutes

77. More than $42,857.14 worth of jewelry

79. $66,666.67

Section 1.6 Solutions

1. The domain of a function depends upon what values of the independent variable make the function undefined or imaginary.

3.  There is no restriction on [latex]x[/latex] for [latex]f\left(x\right)=\sqrt[3]{x}[/latex] because you can take the cube root of any real number. So the domain is all real numbers, [latex]\left(-\infty ,\infty \right)[/latex]. When dealing with the set of real numbers, you cannot take the square root of negative numbers. So [latex]x[/latex] -values are restricted for [latex]f\left(x\right)=\sqrt[]{x}[/latex] to nonnegative numbers and the domain is [latex]\left[0,\infty \right)[/latex].

5. Graph each formula of the piecewise function over its corresponding domain. Use the same scale for the [latex]x[/latex] -axis and [latex]y[/latex] -axis for each graph. Indicate inclusive endpoints with a solid circle and exclusive endpoints with an open circle. Use an arrow to indicate [latex]-\infty [/latex] or [latex]\text{ }\infty [/latex]. Combine the graphs to find the graph of the piecewise function.

7. [latex]\left(-\infty ,\infty \right)[/latex]

9. [latex]\left(-\infty ,3\right][/latex]

11. [latex]\left(-\infty ,\infty \right)[/latex]

13. [latex]\left(-\infty ,\infty \right)[/latex]

15. [latex]\left(-\infty ,-\frac{1}{2}\right)\cup \left(-\frac{1}{2},\infty \right)[/latex]

17. [latex]\left(-\infty ,-11\right)\cup \left(-11,2\right)\cup \left(2,\infty \right)[/latex]

19. [latex]\left(-\infty ,-3\right)\cup \left(-3,5\right)\cup \left(5,\infty \right)[/latex]

21. [latex]\left(-\infty ,5\right)[/latex]

23. [latex]\left[6,\infty \right)[/latex]

25. [latex]\left(-\infty ,-9\right)\cup \left(-9,9\right)\cup \left(9,\infty \right)[/latex]

27. Domain: [latex]\left(2,8\right][/latex]   Range [latex]\left[6,8\right)[/latex]

29. Domain: [latex]\left[-4, 4\right][/latex]   Range: [latex]\left[0, 2\right][/latex]

31. Domain: [latex]\left[-5,\text{ }3\right)[/latex]   Range: [latex]\left[0,2\right][/latex]

33. Domain: [latex]\left(-\infty ,1\right][/latex]   Range: [latex]\left[0,\infty \right)[/latex]

35. Domain: [latex]\left[-6,-\frac{1}{6}\right]\cup \left[\frac{1}{6},6\right][/latex]   Range: [latex]\left[-6,-\frac{1}{6}\right]\cup \left[\frac{1}{6},6\right][/latex]

37. Domain: [latex]\left[-3,\text{ }\infty \right)[/latex]   Range: [latex]\left[0,\infty \right)[/latex]

39. Domain: [latex]\left(-\infty ,\infty \right)[/latex]

41. Domain: [latex]\left(-\infty ,\infty \right)[/latex]

43. Domain: [latex]\left(-\infty ,\infty \right)[/latex]

45. Domain: [latex]\left(-\infty ,\infty \right)[/latex]

47. [latex]\begin{cases}f\left(-3\right)=1;& f\left(-2\right)=0;& f\left(-1\right)=0;& f\left(0\right)=0\end{cases}[/latex]

49. [latex]\begin{cases}f\left(-1\right)=-4;& f\left(0\right)=6;& f\left(2\right)=20;& f\left(4\right)=34\end{cases}[/latex]

51. [latex]\begin{cases}f\left(-1\right)=-5;& f\left(0\right)=3;& f\left(2\right)=3;& f\left(4\right)=16\end{cases}[/latex]

53. Domain: [latex]\left(-\infty ,1\right)\cup \left(1,\infty \right)[/latex]

55. Window: [latex]\left[-0.5,-0.1\right][/latex]   Range: [latex]\left[4,\text{ }100\right][/latex]

Window: [latex]\left[0.1,\text{ }0.5\right][/latex]   Range: [latex]\left[4,\text{ }100\right][/latex]

57. [latex]\left[0,\text{ }8\right][/latex]

59. Many answers. One function is [latex]f\left(x\right)=\frac{1}{\sqrt{x - 2}}[/latex].

61. The domain is [latex]\left[0,\text{ }6\right][/latex]; it takes 6 seconds for the projectile to leave the ground and return to the ground.

Section 1.7 Solutions

1. Yes, the average rate of change of all linear functions is constant.

3. The absolute maximum and minimum relate to the entire graph, whereas the local extrema relate only to a specific region around an open interval.

5. The difference quotient is the slope of the secant line between two points. The difference quotient can be used to find the slope of a curve, as well as the slope of a straight line.

7. [latex]2\left(b+4\right)[/latex]

9. [latex]6x+3h[/latex]

11. 4

13. [latex]4x+2h-5[/latex]

15. [latex]\frac{-1}{(x+3)(x+h+3)}[/latex]

17. [latex]\frac{4}{3}[/latex]

19. y-axis symmetry

21. x-axis symmetry

23. x-axis, y-axis, and origin symmetry

25. no symmetry

27. even

29. odd

31. odd

33. even

35. odd

37. increasing on [latex]\left(-\infty ,-2.5\right)\cup \left(1,\infty \right)[/latex], decreasing on [latex]\left(-2.5,\text{ }1\right)[/latex]

39. increasing on [latex]\left(-\infty ,1\right)\cup \left(3,4\right)[/latex], decreasing on [latex]\left(1,3\right)\cup \left(4,\infty \right)[/latex]

41. local maximum: [latex]\left(-3,\text{ }60\right)[/latex], local minimum: [latex]\left(3,\text{ }-60\right)[/latex]

43. absolute maximum at approximately [latex]\left(7,\text{ }150\right)[/latex], absolute minimum at approximately [latex]\left(-7.5,\text{ }-220\right)[/latex]

45. a. –3000; b. –1250

47. –4

49. 27

51. –0.167

53. Local minimum at [latex]\left(3,-22\right)[/latex], decreasing on [latex]\left(-\infty ,\text{ }3\right)[/latex], increasing on [latex]\left(3,\text{ }\infty \right)[/latex]

55. Local minimum at [latex]\left(-2,-2\right)[/latex], decreasing on [latex]\left(-3,-2\right)[/latex], increasing on [latex]\left(-2,\text{ }\infty \right)[/latex]

57. Local maximum at [latex]\left(-0.5,\text{ }6\right)[/latex], local minima at [latex]\left(-3.25,-47\right)[/latex] and [latex]\left(2.1,-32\right)[/latex], decreasing on [latex]\left(-\infty ,-3.25\right)[/latex] and [latex]\left(-0.5,\text{ }2.1\right)[/latex], increasing on [latex]\left(-3.25,\text{ }-0.5\right)[/latex] and [latex]\left(2.1,\text{ }\infty \right)[/latex]

59. A) a relative (local) maximum of the function

61. [latex]b=5[/latex]

63. 2.7 gallons per minute

65. approximately –0.6 milligrams per day

Section 1.8 Solutions

1. Find the numbers that make the function in the denominator [latex]g[/latex] equal to zero, and check for any other domain restrictions on [latex]f[/latex] and [latex]g[/latex], such as an even-indexed root or zeros in the denominator.

3. Yes. Sample answer: Let [latex]f\left(x\right)=x+1\text{ and }g\left(x\right)=x - 1[/latex]. Then [latex]f\left(g\left(x\right)\right)=f\left(x - 1\right)=\left(x - 1\right)+1=x[/latex] and [latex]g\left(f\left(x\right)\right)=g\left(x+1\right)=\left(x+1\right)-1=x[/latex]. So [latex]f\circ g=g\circ f[/latex].

5. [latex]\left(f+g\right)\left(x\right)=2x+6[/latex], domain: [latex]\left(-\infty ,\infty \right)[/latex]

[latex]\left(f-g\right)\left(x\right)=2{x}^{2}+2x - 6[/latex], domain: [latex]\left(-\infty ,\infty \right)[/latex]

[latex]\left(fg\right)\left(x\right)=-{x}^{4}-2{x}^{3}+6{x}^{2}+12x[/latex], domain: [latex]\left(-\infty ,\infty \right)[/latex]

[latex]\left(\frac{f}{g}\right)\left(x\right)=\frac{{x}^{2}+2x}{6-{x}^{2}}[/latex], domain: [latex]\left(-\infty ,-\sqrt{6}\right)\cup \left(-\sqrt{6},\sqrt{6}\right)\cup \left(\sqrt{6},\infty \right)[/latex]

7. [latex]\left(f+g\right)\left(x\right)=\frac{4{x}^{3}+8{x}^{2}+1}{2x}[/latex], domain: [latex]\left(-\infty ,0\right)\cup \left(0,\infty \right)[/latex]

[latex]\left(f-g\right)\left(x\right)=\frac{4{x}^{3}+8{x}^{2}-1}{2x}[/latex], domain: [latex]\left(-\infty ,0\right)\cup \left(0,\infty \right)[/latex]

[latex]\left(fg\right)\left(x\right)=x+2[/latex], domain: [latex]\left(-\infty ,0\right)\cup \left(0,\infty \right)[/latex]

[latex]\left(\frac{f}{g}\right)\left(x\right)=4{x}^{3}+8{x}^{2}[/latex], domain: [latex]\left(-\infty ,0\right)\cup \left(0,\infty \right)[/latex]

9. [latex]\left(f+g\right)\left(x\right)=3{x}^{2}+\sqrt{x - 5}[/latex], domain: [latex]\left[5,\infty \right)[/latex]

[latex]\left(f-g\right)\left(x\right)=3{x}^{2}-\sqrt{x - 5}[/latex], domain: [latex]\left[5,\infty \right)[/latex]

[latex]\left(fg\right)\left(x\right)=3{x}^{2}\sqrt{x - 5}[/latex], domain: [latex]\left[5,\infty \right)[/latex]

[latex]\left(\frac{f}{g}\right)\left(x\right)=\frac{3{x}^{2}}{\sqrt{x - 5}}[/latex], domain: [latex]\left(5,\infty \right)[/latex]

11. a. 3; b. [latex]f\left(g\left(x\right)\right)=2{\left(3x - 5\right)}^{2}+1[/latex]; c. [latex]f\left(g\left(x\right)\right)=6{x}^{2}-2[/latex]; d. [latex]\left(g\circ g\right)\left(x\right)=3\left(3x - 5\right)-5=9x - 20[/latex]; e. [latex]\left(f\circ f\right)\left(-2\right)=163[/latex]

13. [latex]f\left(g\left(x\right)\right)=\sqrt{{x}^{2}+3}+2,g\left(f\left(x\right)\right)=x+4\sqrt{x}+7[/latex]

15. [latex]f\left(g\left(x\right)\right)=\sqrt[3]{\frac{x+1}{{x}^{3}}}=\frac{\sqrt[3]{x+1}}{x},g\left(f\left(x\right)\right)=\frac{\sqrt[3]{x}+1}{x}[/latex]

17. [latex]\left(f\circ g\right)\left(x\right)=\frac{1}{\frac{2}{x}+4 - 4}=\frac{x}{2},\text{ }\left(g\circ f\right)\left(x\right)=2x - 4[/latex]

19. [latex]f\left(g\left(h\left(x\right)\right)\right)={\left(\frac{1}{x+3}\right)}^{2}+1[/latex]

21. a. [latex]\left(g\circ f\right)\left(x\right)=-\frac{3}{\sqrt{2 - 4x}}[/latex]; b. [latex]\left(-\infty ,\frac{1}{2}\right)[/latex]

23. a. [latex]\left(0,2\right)\cup \left(2,\infty \right)[/latex]; b. [latex]\left(-\infty ,-2\right)\cup \left(2,\infty \right)[/latex]; c. [latex]\left(0,\infty \right)[/latex]

25. [latex]\left(1,\infty \right)[/latex]

27. sample: [latex]\begin{cases}f\left(x\right)={x}^{3}\\ g\left(x\right)=x - 5\end{cases}[/latex]

29. sample: [latex]\begin{cases}f\left(x\right)=\frac{4}{x}\hfill \\ g\left(x\right)={\left(x+2\right)}^{2}\hfill \end{cases}[/latex]

31. sample: [latex]\begin{cases}f\left(x\right)=\sqrt[3]{x}\\ g\left(x\right)=\frac{1}{2x - 3}\end{cases}[/latex]

33. sample: [latex]\begin{cases}f\left(x\right)=\sqrt[4]{x}\\ g\left(x\right)=\frac{3x - 2}{x+5}\end{cases}[/latex]

35. sample: [latex]f\left(x\right)=\sqrt{x}[/latex]
[latex]g\left(x\right)=2x+6[/latex]

37.sample: [latex]f\left(x\right)=\sqrt[3]{x}[/latex]
[latex]g\left(x\right)=\left(x - 1\right)[/latex]

39. sample: [latex]f\left(x\right)={x}^{3}[/latex]
[latex]g\left(x\right)=\frac{1}{x - 2}[/latex]

41. sample: [latex]f\left(x\right)=\sqrt{x}[/latex]
[latex]g\left(x\right)=\frac{2x - 1}{3x+4}[/latex]

43. 2

45. 5

47. 4

49. 0

51. 2

53. 1

55. 4

57. 4

59. 9

61. 4

63. 2

65. 3

67. 11

69. 0

71. 7

73. [latex]f\left(g\left(0\right)\right)=27,g\left(f\left(0\right)\right)=-94[/latex]

75. [latex]f\left(g\left(0\right)\right)=\frac{1}{5},g\left(f\left(0\right)\right)=5[/latex]

77. [latex]18{x}^{2}+60x+51[/latex]

79. [latex]g\circ g\left(x\right)=9x+20[/latex]

81. 2

83. [latex]\left(-\infty ,\infty \right)[/latex]

85. False

87. [latex]\left(f\circ g\right)\left(6\right)=6[/latex] ; [latex]\left(g\circ f\right)\left(6\right)=6[/latex]

89. [latex]\left(f\circ g\right)\left(11\right)=11,\left(g\circ f\right)\left(11\right)=11[/latex]

91. c. Solve [latex]A\left(m\left(t\right)\right)=4[/latex].

93. [latex]A\left(t\right)=\pi {\left(25\sqrt{t+2}\right)}^{2}[/latex] and [latex]A\left(2\right)=\pi {\left(25\sqrt{4}\right)}^{2}=2500\pi [/latex] square inches

95. [latex]A\left(5\right)=\pi {\left(2\left(5\right)+1\right)}^{2}=121\pi [/latex] square units

97. a. [latex]N\left(T\left(t\right)\right)=23{\left(5t+1.5\right)}^{2}-56\left(5t+1.5\right)+1[/latex];
b. 3.38 hours

Section 1.9 Solutions

1. A horizontal shift results when a constant is added to or subtracted from the input. A vertical shifts results when a constant is added to or subtracted from the output.

3. A horizontal compression results when a constant greater than 1 is multiplied by the input. A vertical compression results when a constant between 0 and 1 is multiplied by the output.

5. For a function [latex]f[/latex], substitute [latex]\left(-x\right)[/latex] for [latex]\left(x\right)[/latex] in [latex]f\left(x\right)[/latex]. Simplify. If the resulting function is the same as the original function, [latex]f\left(-x\right)=f\left(x\right)[/latex], then the function is even. If the resulting function is the opposite of the original function, [latex]f\left(-x\right)=-f\left(x\right)[/latex], then the original function is odd. If the function is not the same or the opposite, then the function is neither odd nor even.

7. [latex]g\left(x\right)=|x - 1|-3[/latex]

9. [latex]g\left(x\right)=\frac{1}{{\left(x+4\right)}^{2}}+2[/latex]

11. The graph of [latex]f\left(x+43\right)[/latex] is a horizontal shift to the left 43 units of the graph of [latex]f[/latex].

13. The graph of [latex]f\left(x - 4\right)[/latex] is a horizontal shift to the right 4 units of the graph of [latex]f[/latex].

15. The graph of [latex]f\left(x\right)+8[/latex] is a vertical shift up 8 units of the graph of [latex]f[/latex].

17. The graph of [latex]f\left(x\right)-7[/latex] is a vertical shift down 7 units of the graph of [latex]f[/latex].

19. The graph of [latex]f\left(x+4\right)-1[/latex] is a horizontal shift to the left 4 units and a vertical shift down 1 unit of the graph of [latex]f[/latex].

21. decreasing on [latex]\left(-\infty ,-3\right)[/latex] and increasing on [latex]\left(-3,\infty \right)[/latex]

23. decreasing on [latex]\left(0,\infty \right)[/latex]

25.

27.

29.

31. [latex]g\left(x\right)=f\left(x - 1\right),h\left(x\right)=f\left(x\right)+1[/latex]

33. [latex]f\left(x\right)=|x - 3|-2[/latex]

35. [latex]f\left(x\right)=\sqrt{x+3}-1[/latex]

37. [latex]f\left(x\right)={\left(x - 2\right)}^{2}[/latex]

39. [latex]f\left(x\right)=|x+3|-2[/latex]

41. [latex]f\left(x\right)=-\sqrt{x}[/latex]

43. [latex]f\left(x\right)=-{\left(x+1\right)}^{2}+2[/latex]

45. [latex]f\left(x\right)=\sqrt{-x}+1[/latex]

47. The graph of [latex]g[/latex] is a vertical reflection (across the [latex]x[/latex] -axis) of the graph of [latex]f[/latex].

49. The graph of [latex]g[/latex] is a vertical stretch by a factor of 4 of the graph of [latex]f[/latex].

51. The graph of [latex]g[/latex] is a horizontal compression by a factor of [latex]\frac{1}{5}[/latex] of the graph of [latex]f[/latex].

53. The graph of [latex]g[/latex] is a horizontal stretch by a factor of 3 of the graph of [latex]f[/latex].

55. The graph of [latex]g[/latex] is a horizontal reflection across the [latex]y[/latex] -axis and a vertical stretch by a factor of 3 of the graph of [latex]f[/latex].

57. [latex]g\left(x\right)=|-4x|[/latex]

59. [latex]g\left(x\right)=\frac{1}{3{\left(x+2\right)}^{2}}-3[/latex]

61. [latex]g\left(x\right)=\frac{1}{2}{\left(x - 5\right)}^{2}+1[/latex]

63. The graph of the function [latex]f\left(x\right)={x}^{2}[/latex] is shifted to the left 1 unit, stretched vertically by a factor of 4, and shifted down 5 units.

65. The graph of [latex]f\left(x\right)=|x|[/latex] is stretched vertically by a factor of 2, shifted horizontally 4 units to the right, reflected across the horizontal axis, and then shifted vertically 3 units up.

67. The graph of the function [latex]f\left(x\right)={x}^{3}[/latex] is compressed vertically by a factor of [latex]\frac{1}{2}[/latex].

69. The graph of the function is stretched horizontally by a factor of 3 and then shifted vertically downward by 3 units.

71. The graph of [latex]f\left(x\right)=\sqrt{x}[/latex] is shifted right 4 units and then reflected across the vertical line [latex]x=4[/latex].

73.

75.

 

Section 1.10 Solutions

1. Each output of a function must have exactly one input for the function to be one-to-one.

3. Without any domain restriction, [latex]f(x)=x^{2}[/latex] does not have an inverse function as it fails the horizontal line test. But if we restrict the domain to be [latex]x>0[/latex], then we find that it passes the horizontal line test and therefore has an inverse function.

5. One-to-one functions are always increasing or decreasing. This ensures there is only one input for every output.

7. [latex]f(x)=a-x\\y=a-x\\x=a-y\\x+y=a\\y=a-x\\f^{-1}=a-x[/latex]

9. [latex]f^{-1}(x)=x-5[/latex]

11. [latex]f^{-1}(x)=3-x[/latex]

13. [latex]f^{-1}(x)=\frac{3-4x}{5x-2}[/latex]

15. Domain of [latex]f(x)[/latex] is [latex]\left[6,\infty \right)[/latex] and  [latex]f^{-1}=\sqrt{x}+6[/latex]

17. a. [latex]f(g(x))=\frac{3x}{1-x}[/latex] and [latex]g(f(x))=\frac{6x}{2-3x}[/latex]
b. [latex]f(x)[/latex] and [latex]g(x)[/latex] are not inverses.

19. [latex]f(g(x))=x[/latex] and [latex]g(f(x))=x[/latex]

21. Not one-to-one

23. One-to-one

25. Not one-to-one

27. [latex]f(0)=3[/latex]

29. [latex]f^{-1}(0)=2[/latex]

31.

33. [latex]\left[2,\infty \right)[/latex]

35.[latex]f^{-1}(7)=6[/latex]

37. [latex]f(-8)=-4[/latex]

39. [latex]f(1)=0[/latex]

41. [latex]f^{-1}(0)=1[/latex]

43.

[latex]x[/latex]	1	4	7	12	16
[latex]{f}^{-1}\left(x\right)[/latex]	3	6	9	13	14

45. [latex]{f}^{-1}\left(x\right)={\left(1+x\right)}^{1/3}[/latex]

47. [latex]{f}^{-1}\left(x\right)=\frac{5}{9}\left(x - 32\right)[/latex]. Given the Fahrenheit temperature, [latex]x[/latex], this formula allows you to calculate the Celsius temperature.

49. [latex]t\left(d\right)=\frac{d}{50}[/latex], [latex]t\left(180\right)=\frac{180}{50}[/latex]. The time for the car to travel 180 miles is 3.6 hours.