Section 2.1 Solutions

1. It is a second-degree equation (the highest variable exponent is 2).

3. We want to take advantage of the zero property of multiplication in the fact that if [latex]a\cdot b=0[/latex] then it must follow that each factor separately offers a solution to the product being zero [latex]a=0\text{ or }b=0[/latex].

5. One, when no linear term is present (no [latex]x[/latex] term), such as [latex]x^{2}=16[/latex]. Two, when the equation is already in the form [latex](ax+b)^{2}=d[/latex].

7. [latex]\text{ }x=6,x=3[/latex]

9. [latex]\text{ }x=-\dfrac{5}{2},x=-\dfrac{1}{3}[/latex]

11. [latex]\text{ }x=5,x=-5[/latex]

13. [latex]\text{ }x=-\dfrac{3}{2},x=\dfrac{3}{2}[/latex]

15. [latex]\text{ }x=-2,x=3[/latex]

17. [latex]\text{ }x=0,x=-\dfrac{3}{7}[/latex]

19. [latex]\text{ }x=6,x=-6[/latex]

21. [latex]\text{ }x=6,x=-4[/latex]

23. [latex]\text{ }x=1,x=-2[/latex]

25. [latex]\text{ }x=-2,x=11[/latex]

27. [latex]\text{ }x=3\pm\sqrt{22}[/latex]

29. [latex]\text{ }z=\dfrac{2}{3},z=-\dfrac{1}{2}[/latex]

31. [latex]\text{ }x=\dfrac{3\pm\sqrt{17}}{4}[/latex]

33. Not real

35. One rational

37. Two real; rational

39. [latex]\text{ }x=\dfrac{-1\pm\sqrt{17}}{2}[/latex]

41. [latex]\text{ }x=\dfrac{5\pm\sqrt{13}}{6}[/latex]

43. [latex]\text{ }x=\dfrac{-1\pm\sqrt{17}}{8}[/latex]

45. [latex]ax^{2}+bx+c=0 \\ x^2+\dfrac{b}{a}x=-\dfrac{c}{a} \\ x^{2}+\dfrac{b}{a}x+\dfrac{b^{2}}{4a^{2}}=-\dfrac{c}{a}+\dfrac{b}{4a^{2}} \\ \left(x+\dfrac{b}{2a}\right)^{2}=\dfrac{b^{2}-4ac}{4a^{2}} \\ x+\dfrac{b}{2a}=\pm \sqrt{\dfrac{b^{2}-4ac}{4a^{2}}} \\ x=\dfrac{-b \pm \sqrt{b^{2}-4ac}}{2a}[/latex]

47. [latex]\text{ }x(x+10)=119[/latex]; [latex]7\text{ }ft[/latex] and [latex]9\text{ }ft[/latex]

49. Maximum at [latex]x=70[/latex]

51. The quadratic equation would be [latex]\left(100x-0.5x^{2}\right)-(60x+300)=300[/latex]. The two values of [latex]x[/latex] are 20 and 60.

53. [latex]\text{ }3\text{ }ft[/latex]

Section 2.2 Solutions

1. Add the real parts together and the imaginary parts together.

3. i times i equals –1, which is not imaginary. (answers vary)

5. [latex]-8+2i[/latex]

7. [latex]14+7i[/latex]

9. [latex]-\frac{23}{29}+\frac{15}{29}i[/latex]

11. 2 real and 0 nonreal

13.

15.

17. [latex]8-i[/latex]

19. [latex]-11+4i[/latex]

21. [latex]2 - 5i[/latex]

23. [latex]6+15i[/latex]

25. [latex]-16+32i[/latex]

27. [latex]-4 - 7i[/latex]

29. 25

31. [latex]2-\frac{2}{3}i[/latex]

33. [latex]4 - 6i[/latex]

35. [latex]\frac{2}{5}+\frac{11}{5}i[/latex]

37. 15i

39. [latex]1+i\sqrt{3}[/latex]

41. 1

43. –1

45. 128i

47. [latex]{\left(\frac{\sqrt{3}}{2}+\frac{1}{2}i\right)}^{6}=-1[/latex]

49. 3i

51. 0

53. 5 – 5i

55. –2i

57. [latex]\frac{9}{2}-\frac{9}{2}i[/latex]

Section 2.3 Solutions

1. When written in that form, the vertex can be easily identified.

3. If [latex]a=0[/latex] then the function becomes a linear function.

5. If possible, we can use factoring. Otherwise, we can use the quadratic formula.

7. [latex]f\left(x\right)={\left(x+1\right)}^{2}-2[/latex], Vertex [latex]\left(-1,-4\right)[/latex]

9. [latex]f\left(x\right)={\left(x+\frac{5}{2}\right)}^{2}-\frac{33}{4}[/latex], Vertex [latex]\left(-\frac{5}{2},-\frac{33}{4}\right)[/latex]

11. [latex]f\left(x\right)=3{\left(x - 1\right)}^{2}-12[/latex], Vertex [latex]\left(1,-12\right)[/latex]

13. [latex]f\left(x\right)=3{\left(x-\frac{5}{6}\right)}^{2}-\frac{37}{12}[/latex], Vertex [latex]\left(\frac{5}{6},-\frac{37}{12}\right)[/latex]

15. Minimum is [latex]-\frac{17}{2}[/latex] and occurs at [latex]\frac{5}{2}[/latex]. Axis of symmetry is [latex]x=\frac{5}{2}[/latex].

17. Minimum is [latex]-\frac{17}{16}[/latex] and occurs at [latex]-\frac{1}{8}[/latex]. Axis of symmetry is [latex]x=-\frac{1}{8}[/latex].

19. Minimum is [latex]-\frac{7}{2}[/latex] and occurs at –3. Axis of symmetry is [latex]x=-3[/latex].

21. Domain is [latex]\left(-\infty ,\infty \right)[/latex]. Range is [latex]\left[2,\infty \right)[/latex].

23. Domain is [latex]\left(-\infty ,\infty \right)[/latex]. Range is [latex]\left[-5,\infty \right)[/latex].

25. Domain is [latex]\left(-\infty ,\infty \right)[/latex]. Range is [latex]\left[-12,\infty \right)[/latex].

27. [latex]\left\{2i\sqrt{2},-2i\sqrt{2}\right\}[/latex]

29. [latex]\left\{3i\sqrt{3},-3i\sqrt{3}\right\}[/latex]

31. [latex]\left\{2+i,2-i\right\}[/latex]

33. [latex]\left\{2+3i,2 - 3i\right\}[/latex]

35. [latex]\left\{5+i,5-i\right\}[/latex]

37. [latex]\left\{2+2\sqrt{6}, 2 - 2\sqrt{6}\right\}[/latex]

39. [latex]\left\{-\frac{1}{2}+\frac{3}{2}i, -\frac{1}{2}-\frac{3}{2}i\right\}[/latex]

41. [latex]\left\{-\frac{3}{5}+\frac{1}{5}i, -\frac{3}{5}-\frac{1}{5}i\right\}[/latex]

43. [latex]\left\{-\frac{1}{2}+\frac{1}{2}i\sqrt{7}, -\frac{1}{2}-\frac{1}{2}i\sqrt{7}\right\}[/latex]

45. [latex]f\left(x\right)={x}^{2}-4x+4[/latex]

47. [latex]f\left(x\right)={x}^{2}+1[/latex]

49. [latex]f\left(x\right)=\frac{6}{49}{x}^{2}+\frac{60}{49}x+\frac{297}{49}[/latex]

51. [latex]f\left(x\right)=-{x}^{2}+1[/latex]

53. Vertex [latex]\left(1,\text{ }-1\right)[/latex], Axis of symmetry is [latex]x=1[/latex]. Intercepts are [latex]\left(0,0\right), \left(2,0\right)[/latex].

55. Vertex [latex]\left(\frac{5}{2},\frac{-49}{4}\right)[/latex], Axis of symmetry is [latex]\left(0,-6\right),\left(-1,0\right),\left(6,0\right)[/latex].

57. Vertex [latex]\left(\frac{5}{4}, -\frac{39}{8}\right)[/latex], Axis of symmetry is [latex]x=\frac{5}{4}[/latex]. Intercepts are [latex]\left(0, -8\right)[/latex].

59. [latex]f\left(x\right)={x}^{2}-4x+1[/latex]

61. [latex]f\left(x\right)=-2{x}^{2}+8x - 1[/latex]

63. [latex]f\left(x\right)=\frac{1}{2}{x}^{2}-3x+\frac{7}{2}[/latex]

65. [latex]f\left(x\right)={x}^{2}+1[/latex]

67. [latex]f\left(x\right)=2-{x}^{2}[/latex]

69. [latex]f\left(x\right)=2{x}^{2}[/latex]

71. The graph is shifted up or down (a vertical shift).

73. 50 feet

75. Domain is [latex]\left(-\infty ,\infty \right)[/latex]. Range is [latex]\left[-2,\infty \right)[/latex].

77. Domain is [latex]\left(-\infty ,\infty \right)[/latex] Range is [latex]\left(-\infty ,11\right][/latex].

79. [latex]f\left(x\right)=2{x}^{2}-1[/latex]

81. [latex]f\left(x\right)=3{x}^{2}-9[/latex]

83. [latex]f\left(x\right)=5{x}^{2}-77[/latex]

85. 50 feet by 50 feet. Maximize [latex]f\left(x\right)=-{x}^{2}+100x[/latex].

87. 125 feet by 62.5 feet. Maximize [latex]f\left(x\right)=-2{x}^{2}+250x[/latex].

89. 6 and –6; product is –36; maximize [latex]f\left(x\right)={x}^{2}+12x[/latex].

91. 2909.56 meters

93. $10.70

Section 2.4 Solutions

1. The x-intercept is where the graph of the function crosses the x-axis, and the zero of the function is the input value for which [latex]f\left(x\right)=0[/latex].

3. The maximum number of turning points is always 1 less than the degree.

5. The factored form is used to find the zeros.  The behavior at each zero can be found from the factored form, and this aids in graphing.

7. Degree 5 polynomial; [latex]a_{n}=6[/latex]

9. Not a polynomial

11. Degree 4 polynomial; [latex]a_{n}=2[/latex]

13. Not a polynomial

15. y-intercept is [latex]\left(0,12\right)[/latex], t-intercepts are [latex]\left(1,0\right);\left(-2,0\right);\text{and }\left(3,0\right)[/latex].

17. y-intercept is [latex]\left(0,16\right)[/latex]. x-intercepts are [latex]\left(2,0\right)[/latex] and [latex]\left(-2,0\right)[/latex].

19. y-intercept is [latex]\left(0,0\right)[/latex]. x-intercepts are [latex]\left(0,0\right),\left(4,0\right)[/latex], and [latex]\left(-2, 0\right)[/latex].

21.  2

23.  1

25.  4

27. Yes. Number of turning points is 2. Least possible degree is 3.

29. Yes. Number of turning points is 1. Least possible degree is 2.

31. Yes. Number of turning points is 0. Least possible degree is 1.

33. Yes. Number of turning points is 0. Least possible degree is 1.

33.  0 with multiplicity 2 and behavior: [latex]4x^{2}[/latex]
[latex]–2[/latex] with multiplicity 2 and behavior: [latex]4(x+2)^{2}[/latex]

35.[latex]\text{ }-\frac{2}{3}[/latex] with multiplicity 5 and behavior: [latex]\frac{289}{9}(3x+2)^3[/latex]
5 with multiplicity 2 and behavior: [latex]4913(x-5)^{2}[/latex]

37. 0 with multiplicity 4 and behavior: [latex]-2x^{4}[/latex]
2 with multiplicity 1 and behavior: [latex]48(x-2)[/latex]
-1 with multiplicity 1 and behavior: [latex]-(x+1)[/latex]

39. [latex]\text{ }\frac{3}{2}[/latex] with multiplicity 2 and behavior: [latex]\frac{27}{8}(2x-3)^2[/latex]
0 with multiplicity 3 and behavior: [latex]9x^{3}[/latex]

41. 0 with multiplicity 6 and behavior: [latex]16x^{6}[/latex]
[latex]\frac{2}{3}[/latex] with multiplicity 2 and behavior: [latex]\frac{729}{16}(3x-2)^{2}[/latex]

43. zeros [latex]\left(1, 0\right)[/latex] with multiplicity 2, [latex]\left(-4, 0\right)[/latex] with multiplicity 1, y-intercept [latex]\left(0, 4\right)[/latex]. As [latex]x\to -\infty [/latex] , [latex]f\left(x\right)\to -\infty [/latex] , as [latex]x\to \infty [/latex] , [latex]f\left(x\right)\to \infty [/latex].
At [latex]x=-4[/latex] the behavior is: [latex]25(x+4)[/latex]
At [latex]x=1[/latex] the behavior is: [latex]5(x-1)^{2}[/latex].

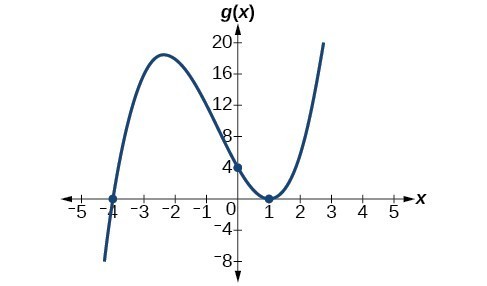
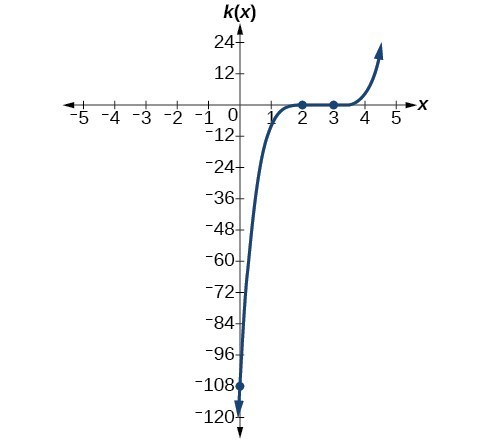
45. zeros [latex]\left(3,0\right)[/latex] with multiplicity 3, [latex]\left(2,0\right)[/latex] with multiplicity 2, y-intercept [latex]\left(0,-108\right)[/latex] . As [latex]x\to -\infty [/latex], [latex]f\left(x\right)\to -\infty [/latex] , as [latex]x\to \infty [/latex] , [latex]f\left(x\right)\to \infty [/latex].
At [latex]x=2[/latex] the behavior is: [latex]-(x-2)^{2}[/latex].
At [latex]x=3[/latex] the behavior is: [latex](x-3)^{3}[/latex].

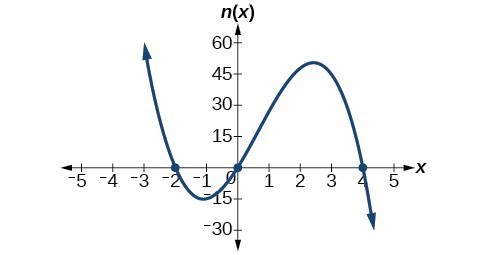
47. x-intercepts [latex]\left(0, 0\right),\left(-2, 0\right),\left(4, 0\right)[/latex] with multiplicity 1, y-intercept [latex]\left(0, 0\right)[/latex]. As [latex]x\to -\infty [/latex] , [latex]f\left(x\right)\to \infty [/latex] , as [latex]x\to \infty [/latex] , [latex]f\left(x\right)\to -\infty [/latex].
At [latex]x=0[/latex] the behavior is: [latex]24x[/latex]
At [latex]x=-2[/latex] the behavior is: [latex]-36(x+2)[/latex]
At [latex]x=4[/latex] the behavior is: [latex]-72(x-4)[/latex].

49. The y-intercept is [latex]\left(0, 0\right)[/latex]. The zeros are [latex]\left(0, 0\right),\text{ }\left(2, 0\right)[/latex]. [latex]\text{As }x\to -\infty ,f\left(x\right)\to \infty ,\text{ as }x\to \infty ,f\left(x\right)\to \infty [/latex]
At [latex]x=0[/latex] the behavior is: [latex]-2x^3[/latex]
At [latex]x=2[/latex] the behavior is: [latex]8(x-2)[/latex].

51. [latex]f\left(x\right)=x^{2}+x-6[/latex]

53. [latex]f\left(x\right)=x(x-2)^{2}[/latex] or [latex]f\left(x\right)=x^{2}(x-2)[/latex]

55. [latex]f\left(x\right)=x^{4}+1[/latex]

57. [latex]f\left(x\right)=-\frac{2}{9}(x+3)(x+1)(x-3)[/latex]

59. [latex]f\left(x\right)=\frac{1}{4}(x+2)^{2}(x-3)[/latex]

61. [latex]f\left(x\right)=-\frac{1}{8}(x+4)(x+2)(x-1)(x-3)[/latex]

63. [latex]f\left(x\right)=\frac{1}/{6}(x+3)(x+2)(x-1)^3[/latex]

65. [latex]f\left(2\right)=-10[/latex] and [latex]f\left(4\right)=28[/latex]. Sign change confirms.

67. [latex]f\left(1\right)=3[/latex] and [latex]f\left(3\right)=-77[/latex]. Sign change confirms.

69. [latex]f\left(0.01\right)=1.000001[/latex] and [latex]f\left(0.1\right)=-7.999[/latex]. Sign change confirms.

Section 2.5 Solutions

1. The binomial is a factor of the polynomial.

3. [latex]x+6+\frac{5}{x - 1}\text{,}\text{quotient:}x+6\text{,}\text{remainder:}\text{5}[/latex]

5. [latex]3x+2\text{,}\text{ quotient: }3x+2\text{, }\text{remainder: 0}[/latex]

7. [latex]x - 5\text{,}\text{quotient: }x - 5\text{,}\text{remainder: }\text{0}[/latex]

9. [latex]2x - 7+\frac{16}{x+2}\text{,}\text{quotient: }\text{ }2x - 7\text{,}\text{remainder: }\text{16}[/latex]

11. [latex]x - 2+\frac{6}{3x+1}\text{,}\text{quotient: }x - 2\text{,}\text{remainder: }\text{6}[/latex]

13. [latex]2{x}^{2}-3x+5\text{,}\text{quotient:}2{x}^{2}-3x+5\text{,}\text{remainder: }\text{0}[/latex]

15. [latex]2{x}^{2}+2x+1+\frac{10}{x - 4}[/latex]

17. [latex]2{x}^{2}-7x+1-\frac{2}{2x+1}[/latex]

19. [latex]3{x}^{2}-11x+34-\frac{106}{x+3}[/latex]

21. [latex]{x}^{2}+5x+1[/latex]

23. [latex]4{x}^{2}-21x+84-\frac{323}{x+4}[/latex]

25. [latex]{x}^{2}-14x+49[/latex]

27. [latex]3{x}^{2}+x+\frac{2}{3x - 1}[/latex]

29. [latex]{x}^{3}-3x+1[/latex]

31. [latex]{x}^{3}-{x}^{2}+2[/latex]

33. [latex]{x}^{3}-6{x}^{2}+12x - 8[/latex]

35. [latex]{x}^{3}-9{x}^{2}+27x - 27[/latex]

37. [latex]2{x}^{3}-2x+2[/latex]

39. [latex]\left(x - 1\right)\left({x}^{2}+2x+4\right)[/latex]

41. [latex]\left(x - 5\right)\left({x}^{2}+x+1\right)[/latex]

43. [latex]\text{Quotient: }4{x}^{2}+8x+16\text{,}\text{remainder: }-1[/latex]

45. [latex]\text{Quotient: }3{x}^{2}+3x+5\text{,}\text{remainder: }0[/latex]

47. [latex]\text{Quotient: }{x}^{3}-2{x}^{2}+4x - 8\text{,}\text{remainder: }-6[/latex]

49. [latex]{x}^{6}-{x}^{5}+{x}^{4}-{x}^{3}+{x}^{2}-x+1[/latex]

51. [latex]{x}^{3}-{x}^{2}+x - 1+\frac{1}{x+1}[/latex]

53. [latex]1+\frac{1+i}{x-i}[/latex]

55. [latex]1+\frac{1-i}{x+i}[/latex]

57. [latex]{x}^{2}-ix - 1+\frac{1-i}{x-i}[/latex]

59. [latex]2{x}^{2}+3[/latex]

61. [latex]2x+3[/latex]

63. [latex]x+2[/latex]

65. [latex]x - 3[/latex]

67. [latex]3{x}^{2}-2[/latex]

Section 2.6 Solutions

1. The theorem can be used to evaluate a polynomial.

3. Rational zeros can be expressed as fractions whereas real zeros include irrational numbers.

5. Polynomial functions can have repeated zeros, so the fact that number is a zero doesn’t preclude it being a zero again.

7. –106

9. 0

11. 255

13. –1

15. –2, 1, [latex]\frac{1}{2}[/latex]

17. –2

19. –3

21. [latex]-\frac{5}{2}, \sqrt{6}, -\sqrt{6}[/latex]

23. [latex]2, -4, -\frac{3}{2}[/latex]

25. 4, –4, –5

27. [latex]5, -3, -\frac{1}{2}[/latex]

29. [latex]\frac{1}{2}, \frac{1+\sqrt{5}}{2}, \frac{1-\sqrt{5}}{2}[/latex]

31. [latex]\frac{3}{2}[/latex]

33. 2, 3, –1, –2

35. [latex]\frac{1}{2}, -\frac{1}{2}, 2, -3[/latex]

37. [latex]-1, -1, \sqrt{5}, -\sqrt{5}[/latex]

39. [latex]-\frac{3}{4}, -\frac{1}{2}[/latex]

41. [latex]2, 3+2i, 3 - 2i[/latex]

43. [latex]-\frac{2}{3}, 1+2i, 1 - 2i[/latex]

45. [latex]-\frac{1}{2}, 1+4i, 1 - 4i[/latex]

47. 1 positive, 1 negative

49. 3 or 1 positive, 0 negative

51. 0 positive, 3 or 1 negative

53. 2 or 0 positive, 2 or 0 negative

55. 2 or 0 positive, 2 or 0 negative

57. [latex]\pm 5, \pm 1, \pm \frac{5}{2}[/latex]

59. [latex]\pm 1, \pm \frac{1}{2}, \pm \frac{1}{3}, \pm \frac{1}{6}[/latex]

61. [latex]1, \frac{1}{2}, -\frac{1}{3}[/latex]

63. [latex]2, \frac{1}{4}, -\frac{3}{2}[/latex]

65. [latex]\frac{5}{4}[/latex]

67. [latex]f\left(x\right)=\frac{4}{9}\left({x}^{3}+{x}^{2}-x - 1\right)[/latex]

69. [latex]f\left(x\right)=-\frac{1}{5}\left(4{x}^{3}-x\right)[/latex]

71. 8 by 4 by 6 inches

73. 5.5 by 4.5 by 3.5 inches

75. 8 by 5 by 3 inches

77. Radius = 6 meters, Height = 2 meters

79. Radius = 2.5 meters, Height = 4.5 meters

Section 2.7 Solutions

1. Critical points are where the polynomial is equal to zero. It allows you to create the different intervals on the number line for testing.

3. [latex]\left(-3,4\right)[/latex]

5. [latex]\left(-\infty,1\right]\cup\left[\frac{4}{3},\infty\right)[/latex]

7. [latex]\left(-4,-1\right)[/latex]

9. [latex]\left[-8,-2\right]\cup\left[3,\infty\right)[/latex]

11. [latex]\left(-\infty,-8\right)\cup\left(-8,-7\right)\cup\left(-5,\infty\right)[/latex]

13. [latex]\left(-\infty,-4\right]\cup\left[-3,\frac{1}{2}\right)[/latex]

15. [latex]\left(-5,-1\right)\cup\left(1,2\right)[/latex]

17. [latex]\left(1,7\right)[/latex]

19. [latex]\left[-\infty,-4\right]\cup\left[\frac{3}{2},\infty\right)[/latex]

21. [latex]\left(-6,7\right][/latex]

23. [latex]\left[-5,-3\right]\cup\left(-2,\infty\right)[/latex]

25. [latex]\left(-\infty,-\frac{2}{3}\right)\cup\left[2,7\right][/latex]

27. [latex]\left(-\infty,-6\right]\cup\left(-3,8\right)[/latex]

29. [latex]\left(-7,3\right)[/latex]

31. [latex]\left(-3,-2\right)\cup\left(\frac{1}{2},\infty\right)[/latex]

Section 2.8 Solutions

1. The rational function will be represented by a quotient of polynomial functions.

3. The numerator and denominator must have a common factor.

5. Yes. The numerator of the formula of the functions would have only complex roots and/or factors common to both the numerator and denominator.

7. [latex]\text{All reals }x\ne -1, 1[/latex]

9. [latex]\text{All reals }x\ne -1, -2, 1, 2[/latex]

11. V.A. at [latex]x=-\frac{2}{5}[/latex]; H.A. at [latex]y=0[/latex]; Domain is all reals [latex]x\ne -\frac{2}{5}[/latex]

13. V.A. at [latex]x=4, -9[/latex]; H.A. at [latex]y=0[/latex]; Domain is all reals [latex]x\ne 4, -9[/latex]

15. V.A. at [latex]x=0, 4, -4[/latex]; H.A. at [latex]y=0[/latex]; Domain is all reals [latex]x\ne 0,4, -4[/latex]

17. V.A. at [latex]x=-5[/latex]; H.A. at [latex]y=0[/latex]; Domain is all reals [latex]x\ne 5,-5[/latex]

19. V.A. at [latex]x=\frac{1}{3}[/latex]; H.A. at [latex]y=-\frac{2}{3}[/latex]; Domain is all reals [latex]x\ne \frac{1}{3}[/latex].

21. none

23. [latex]x\text{-intercepts none, }y\text{-intercept }\left(0,\frac{1}{4}\right)[/latex]

25. Local behavior: [latex]x\to -{\frac{1}{2}}^{+},f\left(x\right)\to -\infty ,x\to -{\frac{1}{2}}^{-},f\left(x\right)\to \infty [/latex]

End behavior: [latex]x\to \pm \infty ,f\left(x\right)\to \frac{1}{2}[/latex]

27. Local behavior: [latex]x\to {6}^{+},f\left(x\right)\to -\infty ,x\to {6}^{-},f\left(x\right)\to \infty [/latex], End behavior: [latex]x\to \pm \infty ,f\left(x\right)\to -2[/latex]

29. Local behavior: [latex]x\to -{\frac{1}{3}}^{+},f\left(x\right)\to \infty ,x\to -{\frac{1}{3}}^{-}[/latex], [latex]f\left(x\right)\to -\infty ,x\to {\frac{5}{2}}^{-},f\left(x\right)\to \infty ,x\to {\frac{5}{2}}^{+}[/latex] , [latex]f\left(x\right)\to -\infty [/latex]

End behavior: [latex]x\to \pm \infty\\ [/latex], [latex]f\left(x\right)\to \frac{1}{3}[/latex]

31. [latex]y=2x+4[/latex]

33. [latex]y=2x[/latex]

35. [latex]V.A.\text{ }x=0,H.A.\text{ }y=2[/latex]

37. [latex]V.A.\text{ }x=2,\text{ }H.A.\text{ }y=0[/latex]

39. [latex]V.A.\text{ }x=-4,\text{ }H.A.\text{ }y=2;\left(\frac{3}{2},0\right);\left(0,-\frac{3}{4}\right)[/latex]

41. [latex]V.A.\text{ }x=2,\text{ }H.A.\text{ }y=0,\text{ }\left(0,1\right)[/latex]

43. [latex]V.A.\text{ }x=-4,\text{ }x=\frac{4}{3},\text{ }H.A.\text{ }y=1;\left(5,0\right);\left(-\frac{1}{3},0\right);\left(0,\frac{5}{16}\right)[/latex]

45. [latex]V.A.\text{ }x=-1,\text{ }H.A.\text{ }y=1;\left(-3,0\right);\left(0,3\right)[/latex]

47. [latex]V.A.\text{ }x=4,\text{ }S.A.\text{ }y=2x+9;\left(-1,0\right);\left(\frac{1}{2},0\right);\left(0,\frac{1}{4}\right)[/latex]

49. [latex]V.A.\text{ }x=-2,\text{ }x=4,\text{ }H.A.\text{ }y=1,\left(1,0\right);\left(5,0\right);\left(-3,0\right);\left(0,-\frac{15}{16}\right)[/latex]

51. [latex]y=50\frac{{x}^{2}-x - 2}{{x}^{2}-25}[/latex]

53. [latex]y=7\frac{{x}^{2}+2x - 24}{{x}^{2}+9x+20}[/latex]

55. [latex]y=\frac{1}{2}\frac{{x}^{2}-4x+4}{x+1}[/latex]

57. [latex]y=4\frac{x - 3}{{x}^{2}-x - 12}[/latex]

59. [latex]y=-9\frac{x - 2}{{x}^{2}-9}[/latex]

61. [latex]y=\frac{1}{3}\frac{{x}^{2}+x - 6}{x - 1}[/latex]

63. [latex]y=-6\frac{{\left(x - 1\right)}^{2}}{\left(x+3\right){\left(x - 2\right)}^{2}}[/latex]

65.

x	2.01	2.001	2.0001	1.99	1.999
y	100	1,000	10,000	–100	–1,000

x	10	100	1,000	10,000	100,000
y	.125	.0102	.001	.0001	.00001

Vertical asymptote [latex]x=2[/latex], Horizontal asymptote [latex]y=0[/latex]

67.

x	–4.1	–4.01	–4.001	–3.99	–3.999
y	82	802	8,002	–798	–7998

x	10	100	1,000	10,000	100,000
y	1.4286	1.9331	1.992	1.9992	1.999992

Vertical asymptote [latex]x=-4[/latex], Horizontal asymptote [latex]y=2[/latex]

69.

x	–.9	–.99	–.999	–1.1	–1.01
y	81	9,801	998,001	121	10,201

x	10	100	1,000	10,000	100,000
y	.82645	.9803	.998	.9998

Vertical asymptote [latex]x=-1[/latex], Horizontal asymptote [latex]y=1[/latex]

71. [latex]\left(\frac{3}{2},\infty \right)[/latex]

73. [latex]\left(-2,1\right)\cup \left(4,\infty \right)[/latex]

75. [latex]\left(2,4\right)[/latex]

77. [latex]\left(2,5\right)[/latex]

79. [latex]\left(-1,\text{1}\right)[/latex]

81. [latex]C\left(t\right)=\frac{8+2t}{300+20t}[/latex]

83. After about 6.12 hours.

85. [latex]A\left(x\right)=50{x}^{2}+\frac{800}{x}[/latex]. 2 by 2 by 5 feet.

87. [latex]A\left(x\right)=\pi {x}^{2}+\frac{100}{x}[/latex]. Radius = 2.52 meters.