Section 7.2: The Inverse Trigonometric Functions (Continued)

Learning Outcomes

Find exact values of composite functions with inverse trigonometric functions.
Write a trigonometric expression as an algebraic expression.

Evaluating Compositions of the Form $f^{−1}(g(x))$

Now that we can compose a trigonometric function with its inverse, we can explore how to evaluate a composition of a trigonometric function and the inverse of another trigonometric function. We will begin with compositions of the form $f^{−1}(g(x))$ . For special values of x, we can exactly evaluate the inner function and then the outer, inverse function. However, we can find a more general approach by considering the relation between the two acute angles of a right triangle where one is θ, making the other $\frac{\pi}{2}−\theta$ . Consider the sine and cosine of each angle of the right triangle in Figure 10.

An illustration of a right triangle with angles theta and pi/2 - theta. Opposite the angle theta and adjacent the angle pi/2-theta is the side a. Adjacent the angle theta and opposite the angle pi/2 - theta is the side b. The hypoteneuse is labeled c.

Figure 10. Right triangle illustrating the cofunction relationships

Because $\cos\theta=\frac{b}{c}=\sin\left(\frac{\pi}{2}−\theta\right)$ , we have $\sin^{−1}(\cos\theta)=\frac{\pi}{2}−\theta\text{ if }0\leq\theta\leq\pi$ . If θ is not in this domain, then we need to find another angle that has the same cosine as θ and does belong to the restricted domain; we then subtract this angle from $\frac{\pi}{2}$ . Similarly, $\sin\theta=\frac{a}{c}=\cos\left(\frac{\pi}{2}−\theta\right)$ , so $\cos^{−1}(\sin\theta)=\frac{\pi}{2}−\theta\text{ if }−\frac{\pi}{2}\leq\theta\leq\frac{\pi}{2}$ . These are just the function-cofunction relationships presented in another way.

How To: Given functions of the form $\sin^{−1}(\cos x)\text{ and }\cos^{−1}(\sin x)$ , evaluate them.

If x is in [0,π], then $\sin^{−1}(\cos x)=\frac{\pi}{2}−x$ .
If x is not in [0,π], then find another angle y in [0,π] such that $\cos y=\cos x$ .

$\sin^{−1}(\cos x)=\frac{\pi}{2}−y$
If x is in $\left[−\frac{\pi}{2},\frac{\pi}{2}\right]$ , then $\cos^{−1}(\sin x)=\frac{\pi}{2}−x$ .
If x is not in $\left[−\frac{\pi}{2},\frac{\pi}{2}\right]$ , then find another angle y in $\left[−\frac{\pi}{2}\text{, }\frac{\pi}{2}\right]$ such that $\sin y=\sin x$ .

$\cos^{−1}(\sin x)=\frac{\pi}{2}−y$

Example 6: Evaluating the Composition of an Inverse Sine with a Cosine

Evaluate $\sin^{−1}(\cos(\frac{13\pi}{6}))$

by direct evaluation.
by the method described previously.

Show Solution

Here, we can directly evaluate the inside of the composition.
$\begin{align}\cos\left(\frac{13\pi}{6}\right)&=\cos\left(\frac{\pi}{6}+2\pi\right) \\ &=\cos\left(\frac{\pi}{6}\right) \\ &=\frac{\sqrt{3}}{2} \end{align}$

Now, we can evaluate the inverse function as we did earlier.

$\sin^{−1}\left(\frac{\sqrt{3}}{2}\right)=\frac{\pi}{3}$
We have $x=\frac{13\pi}{6}$ , $y=\frac{\pi}{6}$ , and
$\begin{align}\sin^{−1}\left(\cos\left(\frac{13\pi}{6}\right)\right)=\frac{\pi}{2}−\frac{\pi}{6} =\frac{\pi}{3} \end{align}$

Try It

Evaluate $\cos^{−1}(\sin(−\frac{11\pi}{4}))$ .

Show Solution

$\frac{3\pi}{4}$

Try It

Evaluating Compositions of the Form $f(g^{−1}(x))$

To evaluate compositions of the form $f(g^{−1}(x))$ , where f and g are any two of the functions sine, cosine, or tangent and x is any input in the domain of $g−1$ , we have exact formulas, such as $\sin\left({\cos}^{−1}x\right)=\sqrt{1−{x}^{2}}$ . When we need to use them, we can derive these formulas by using the trigonometric relations between the angles and sides of a right triangle, together with the use of Pythagoras’s relation between the lengths of the sides. We can use the Pythagorean identity, $\sin^{2}x+cos^{2}x=1$ , to solve for one when given the other. We can also use the inverse trigonometric functions to find compositions involving algebraic expressions.

Example 7: Evaluating the Composition of a Sine with an Inverse Cosine

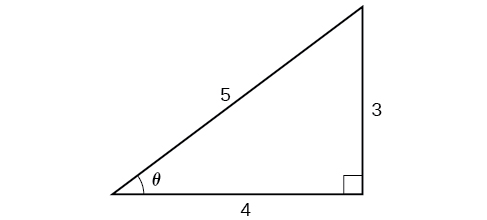
Find an exact value for $\sin\left(\cos^{−1}\left(\frac{4}{5}\right)\right)$ .

Show Solution

Beginning with the inside, we can say there is some angle such that $\theta=\cos^{−1}(\frac{4}{5})$ , which means $\cos\theta=\frac{4}{5}$ , and we are looking for $\sin\theta$ . We can use the Pythagorean identity to do this.

$\begin{align} &\sin^{2}\theta+\cos^{2}\theta=1 && \text{Use our known value for cosine.} \\ &\sin^{2}\theta+\left(\frac{4}{5}\right)^{2}=1 && \text{Solve for sine.} \\ &\sin^{2}\theta=1−\frac{16}{25} \\ &\sin\theta=\pm\sqrt{\frac{9}{25}}=\pm\frac{3}{5} \end{align}$

Since $\theta=\cos^{−1}(\frac{4}{5})$ is in quadrant I, $\sin{\theta}$ must be positive, so the solution is $\frac{3}{5}$ . See Figure 11.

An illustration of a right triangle with an angle theta. Oppostie the angle theta is a side with length 3. Adjacent the angle theta is a side with length 4. The hypoteneuse has angle of length 5.

Figure 11. Right triangle illustrating that if $\cos\theta=\frac{4}{5}$ , then $\sin\theta=\frac{3}{5}$

We know that the inverse cosine always gives an angle on the interval [0, π], so we know that the sine of that angle must be positive; therefore $\sin\left(\cos^{−1}\left(\frac{4}{5}\right)\right)=\sin\theta=\frac{3}{5}$ .

Try It

Evaluate $\cos(\tan^{−1}(\frac{5}{12}))$ .

Show Solution

$\frac{12}{13}$

Example 8: Evaluating the Composition of a Sine with an Inverse Tangent

Find an exact value for $\sin\left(\tan^{−1}\left(\frac{7}{4}\right)\right)$ .

Show Solution

While we could use a similar technique as in Example 6, we will demonstrate a different technique here. From the inside, we know there is an angle such that $\tan\theta=\frac{7}{4}$ . We can envision this as the opposite and adjacent sides on a right triangle, as shown in Figure 12.

An illustration of a right triangle with angle theta. Adjacent the angle theta is a side with length 4. Opposite the angle theta is a side with length 7.

Figure 12. A right triangle with two sides known

Using the Pythagorean Theorem, we can find the hypotenuse of this triangle.

$\begin{gathered}4^{2}+7^{2}=\text{hypotenuse}^{2} \\ \text{hypotenuse}=\sqrt{65} \end{gathered}$

Try It

Evaluate $\cos(\sin^{−1}(\frac{7}{9}))$ .

Show Solution

$\frac{4\sqrt{2}}{9}$

Try It

Example 9: Finding the Cosine of the Inverse Sine of an Algebraic Expression

Use a right triangle to write $\cos\left(\sin^{−1}\left(\frac{6}{x}\right)\right)$ as an algebraic expression. Assume that $x$ is positive and in the domain of the inverse sine.

Show Solution

Let $\theta=\sin^{-1}\left(\frac{6}{x}\right)\text{, so }\sin\theta=\frac{6}{x}$ . We can represent this on a triangle. Using the definition for sine, the opposite side is 6 and the hypotenuse is $x$ . Then we can find the adjacent side:
Right triangle with opposite side of 6 and hypotenuse of x

$\begin{align}&a^{2}+6^{2}=x^{2}&& \text{Use the Pythagorean Theorem.} \\ &a^{2}=x^{2}-36 && \text{Isolate a.} \\ &a=\pm\sqrt{x^{2}-36} &&\text{Take the square root of both sides}\\&a=\sqrt{x^{2}-36} && \text{Take the positive root. This is the adjacent side.} \end{align}$

Now that we have the triangle complete and latex]\theta=\sin^{-1}\left(\frac{6}{x}\right)[/latex], we need to find the cosine. The definition of cosine is the adjacent side divided by the hypotenuse, so $\cos\left(\sin^{−1}\left(\frac{6}{x}\right)\right)=\frac{\sqrt{x^{2}-36}}{x}$ .

Try It

Use a right triangle to write $\sin\left(\tan^{−1}\left(4x\right)\right)$ as an algebraic expression. Assume that $x$ is positive and that the given inverse trigonometric function is defined for the expression in $x$ .

Show Solution

$\frac{4x}{\sqrt{16x^{2}+1}}$

Try It

Key Concepts

In function composition, if the inside function is an inverse trigonometric function, then there are exact expressions; for example, $\sin\left(\cos^{−1}\left(x\right)\right)=\sqrt{1−x^{2}}$ .
If the inside function is a trigonometric function, then the only possible combinations are $\sin^{−1}\left(\cos x\right)=\frac{\pi}{2}−x$ if $0\leq x\leq\pi$ and $\cos^{−1}\left(\sin x\right)=\frac{\pi}{2}−x$ if $−\frac{\pi}{2}\leq x \leq\frac{\pi}{2}$ .
When evaluating the composition of a trigonometric function with an inverse trigonometric function, draw a reference triangle to assist in determining the ratio of sides that represents the output of the trigonometric function.
When evaluating the composition of a trigonometric function with an inverse trigonometric function, you may use trig identities to assist in determining the ratio of sides.

Section 7.2 Homework Exercises

For the following exercises, find the exact value, if possible, without a calculator. If it is not possible, explain why.

1. $\cos^{−1}(\sin(\pi))$

2. $\sin^{−1}(\cos(\pi))$

3. $\tan^{−1}(\sin(\pi))$

4. $\cos^{−1}\left(\sin\left(\frac{\pi}{3}\right)\right)$

5. $\tan^{−1}\left(\sin\left(\frac{\pi}{3}\right)\right)$

6. $\sin^{−1}\left(\cos\left(\frac{−\pi}{2}\right)\right)$

7. $\tan^{−1}\left(\sin\left(\frac{4\pi}{3}\right)\right)$

8. $\sin^{−1}\left(\sin\left(\frac{5\pi}{6}\right)\right)$

9. $\tan^{−1}\left(\sin\left(\frac{−5\pi}{2}\right)\right)$

10. $\cos\left(\sin^{−1}\left(\frac{4}{5}\right)\right)$

11. $\sin\left(\cos^{−1}\left(\frac{3}{5}\right)\right)$

12. $\sin\left(\tan^{−1}\left(\frac{4}{3}\right)\right)$

13. $\cos\left(\tan^{−1}\left(\frac{12}{5}\right)\right)$

14. $\cos\left(\sin^{−1}\left(\frac{1}{2}\right)\right)$

For the following exercises, use a right triangle to write the expression as an algebraic expression. Assume that x is positive and in the domain of the given inverse trigonometric function.

15. $\tan\left(\sin^{−1}\left(x\right)\right)$

16. $\sin\left(\cos^{−1}\left(x\right)\right)$

17. $\cos\left(\sin^{−1}\left(\frac{1}{x}\right)\right)$

18. $\cos\left(\tan^{−1}\left(3x\right)\right)$

19. $\sin\left(\tan^{−1}\left(2x\right)\right)$

20. $\tan\left(\cos^{−1}\left(4x\right)\right)$

Chapter 7: Analytic Trigonometry