Section 1.4: Circles

Learning Outcomes

Write the equation of a circle in standard form
Graph a circle

DEFINITION OF A CIRCLE

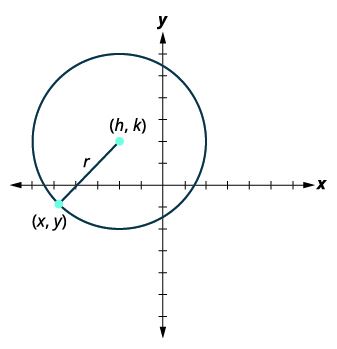
A circle is all points in a plane that are a fixed distance from a given point in the plane. The given point is called the center, $(h,k)$ , and the fixed distance is called the radius, $r$ , of the circle.

Picture of a Circle with center marked as (h,k) and radius r

DERIVING THE STANDARD FORM OF A CIRCLE

To derive the equation of a circle, we can use the distance formula with the points $(h,k)$ , $(x,y)$ , and the distance $r$ .

d = \sqrt{(x_{2} - x_{1})^{2} + (y_{2} - y_{1})^{2}}

$d=\sqrt{(x_{2}-x_{1})^{2}+(y_{2}-y_{1})^{2}}$

Substitute the values.

r = \sqrt{(x - h)^{2} + (y - k)^{2}}

$r=\sqrt{(x-h)^{2}+(y-k)^{2}}$

Square both sides.

r^{2} = (x - h)^{2} + (y - k)^{2}

$r^{2}=(x-h)^{2}+(y-k)^{2}$

STANDARD FORM OF A CIRCLE

The standard form of a circle is as follows:

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Write the Equation of a Circle in Standard Form

Example 1: WRITE THE STANDARD FORM Equation OF A CIRCLE

Write the standard form of a circle with radius $3$ and center $(0,0)$ .

Use the standard form of a circle.

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Substitute in the values $r=3, h=0, k=0$ .

${\left(x-0\right)}^{2}+{\left(y-0\right)}^{2}=3^{2}$

Simplify.

${x}^{2}+{y}^{2}=9$

Example 2: WRITE THE STANDARD FORM equation OF A CIRCLE

Write the standard form of a circle with radius $2$ and center $(-1,3)$ .

Show Solution

Use the standard form of a circle.

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Substitute in the values $r=2, h=-1, k=3$ .

${\left(x-(-1)\right)}^{2}+{\left(y-3\right)}^{2}=2^{2}$

Simplify.

${\left(x+1\right)}^{2}+{\left(y-3\right)}^{2}=4$

Example 3: finding the center and radius

Find the center and radius, then graph the circle: ${\left(x+2\right)}^{2}+{\left(y-1\right)}^{2}=9$ .

Use the standard form of a circle.

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Identify the center $(h,k)$ , and radius $r$ .

${\left(x-(-2)\right)}^{2}+{\left(y-1\right)}^{2}=3^{2}$

The center is $(-2,1)$ , and the radius is $3$ .

Now graph the circle. Plot the center first and then go up, down, left, and right 2 places.

Graph of a Circle with center (-2,1) and radius 3

Example 4: finding the center and radius

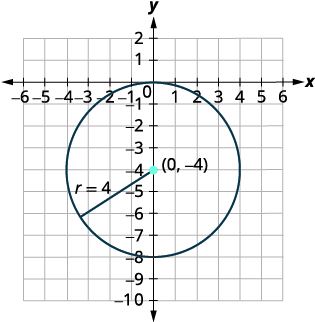
Find the center and radius, then graph the circle: ${4x}^{2}+{4y}^{2}=64$ .

Show Solution

Divide each side by 4.

${x}^{2}+{y}^{2}=16$

Use the standard form of a circle.

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Identify the center $(h,k)$ , and radius $r$ .

${\left(x-0\right)}^{2}+{\left(y-0\right)}^{2}=4^{2}$

The center is $(0,0)$ , and the radius is $4$ .

Now graph the circle. Plot the center first and then go up, down, left, and right 4 places.

Graph of a Circle with center (0,0) and radius 4

GENERAL FORM OF A CIRCLE

The general form of a circle is as follows:

${x}^{2}+{y}^{2}+ax+by+c=0$

Example 5: WRITE THE STANDARD FORM Equation OF A CIRCLE

Find the center and radius, then graph: ${x}^{2}+{y}^{2}-4x-6y+4=0$ .

We need to rewrite this general form into standard form in order to find the center and radius.

${x}^{2}+{y}^{2}-4x-6y+4=0$

Group the x-terms and y-terms. Collect the constants on the right right side.

${x}^{2}-4x+{y}^{2}-6y=-4$

Complete the squares.

${x}^{2}-4x+4+{y}^{2}-6y+9=-4+4+9$

Rewrite as binomial squares.

${\left(x-2\right)}^{2}+{\left(y-3\right)}^{2}=9$

The center is $(2,3)$ , and the radius is $3$ .

Now graph the circle. Plot the center first and then go up, down, left, and right 3 places.

Graph of a Circle with center centered at (2,3) and radius 3

Example 6: WRITE THE STANDARD FORM Equation OF A CIRCLE

Find the center and radius, then graph: ${x}^{2}+{y}^{2}+8y=0$ .

Show Solution

We need to rewrite this general form into standard form in order to find the center and radius.

${x}^{2}+{y}^{2}+8y=0$

Group the x-terms and y-terms.

${x}^{2}+{y}^{2}+8y=0$

Complete the squares.

${x}^{2}+{y}^{2}+8y+16=0+16$

Rewrite as binomial squares.

${\left(x-0\right)}^{2}+{\left(y+4\right)}^{2}=16$

The center is $(0,-4)$ , and the radius is $4$ .

Now graph the circle. Plot the center first and then go up, down, left, and right 4 places.

Graph of a Circle with center centered at (0,-4) and radius 4

Example 7: APPLYING THE DISTANCE AND MIDPOINT FORMULAS TO A CIRCLE EQUATION

The diameter of a circle has endpoints $(-1,-4)$ and $(7,2)$ . Find the center and radius of the circle and also write its standard form equation.

The center of a circle is the center, or midpoint, of its diameter. Thus the midpoint formula will yield the center point.

$M=\left(\frac{{x}_{1}+{x}_{2}}{2},\frac{{y}_{1}+{y}_{2}}{2}\right)$

$=\left(\frac{-1+7}{2},\frac{-4+2}{2}\right)$

$=\left(\frac{6}{2},\frac{-2}{2}\right)$

$=(3,-1)$

The center is $(3,-1)$ . The distance formula will be used to find the distance from the center to one of the points on the circle. This will yield the radius:

$d=\sqrt{(x_{2}-x_{1})^{2}+(y_{2}-y_{1})^{2}}$

$d=\sqrt{(7-3)^{2}+(2-(-1))^{2}}$

$d=\sqrt{4^{2}+3^{2}}=5$

The distance from the center to a point on the circle is 5. Therefore the radius is 5. The center and radius can now be used to find the standard form of the circle:

Start with the standard form of a circle.

${\left(x-h\right)}^{2}+{\left(y-k\right)}^{2}=r^{2}$

Substitute in the values $r=5, h=3, k=-1$ .

${\left(x-3\right)}^{2}+{\left(y-(-1)\right)}^{2}=5^{2}$

Simplify.

${\left(x-3\right)}^{2}+{\left(y+1\right)}^{2}=25$

Example 8: Finding the Center of a Circle

The diameter of a circle has endpoints $\left(-1,-4\right)$ and $\left(5,-4\right)$ . Find the center of the circle.

Show Solution

The center of a circle is the center or midpoint of its diameter. Thus, the midpoint formula will yield the center point.

\begin{array}{l} (\frac{x_{1} + x_{2}}{2}, \frac{y_{1} + y_{2}}{2}) \\ (\frac{- 1 + 5}{2}, \frac{- 4 - 4}{2}) = (\frac{4}{2}, - \frac{8}{2}) = (2, - 4) \end{array}

$\begin{array}{l}\left(\frac{{x}_{1}+{x}_{2}}{2},\frac{{y}_{1}+{y}_{2}}{2}\right)\\ \left(\frac{-1+5}{2},\frac{-4 - 4}{2}\right)=\left(\frac{4}{2},-\frac{8}{2}\right)=\left(2,-4\right)\end{array}$

Key Concepts

A circle is all points in a plane that are a fixed distance from a given point on the plane. The given point is called the center, and the fixed distance is called the radius.
The standard form of the equation of a circle with center $(h,k)$ and radius $r$ is ${\left(x-h\right)}^{2}{+}{\left(y-k\right)}^{2}=r^{2}$

Section 1.2 Homework Exercises

For the following exercises, write the standard form of the equation of the circle with the given radius and center $(0,0)$ .

1. Radius: $7$

2. Radius: $9$

3. Radius: $\sqrt{2}$

4. Radius: $\sqrt{5}$

In the following exercises, write the standard form of the equation of the circle with the given radius and center.

5. Radius: $1$ , center: $(3,5)$

6. Radius: $10$ , center: $(-2,6)$

7. Radius: $2.5$ , center: $(1.5,-3.5)$

8. Radius: $1.5$ , center: $(-5.5,-6.5)$

For the following exercises, write the standard form of the equation of the circle with the given center and point on the circle.

9. Center: $(3,-2)$ with point $(3,6)$

10. Center: $(6,-6)$ with point $(2,-3)$

11. Center: $(4,4)$ with point $(2,2)$

12. Center: $(-5,6)$ with point $(-2,3)$

In the following exercises, find the center and radius and then graph each circle.

13. ${\left(x+5\right)}^{2}+{\left(y+3\right)}^{2}=1$

14. ${\left(x-2\right)}^{2}+{\left(y-3\right)}^{2}=9$

15. ${\left(x-4\right)}^{2}+{\left(y+2\right)}^{2}=16$

16. ${\left(x+2\right)}^{2}+{\left(y-5\right)}^{2}=4$

17. $x^{2}+{\left(y+2\right)}^{2}=25$

18. ${\left(x-1\right)}^{2}+{y}^{2}=36$

19. ${\left(x-1.5\right)}^{2}+{\left(y-2.5\right)}^{2}=0.25$

20. ${\left(x-1\right)}^{2}+{\left(y-3\right)}^{2}=\frac{9}{4}$

21. $x^{2}+y^{2}=64$

22. $x^{2}+y^{2}=49$

23. $2x^{2}+2y^{2}=8$

24. $6x^{2}+6y^{2}=216$

In the following exercises, identify the center and radius and graph.

25. $x^{2}+y^{2}+2x+6y+9=0$

26. $x^{2}+y^{2}-6x-8y=0$

27. $x^{2}+y^{2}-4x+10y-7=0$

28. $x^{2}+y^{2}+12x-14y+21=0$

29. $x^{2}+y^{2}+6y+5=0$

30. $x^{2}+y^{2}-10y=0$

31. $x^{2}+y^{2}+4x=0$

32. $x^{2}+y^{2}-14x+13=0$

33. Explain the relationship between the distance formula and the equation of a circle.

34. In your own words, state the definition of a circle.

35. In your own words, explain the steps you would take to change the general form of the equation of a circle to the standard form.

Chapter 1: Graphs

Learning Outcomes

DEFINITION OF A CIRCLE

DERIVING THE STANDARD FORM OF A CIRCLE

STANDARD FORM OF A CIRCLE

Write the Equation of a Circle in Standard Form

Example 1: WRITE THE STANDARD FORM Equation OF A CIRCLE

Example 2: WRITE THE STANDARD FORM equation OF A CIRCLE

Example 3: finding the center and radius

Example 4: finding the center and radius

GENERAL FORM OF A CIRCLE

Example 5: WRITE THE STANDARD FORM Equation OF A CIRCLE

Example 6: WRITE THE STANDARD FORM Equation OF A CIRCLE

Example 7: APPLYING THE DISTANCE AND MIDPOINT FORMULAS TO A CIRCLE EQUATION

Example 8: Finding the Center of a Circle

Key Concepts

Section 1.2 Homework Exercises