The Graph of a Linear Function

Learning Outcome

  • Graph linear functions using tables

A helpful first step in graphing a function is to make a table of values. This is particularly useful when you do not know the general shape the function will have. You probably already know that a linear function will be a straight line, but let us make a table first to see how it can be helpful.

When making a table, it is a good idea to include negative values, positive values, and zero to ensure that you do have a linear function.

Make a table of values for [latex]f(x)=3x+2[/latex].

Make a two-column table. Label the columns x and f(x).

x f(x)

Choose several values for x and put them as separate rows in the x column. These are YOUR CHOICE – there is no “right” or “wrong” values to pick, just go for it.

Tip: It is always good to include 0, positive values, and negative values, if possible.

x f(x)
[latex]−2[/latex]
[latex]−1[/latex]
[latex]0[/latex]
[latex]1[/latex]
[latex]3[/latex]

Evaluate the function for each value of x, and write the result in the f(x) column next to the x value you used.

When [latex]x=0[/latex], [latex]f(0)=3(0)+2=2[/latex],

[latex]f(1)=3(1)+2=5[/latex],

[latex]f(−1)=3(−1)+2=−3+2=−1[/latex], and so on.

x f(x)
[latex]−2[/latex] [latex]−4[/latex]
[latex]−1[/latex] [latex]−1[/latex]
[latex]0[/latex] [latex]2[/latex]
[latex]1[/latex] [latex]5[/latex]
[latex]3[/latex] [latex]11[/latex]

(Note that your table of values may be different from someone else’s. You may each choose different numbers for x.)

Now that you have a table of values, you can use them to help you draw both the shape and location of the function. Important: The graph of the function will show all possible values of x and the corresponding values of y. This is why the graph is a line and not just the dots that make up the points in our table.

Now graph [latex]f(x)=3x+2[/latex].
Using the table of values we created above, you can think of f(x) as y. Each row forms an ordered pair that you can plot on a coordinate grid.

x f(x)
[latex]−2[/latex] [latex]−4[/latex]
[latex]−1[/latex] [latex]−1[/latex]
[latex]0[/latex] [latex]2[/latex]
[latex]1[/latex] [latex]5[/latex]
[latex]3[/latex] [latex]11[/latex]

Plot the points.
The points negative 2, negative 4; the point negative 1, negative 1; the point 0, 2; the point 1, 5; the point 3, 11.

Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.

A line through the points in the previous graph.

 

Let us try another one. Before you look at the answer, try to make the table yourself and draw the graph on a piece of paper.

Example

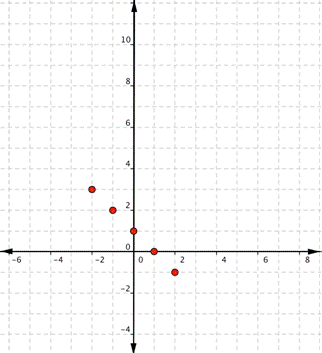
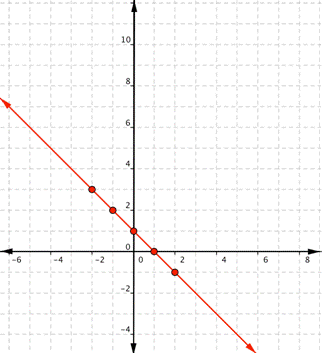
Graph [latex]f(x)=−x+1[/latex].

The following video shows another example of how to graph a linear function on a set of coordinate axes.