Solution Sets of Inequalities

Learning Outcome

Identify whether an ordered pair is in the solution set of a linear inequality

The graph below shows the region of values that makes the inequality $3x+2y\leq6$ true (shaded red), the boundary line $3x+2y=6$ , as well as a handful of ordered pairs. The boundary line is solid because points on the boundary line $3x+2y=6$ will make the inequality $3x+2y\leq6$ true.

A solid downward-sloping line running. The region below the line is shaded and is labeled 3x+2y is less than or equal to 6. The region above the line is unshaded and is labeled 3x+2y=6. The points (-5,5) and (-2,-2) are in the shaded region. The points (2,3) and (4,-1) are in the unshaded region. The point (2,0) is on the line.

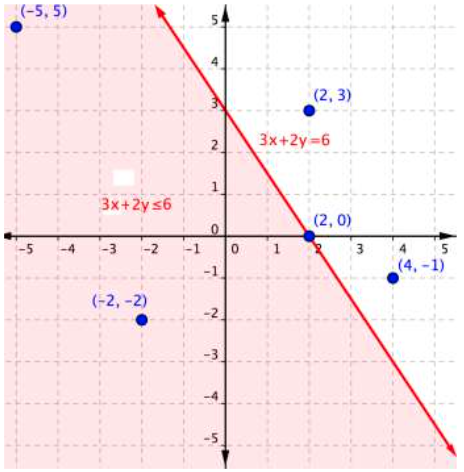
You can substitute the x and y-values of each of the $(x,y)$ ordered pairs into the inequality to find solutions. Sometimes making a table of values makes sense for more complicated inequalities.

Ordered Pair	Makes the inequality $3x+2y\leq6$ a true statement	Makes the inequality $3x+2y\leq6$ a false statement
$(−5, 5)$	$\begin{array}{r}3\left(−5\right)+2\left(5\right)\leq6\\−15+10\leq6\\−5\leq6\end{array}$
$(−2,−2)$	$\begin{array}{r}3\left(−2\right)+2\left(–2\right)\leq6\\−6+\left(−4\right)\leq6\\–10\leq6\end{array}$
$(2,3)$		$\begin{array}{r}3\left(2\right)+2\left(3\right)\leq6\\6+6\leq6\\12\leq6\end{array}$
$(2,0)$	$\begin{array}{r}3\left(2\right)+2\left(0\right)\leq6\\6+0\leq6\\6\leq6\end{array}$
$(4,−1)$		$\begin{array}{r}3\left(4\right)+2\left(−1\right)\leq6\\12+\left(−2\right)\leq6\\10\leq6\end{array}$

If substituting $(x,y)$ into the inequality yields a true statement, then the ordered pair is a solution to the inequality, and the point will be plotted within the shaded region or the point will be part of a solid boundary line. A false statement means that the ordered pair is not a solution, and the point will graph outside the shaded region, or the point will be part of a dotted boundary line.

Example

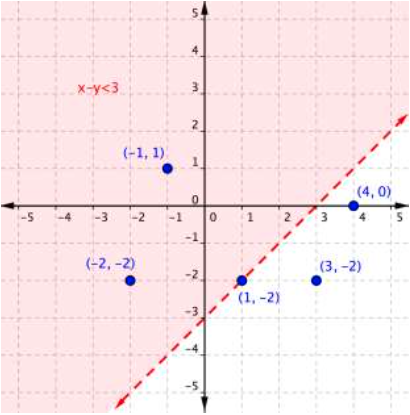
Use the graph to determine which ordered pairs plotted below are solutions of the inequality $x–y<3$ . Upward-sloping dotted line. The region above the line is shaded and labeled x-y<3. The points (4,0) and (3,-2) are in the unshaded region. The point (1,-2) is on the dotted line. The points (-1,1) and (-2,-2) are in the shaded region.

Show Solution

Solutions will be located in the shaded region. Since this is a “less than” problem, ordered pairs on the boundary line are not included in the solution set.

The values below are located in the shaded region so they are solutions. When substituted into the inequality $x–y<3$ , they produce true statements.

$(−1,1)$

$(−2,−2)$

These values are not located in the shaded region, so are not solutions. When substituted into the inequality $x-y<3$ , they produce false statements.

$(1,−2)$

$(3,−2)$

$(4,0)$

$(−1,1)$ and $(−2,−2)$ are the ordered pairs indicated in the graph that are solutions to the inequality.

The following video shows an example of determining whether an ordered pair is a solution to an inequality.

Example

Is $(2,−3)$ a solution of the inequality $y<−3x+1$ ?

Show Solution

If $(2,−3)$ is a solution, then it will yield a true statement when substituted into the inequality $y<−3x+1$ .

$y<−3x+1$

Substitute $x=2$ and $y=−3$ into inequality.

$−3<−3\left(2\right)+1$

Evaluate.

$\begin{array}{l}−3<−6+1\\−3<−5\end{array}$

This statement is not true, so the ordered pair $(2,−3)$ is not a solution.

The following video shows another example of determining whether an ordered pair is a solution to an inequality.

Course Enrichment Unit