Transformations of exponential graphs behave similarly to those of other functions. Just as with other parent functions, we can apply the four types of transformations—shifts, reflections, stretches, and compressions—to the parent function [latex]f\left(x\right)={b}^{x}[/latex] without loss of shape. For instance, just as the quadratic function maintains its parabolic shape when shifted, reflected, stretched, or compressed, the exponential function also maintains its general shape regardless of the transformations applied.

Graphing a Vertical Shift

The first transformation occurs when we add a constant d to the parent function [latex]f\left(x\right)={b}^{x}[/latex] giving us a vertical shift d units in the same direction as the sign. For example, if we begin by graphing a parent function, [latex]f\left(x\right)={2}^{x}[/latex], we can then graph two vertical shifts alongside it using [latex]d=3[/latex]: the upward shift, [latex]g\left(x\right)={2}^{x}+3[/latex] and the downward shift, [latex]h\left(x\right)={2}^{x}-3[/latex]. Both vertical shifts are shown in the figure below.

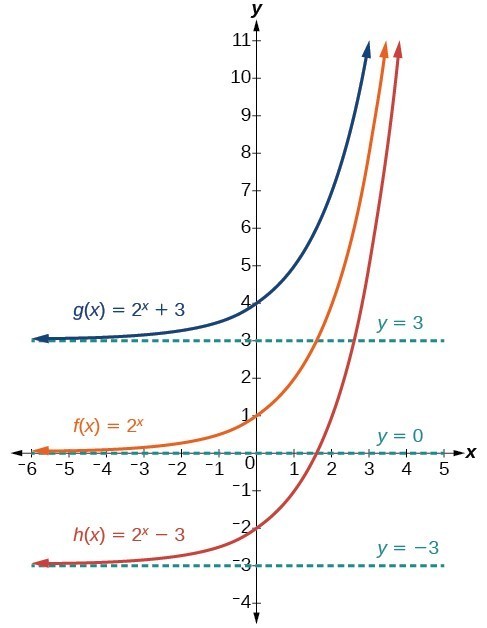
Observe the results of shifting [latex]f\left(x\right)={2}^{x}[/latex] vertically:

• The domain [latex]\left(-\infty ,\infty \right)[/latex] remains unchanged.
• When the function is shifted up 3 units giving [latex]g\left(x\right)={2}^{x}+3[/latex]:
  • The y-intercept shifts up 3 units to [latex]\left(0,4\right)[/latex].
  • The asymptote shifts up 3 units to [latex]y=3[/latex].
  • The range becomes [latex]\left(3,\infty \right)[/latex].
• When the function is shifted down 3 units giving [latex]h\left(x\right)={2}^{x}-3[/latex]:
  • The y-intercept shifts down 3 units to [latex]\left(0,-2\right)[/latex].
  • The asymptote also shifts down 3 units to [latex]y=-3[/latex].
  • The range becomes [latex]\left(-3,\infty \right)[/latex].

Graphing a Horizontal Shift

The next transformation occurs when we add a constant c to the input of the parent function [latex]f\left(x\right)={b}^{x}[/latex] giving us a horizontal shift c units in the opposite direction of the sign. For example, if we begin by graphing the parent function [latex]f\left(x\right)={2}^{x}[/latex], we can then graph two horizontal shifts alongside it using [latex]c=3[/latex]: the shift left, [latex]g\left(x\right)={2}^{x+3}[/latex], and the shift right, [latex]h\left(x\right)={2}^{x - 3}[/latex]. Both horizontal shifts are shown in the graph below.

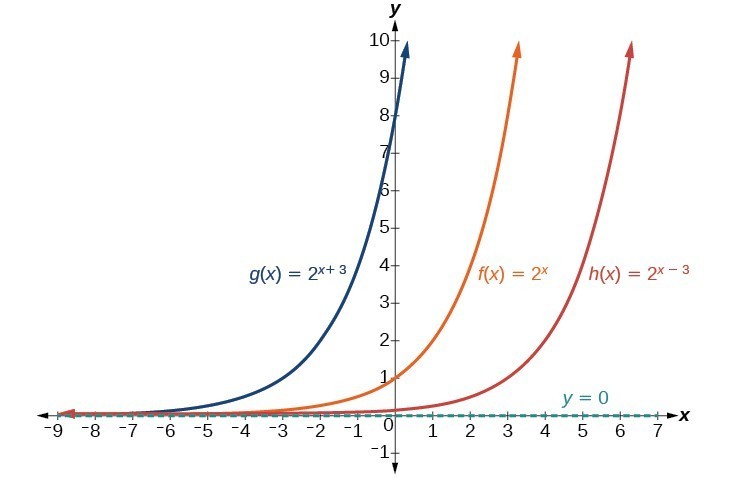
Observe the results of shifting [latex]f\left(x\right)={2}^{x}[/latex] horizontally:

• The domain, [latex]\left(-\infty ,\infty \right)[/latex], remains unchanged.
• The asymptote, [latex]y=0[/latex], remains unchanged.
• The y-intercept shifts such that:
  • When the function is shifted left 3 units to [latex]g\left(x\right)={2}^{x+3}[/latex], the y-intercept becomes [latex]\left(0,8\right)[/latex]. This is because [latex]{2}^{x+3}=\left({2}^{3}\right){2}^{x}=\left(8\right){2}^{x}[/latex], so the initial value of the function is 8.
  • When the function is shifted right 3 units to [latex]h\left(x\right)={2}^{x - 3}[/latex], the y-intercept becomes [latex]\left(0,\frac{1}{8}\right)[/latex]. Again, see that [latex]{2}^{x-3}=\left({2}^{-3}\right){2}^{x}=\left(\frac{1}{8}\right){2}^{x}[/latex], so the initial value of the function is [latex]\frac{1}{8}[/latex].

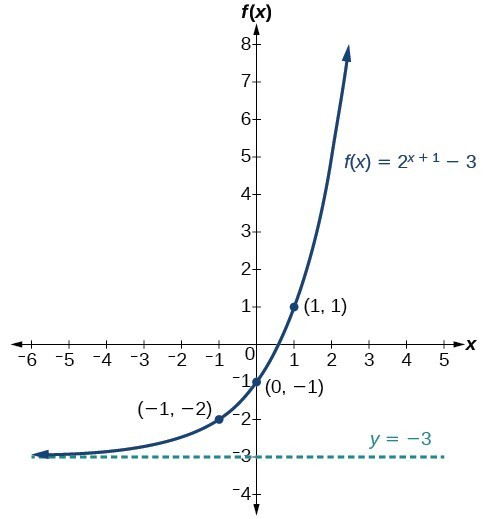
Example: Graphing a Shift of an Exponential Function

Graph [latex]f\left(x\right)={2}^{x+1}-3[/latex]. State the domain, range, and asymptote.

Show Solution

We have an exponential equation of the form [latex]f\left(x\right)={b}^{x+c}+d[/latex], with [latex]b=2[/latex], [latex]c=1[/latex], and [latex]d=-3[/latex].

Draw the horizontal asymptote [latex]y=d[/latex], so draw [latex]y=-3[/latex].

Identify the shift; it is [latex]\left(-1,-3\right)[/latex].

The domain is [latex]\left(-\infty ,\infty \right)[/latex], the range is [latex]\left(-3,\infty \right)[/latex], and the horizontal asymptote is [latex]y=-3[/latex].

Shift the graph of [latex]f\left(x\right)={b}^{x}[/latex] left 1 unit and down 3 units.
The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(-3,\infty \right)[/latex]; the horizontal asymptote is [latex]y=-3[/latex].

Watch the following video for more examples of the difference between horizontal and vertical shifts of exponential functions and the resulting graphs and equations.

Using a Graph to Approximate a Solution to an Exponential Equation

As we discussed in the previous section, graphing can help you confirm or find the solution to an exponential equation. An exponential equation is different from a function because a function is a large collection of points made of inputs and corresponding outputs, whereas equations that you have seen typically have one, two, or no solutions.  For example, [latex]f(x)=2^{x}[/latex] is a function and is comprised of many points [latex](x,f(x))[/latex]. However, [latex]4=2^{x}[/latex] can be solved to find the specific value for x that makes it a true statement. The graph below shows the intersection of the line [latex]f(x)=4[/latex] and [latex]f(x)=2^{x}[/latex], which is [latex](2, 4)[/latex]. You can see they cross at [latex]y=4[/latex].

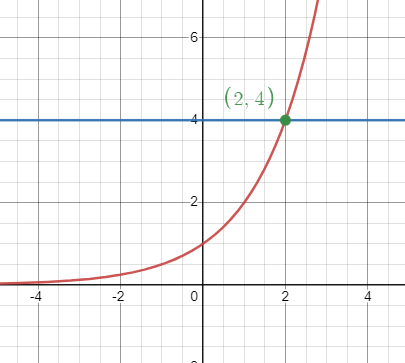
In the following example you can try this yourself.

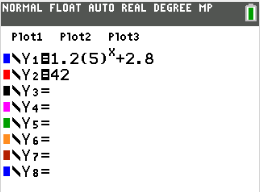
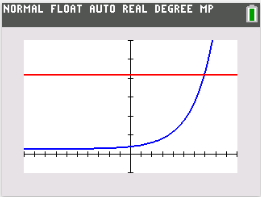
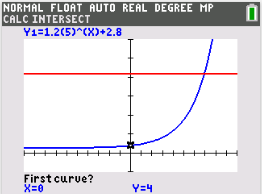
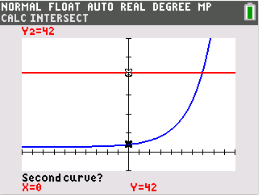
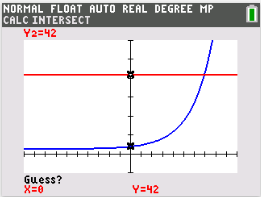
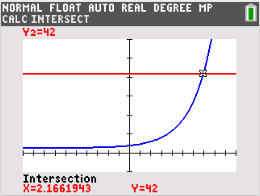
Example : Approximating the Solution of an Exponential Equation

Use an online graphing calculator to solve [latex]42=1.2{\left(5\right)}^{x}+2.8[/latex] graphically.

Show Solution

[Case 1] Using online graphing calculators

First, graph the function [latex]f(x)=1.2{\left(5\right)}^{x}+2.8[/latex] and graph [latex]f(x) = 42[/latex].

Online graphing calculators automatically calculate points of interest including intersections. Essentially, you are looking for the intersection of two functions. Click on the point of intersection, and you will see the x and y values for the point.

[Case 2] Using TI-84 CE

First, graph the function [latex]f(x)=1.2{\left(5\right)}^{x}+2.8[/latex] and graph [latex]f(x) = 42[/latex] by typing in [latex]Y1=1.2{\left(5\right)}^{x}+2.8[/latex] and [latex]Y2= 42[/latex], respectively.

Second, click “calc (2nd+trace)” and then “5: intersect.” Now TI-84 will ask you the “first curve” and “second curve.” Since TI-84 chooses the first and second curve automatically, just click “enter.”

Finally, we can find the solution [latex]x\approx. 2.166[/latex], which is the x value of the intersecting point.