Learning Outcomes
By the end of this section, you will be able to:
- Solve compound inequalities involving “or,” and express solutions both graphically and with interval notation
- Solve compound inequalities involving “and,” and express solutions both graphically and with interval notation
Many times we encounter situations with multiple requirements. Sometimes we need only satisfy at least one requirement on a list, and other times we need to satisfy all the requirements on a list. For example, one class may require that you purchase a physical textbook or an e-text. If you have one of these, or both, you have satisfied the requirement. On the other hand, if your class requires you to have a textbook and a calculator, you must have both of these items to satisfy the requirement.
A compound inequality consists of two inequalities joined with the word and or the word or.
Solve Compound Inequalities in the Form of “or”
A compound inequality consisting of two inequalities joined with the word or has a solution set made up of all real numbers which satisfy the first inequality, the second inequality, or both. Unions allow us to create a new set from two that may or may not have elements in common.
Some inequalities need to be simplified before their solution can be written or graphed. In the following example, you will see an example of how to solve a one-step inequality in the or form. Note how each inequality is treated independently until the end, where the solution is described in terms of both inequalities. You will use the same properties to solve compound inequalities that you used to solve regular inequalities.
Example
Solve for [latex]x[/latex].
[latex]3x–1<8[/latex] or [latex]x–5>0[/latex]
Remember to apply the properties of inequalities when you are solving compound inequalities. The next example involves dividing by a negative to isolate a variable.
Example
Solve for [latex]y[/latex].
[latex]2y+7\lt13[/latex] or [latex]−3y–2\lt10[/latex]
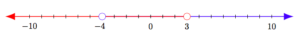
In the last example, the final answer included solutions whose intervals overlapped. This caused the answer to include all numbers on the number line. In words, we call this solution “all real numbers”. Any real number will produce a true statement for either [latex]y<3\text{ or }y\gt -4[/latex] when it is substituted for y.
Example
Solve for [latex]z[/latex].
[latex]5z–3\gt−18[/latex] or [latex]−2z–1\gt15[/latex]
The following video contains an example of solving a compound inequality involving or and drawing the associated graph.
Try It
Solve Compound Inequalities in the Form of “and”
The solution of a compound inequality that consists of two inequalities joined with the word and is the intersection of the solutions of each inequality. In other words, both statements must be true at the same time. The solution to an and compound inequality are all the solutions that the two inequalities have in common. As we saw in the last sections, this is where the two graphs overlap.
In this section, we will see more examples where we have to simplify the compound inequalities before we can express their solutions graphically or with an interval.
Example
Solve for x.
[latex]\displaystyle 1-4x\le 21\,\,\,\,\text{and}\,\,\,\,5x+2\ge22[/latex]
EXample
Solve for x:
[latex]\displaystyle {5}{x}-{2}\le{3}\text{ and }{4}{x}{+7}>{3}[/latex]
Try It
Compound inequalities in the form [latex]a
Rather than splitting a compound inequality in the form of [latex]a and [latex]x>a[/latex], you can more quickly to solve the inequality by applying the properties of inequality to all three segments of the compound inequality.
Example
Solve for x.
[latex]3\lt2x+3\leq 7[/latex]
Show Solution
In the video below, you will see another example of how to solve an inequality in the form [latex]a
To solve inequalities like [latex]ax. Whatever operation you perform on the middle portion of the inequality, you must also perform to each of the outside sections as well. Pay particular attention to division or multiplication by a negative.
The solution to a compound inequality with and is always the overlap between the solution to each inequality. There are three possible outcomes for compound inequalities joined by the word and:
Case 1:
Description
The solution could be all the values between two endpoints
Inequalities
[latex]x\le{1}[/latex] and [latex]x\gt{-1}[/latex], or as a bounded inequality: [latex]{-1}\lt{x}\le{1}[/latex]
Interval
[latex]\left(-1,1\right][/latex]
Graphs


Case 2:
Description
The solution could begin at a point on the number line and extend in one direction.
Inequalities
[latex]x\gt3[/latex] and [latex]x\ge4[/latex]
Interval
[latex]\left[4,\infty\right)[/latex]
Graphs


Case 3:
Description
In cases where there is no overlap between the two inequalities, there is no solution to the compound inequality
Inequalities
[latex]x\lt{-3}[/latex] and [latex]x\gt{3}[/latex]
Intervals
[latex]\left(-\infty,-3\right)[/latex] and [latex]\left(3,\infty\right)[/latex]
Graph

In the example below, there is no solution to the compound inequality because there is no overlap between the inequalities.
Example
Solve for x. [latex]x+2>5[/latex] and [latex]x+4<5[/latex]
Show Solution
Candela Citations
CC licensed content, Original- Revision and Adaptation. Provided by: Lumen Learning. License: CC BY: Attribution
CC licensed content, Shared previously- Ex 1: Solve a Compound Inequality Involving AND (Intersection). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. Located at: https://youtu.be/UU_KJI59_08. License: CC BY: Attribution
- Unit 10: Solving Equations and Inequalities, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology. Located at: http://nrocnetwork.org/resources/downloads/nroc-math-open-textbook-units-1-12-pdf-and-word-formats/. License: CC BY: Attribution
- Question ID 3921, 3920. Authored by: Lippman, D. License: CC BY: Attribution. License Terms: IMathAS Community License, CC-BY + GPL
Rather than splitting a compound inequality in the form of [latex]a
Example
Solve for x.
[latex]3\lt2x+3\leq 7[/latex]
In the video below, you will see another example of how to solve an inequality in the form [latex]a
To solve inequalities like [latex]a
The solution to a compound inequality with and is always the overlap between the solution to each inequality. There are three possible outcomes for compound inequalities joined by the word and:
| Case 1: | |
|---|---|
| Description | The solution could be all the values between two endpoints |
| Inequalities | [latex]x\le{1}[/latex] and [latex]x\gt{-1}[/latex], or as a bounded inequality: [latex]{-1}\lt{x}\le{1}[/latex] |
| Interval | [latex]\left(-1,1\right][/latex] |
| Graphs | 
|
| Case 2: | |
| Description | The solution could begin at a point on the number line and extend in one direction. |
| Inequalities | [latex]x\gt3[/latex] and [latex]x\ge4[/latex] |
| Interval | [latex]\left[4,\infty\right)[/latex] |
| Graphs | 
|
| Case 3: | |
| Description | In cases where there is no overlap between the two inequalities, there is no solution to the compound inequality |
| Inequalities | [latex]x\lt{-3}[/latex] and [latex]x\gt{3}[/latex] |
| Intervals | [latex]\left(-\infty,-3\right)[/latex] and [latex]\left(3,\infty\right)[/latex] |
| Graph |  |
In the example below, there is no solution to the compound inequality because there is no overlap between the inequalities.
Example
Solve for x. [latex]x+2>5[/latex] and [latex]x+4<5[/latex]
Candela Citations
- Revision and Adaptation. Provided by: Lumen Learning. License: CC BY: Attribution
- Ex 1: Solve a Compound Inequality Involving AND (Intersection). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. Located at: https://youtu.be/UU_KJI59_08. License: CC BY: Attribution
- Unit 10: Solving Equations and Inequalities, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology. Located at: http://nrocnetwork.org/resources/downloads/nroc-math-open-textbook-units-1-12-pdf-and-word-formats/. License: CC BY: Attribution
- Question ID 3921, 3920. Authored by: Lippman, D. License: CC BY: Attribution. License Terms: IMathAS Community License, CC-BY + GPL