The area of the front of the doghouse described in the introduction was [latex]4{x}^{2}+\frac{1}{2}x[/latex] ft².

This is an example of a polynomial, which is a sum of or difference of terms, each consisting of a variable raised to a nonnegative integer power. A number multiplied by a variable raised to an exponent, such as [latex]384\pi [/latex], is known as a coefficient. Coefficients can be positive, negative, or zero, and can be whole numbers, decimals, or fractions. Each product [latex]{a}_{i}{x}^{i}[/latex], such as [latex]384\pi w[/latex], is a term of a polynomial. If a term does not contain a variable, it is called a constant.

A polynomial containing only one term, such as [latex]5{x}^{4}[/latex], is called a monomial. A polynomial containing two terms, such as [latex]2x - 9[/latex], is called a binomial. A polynomial containing three terms, such as [latex]-3{x}^{2}+8x - 7[/latex], is called a trinomial.

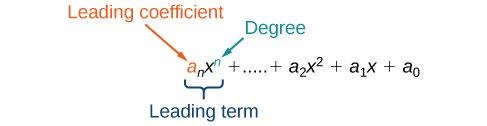
We can find the degree of a polynomial by identifying the highest power of the variable that occurs in the polynomial. The term with the highest degree is called the leading term because it is usually written first. The coefficient of the leading term is called the leading coefficient. When a polynomial is written so that the powers are descending, we say that it is in standard form.

In the following video example, we will identify the terms, leading coefficient, and degree of a polynomial.

Add and Subtract Polynomials

We can add and subtract polynomials by combining like terms, which are terms that contain the same variables raised to the same exponents. For example, [latex]5{x}^{2}[/latex] and [latex]-2{x}^{2}[/latex] are like terms, and can be added to get [latex]3{x}^{2}[/latex], but [latex]3x[/latex] and [latex]3{x}^{2}[/latex] are not like terms, and therefore cannot be added.

Watch this video to see more examples of adding and subtracting polynomials.

Multiply Polynomials

Multiplying polynomials is a bit more challenging than adding and subtracting polynomials. We must use the distributive property to multiply each term in the first polynomial by each term in the second polynomial. We then combine like terms. We can also use a shortcut called the FOIL method when multiplying binomials. Certain special products follow patterns that we can memorize and use instead of multiplying the polynomials by hand each time. We will look at a variety of ways to multiply polynomials.

Multiplying Polynomials Using the Distributive Property

To multiply a number by a polynomial, we use the distributive property. The number must be distributed to each term of the polynomial. We can distribute the [latex]2[/latex] in [latex]2\left(x+7\right)[/latex] to obtain the equivalent expression [latex]2x+14[/latex]. When multiplying polynomials, the distributive property allows us to multiply each term of the first polynomial by each term of the second. We then add the products together and combine like terms to simplify.

Watch this video to see more examples of how to use the distributive property to multiply polynomials.

Using FOIL to Multiply Binomials

A shortcut called FOIL is sometimes used to find the product of two binomials. It is called FOIL because we multiply the first terms, the outer terms, the inner terms, and then the last terms of each binomial.

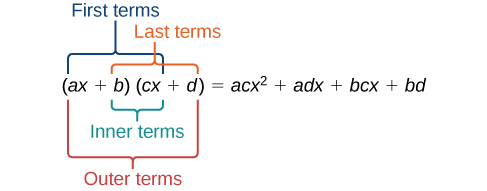
The FOIL method arises out of the distributive property. We are simply multiplying each term of the first binomial by each term of the second binomial, and then combining like terms.

Example: Using FOIL to Multiply Binomials

Use FOIL to find the product.

Solution

Find the product of the first terms.

Find the product of the outer terms.

Find the product of the inner terms.

Find the product of the last terms.

[latex]\begin{array}{cc}6{x}^{2}+6x - 54x - 54\hfill & \text{Add the products}.\hfill \\ 6{x}^{2}+\left(6x - 54x\right)-54\hfill & \text{Combine like terms}.\hfill \\ 6{x}^{2}-48x - 54\hfill & \text{Simplify}.\hfill \end{array}[/latex]