Learning Outcomes
- Construct and interpret Tree Diagrams
- Construct and interpret Venn Diagrams
Sometimes, when the probability problems are complex, it can be helpful to graph the situation. Tree diagrams and Venn diagrams are two tools that can be used to visualize and solve conditional probabilities.
Tree Diagrams
A tree diagram is a special type of graph used to determine the outcomes of an experiment. It consists of “branches” that are labeled with either frequencies or probabilities. Tree diagrams can make some probability problems easier to visualize and solve. The following example illustrates how to use a tree diagram.
Example
In an urn, there are [latex]11[/latex] balls. Three balls are red ([latex]R[/latex]) and eight balls are blue ([latex]B[/latex]). Draw two balls, one at a time, with replacement. “With replacement” means that you put the first ball back in the urn before you select the second ball. The tree diagram using frequencies that show all the possible outcomes follows.
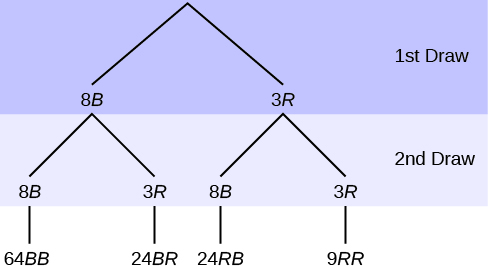
The first set of branches represents the first draw. The second set of branches represents the second draw. Each of the outcomes is distinct. In fact, we can list each red ball as [latex]R1[/latex], [latex]R2[/latex], and [latex]R3[/latex] and each blue ball as [latex]B1[/latex], [latex]B2[/latex], [latex]B3[/latex], [latex]B4[/latex], [latex]B5[/latex], [latex]B6[/latex], [latex]B7[/latex], and [latex]B8[/latex]. Then the nine [latex]RR[/latex] outcomes can be written as:
[latex]R1R1;\,\, R1R2;\,\, R1R3;\,\, R2R1;\,\, R2R2;\,\, R2R3;\,\, R3R1;\,\, R3R2;\,\, R3R3[/latex]
The other outcomes are similar.
There are a total of [latex]11[/latex] balls in the urn. Draw two balls, one at a time, with replacement. There are [latex]11(11) = 121[/latex] outcomes, the size of the sample space.
- List the [latex]24[/latex] [latex]BR[/latex] outcomes: [latex]B1R1[/latex], [latex]B1R2[/latex], [latex]B1R3[/latex], …
- Using the tree diagram, calculate [latex]P(RR)[/latex].
- Using the tree diagram, calculate [latex]P(RB \text{ OR } BR)[/latex].
- Using the tree diagram, calculate [latex]P(R \text{ on 1st draw AND } B \text { on 2nd draw })[/latex].
- Using the tree diagram, calculate [latex]P(R \text{ on 2nd draw GIVEN } B \text { on 1st draw })[/latex].
- Using the tree diagram, calculate [latex]P(BB)[/latex].
- Using the tree diagram, calculate [latex]P(B \text{ on the 2nd draw given } R \text { on the first draw })[/latex].
Example
An urn has three red marbles and eight blue marbles in it. Draw two marbles, one at a time, this time without replacement, from the urn. “Without replacement” means that you do not put the first ball back before you select the second marble. Following is a tree diagram for this situation. The branches are labeled with probabilities instead of frequencies. The numbers at the ends of the branches are calculated by multiplying the numbers on the two corresponding branches, for example, [latex](\frac{3}{11})(\frac{2}{10})=(\frac{6}{110})[/latex].
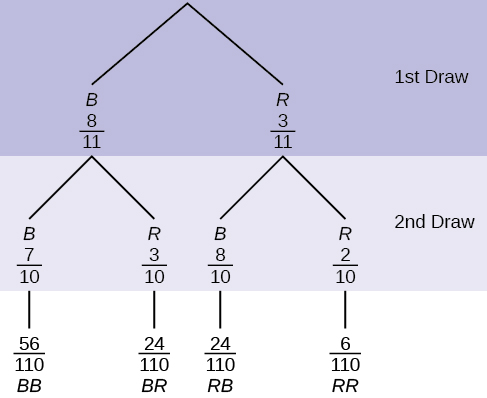
Total = [latex]\displaystyle\frac{{56+24+24+6}}{{110}}=\frac{{110}}{{110}}=1[/latex]
Note
If you draw a red on the first draw from the three red possibilities, there are two red marbles left to draw on the second draw. You do not put back or replace the first marble after you have drawn it. You draw without replacement, so that on the second draw there are ten marbles left in the urn.
Calculate the following probabilities using the tree diagram.
a. [latex]P(RR)[/latex] = ________
b. Fill in the blanks:
[latex]P(RB \text{ OR } BR = (\frac{3}{11})(\frac{8}{10}) + (\rule{1cm}{0.15mm})(\rule{1cm}{0.15mm}) = \frac{48}{110}[/latex]
c. [latex]P(R \text{ on 2nd|}B \text{ on 1st}) =[/latex]
d. Fill in the blanks.
[latex]P(R \text{ on 1st AND } B \text{ on 2nd }) = P(RB) = (\rule{1cm}{0.15mm})(\rule{1cm}{0.15mm})= \frac{24}{110}[/latex]
e. Find [latex]P(BB)[/latex].
f. Find [latex]P(B \text{ on 2nd|}R \text{ on 1st})[/latex].
a. [latex]P(RR) = (\frac{3}{11})(\frac{2}{10}) = \frac{6}{110}[/latex]
b. [latex]P(RB \text{ OR } BR = (\frac{3}{11})(\frac{8}{10}) + (\frac{8}{11})(\frac{3}{10}) = \frac{48}{110}[/latex]
c. [latex]P(R \text{ on 2nd|}B \text{ on 1st }) =\frac{3}{10}[/latex]
d. [latex]P(R \text{ on 1st AND } B \text{ on 2nd }) = P(RB) = (\frac{3}{11})(\frac{8}{10})= \frac{24}{110}[/latex]
e. [latex]P(BB) = (\frac{8}{11})(\frac{7}{10})[/latex]
f. Using the tree diagram, [latex]P(B \text{ on 2nd|}R \text{ on 1st }) = P(R|B) = (\frac{8}{10})[/latex]
If we are using probabilities, we can label the tree in the following general way.

- [latex]P(RR)[/latex] here means [latex]P(R \text{ on 2nd|}R \text{ on 1st})[/latex]
- [latex]P(BR)[/latex] here means [latex]P(B \text{ on 2nd|}R \text{ on 1st})[/latex]
- [latex]P(RB)[/latex] here means [latex]P(R \text{ on 2nd|}B \text{ on 1st})[/latex]
- [latex]P(BB)[/latex] here means [latex]P(B \text{ on 2nd|}B \text{ on 1st})[/latex]
Venn Diagram
A Venn diagram is a picture that represents the outcomes of an experiment. It generally consists of a box that represents the sample space S together with circles or ovals. The circles or ovals represent events.
Example
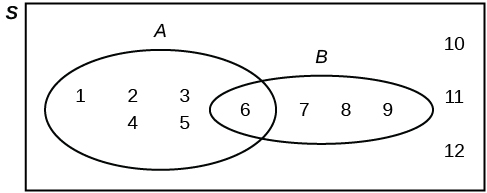
Suppose an experiment has the outcomes [latex]1, 2, 3, ... , 12[/latex] where each outcome has an equal chance of occurring. Let event [latex]A = \{1, 2, 3, 4, 5, 6\}[/latex] and event [latex]B = \{6, 7, 8, 9\}[/latex]. Then [latex]A \text{ AND } B = \{6\}[/latex] and [latex]A \text{ OR }B = \{1, 2, 3, 4, 5, 6, 7, 8, 9\}[/latex]. The Venn diagram is as follows:
Example
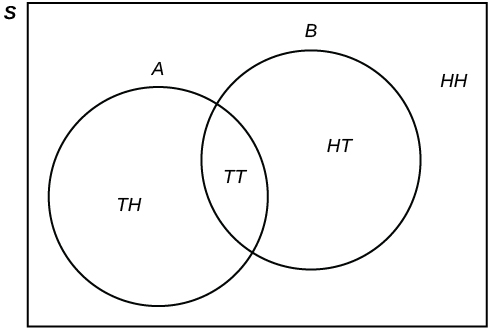
Flip two fair coins. Let [latex]A[/latex] = tails on the first coin. Let [latex]B[/latex] = tails on the second coin. Then [latex]A = \{TT, TH\}[/latex] and [latex]B = \{TT, HT\}[/latex]. Therefore,[latex]A \text{ AND } B = \{TT\}[/latex]. [latex]A \text{ OR } B = \{TH, TT, HT\}[/latex].
The sample space when you flip two fair coins is [latex]X = \{HH, HT, TH, TT\}[/latex]. The outcome [latex]HH[/latex] is in NEITHER [latex]A[/latex] NOR [latex]B[/latex]. The Venn diagram is as follows:
Glossary
- Tree Diagram
- the useful visual representation of a sample space and events in the form of a “tree” with branches marked by possible outcomes together with associated probabilities (frequencies, relative frequencies)
- Venn Diagram
- the visual representation of a sample space and events in the form of circles or ovals showing their intersections
B1R1 B1R2 B1R3 B2R1 B2R2 B2R3 B3R1 B3R2 B3R3 B4R1 B4R2 B4R3 B5R1 B5R2 B5R3 B6R1 B6R2 B6R3 B7R1B7R2 B7R3 B8R1 B8R2 B8R3
e. P(R on 2nd draw GIVEN B on 1st draw) = P(R on 2nd|B on 1st) = 2488 = 311
This problem is a conditional one. The sample space has been reduced to those outcomes that already have a blue on the first draw. There are 24 + 64 = 88 possible outcomes (24 BR and 64 BB). Twenty-four of the 88 possible outcomes are BR. 2488 = 311.
f. P(BB) = 64121
g. P(B on 2nd draw|R on 1st draw) = 811
There are 9 + 24 outcomes that have R on the first draw (9 RR and 24 RB). The sample space is then 9 + 24 = 33. 24 of the 33 outcomes have B on the second draw. The probability is then 2433.
Solutions to Try These 2:
Candela Citations
- Tree and Venn Diagrams. Provided by: OpenStax. Located at: http://cnx.org/contents/30189442-6998-4686-ac05-ed152b91b9de@17.44. License: Public Domain: No Known Copyright
- Introductory Statistics . Authored by: Barbara Illowski, Susan Dean. Provided by: Open Stax. Located at: http://cnx.org/contents/30189442-6998-4686-ac05-ed152b91b9de@17.44. License: CC BY: Attribution. License Terms: Download for free at http://cnx.org/contents/30189442-6998-4686-ac05-ed152b91b9de@17.44
- Count outcomes using tree diagram. Provided by: Khan Acadamy. Located at: https://www.khanacademy.org/math/cc-seventh-grade-math/cc-7th-probability-statistics/cc-7th-compound-events/v/tree-diagram-to-count-outcomes. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike
- Solving Problems with Venn Diagrams. Authored by: Mathispower4u. Located at: https://youtu.be/MassxXy8iko. License: All Rights Reserved. License Terms: Standard YouTube License
