Solutions

Solutions to Try Its

1. [latex]{f}^{-1}\left(f\left(x\right)\right)={f}^{-1}\left(\frac{x+5}{3}\right)=3\left(\frac{x+5}{3}\right)-5=\left(x - 5\right)+5=x[/latex] and [latex]f\left({f}^{-1}\left(x\right)\right)=f\left(3x - 5\right)=\frac{\left(3x - 5\right)+5}{3}=\frac{3x}{3}=x[/latex]

2. [latex]{f}^{-1}\left(x\right)={x}^{3}-4[/latex]

3. [latex]{f}^{-1}\left(x\right)=\sqrt{x - 1}[/latex]

4. [latex]{f}^{-1}\left(x\right)=\frac{{x}^{2}-3}{2},x\ge 0[/latex]

5. [latex]{f}^{-1}\left(x\right)=\frac{2x+3}{x - 1}[/latex]

Solutions to Odd-Numbered Exercises

1. It can be too difficult or impossible to solve for x in terms of y.

3. We will need a restriction on the domain of the answer.

5. [latex]{f}^{-1}\left(x\right)=\sqrt{x}+4[/latex]

7. [latex]{f}^{-1}\left(x\right)=\sqrt{x+3}-1[/latex]

9. [latex]{f}^{-1}\left(x\right)=-\sqrt{\frac{x - 5}{3}}[/latex]

11. [latex]f\left(x\right)=\sqrt{9-x}[/latex]

13. [latex]{f}^{-1}\left(x\right)=\sqrt[3]{x - 5}[/latex]

15. [latex]{f}^{-1}\left(x\right)=\sqrt[3]{4-x}[/latex]

17. [latex]{f}^{-1}\left(x\right)=\frac{{x}^{2}-1}{2},\left[0,\infty \right)[/latex]

19. [latex]{f}^{-1}\left(x\right)=\frac{{\left(x - 9\right)}^{2}+4}{4},\left[9,\infty \right)[/latex]

21. [latex]{f}^{-1}\left(x\right)={\left(\frac{x - 9}{2}\right)}^{3}[/latex]

23. [latex]{f}^{-1}\left(x\right)={\frac{2 - 8x}{x}}[/latex]

25. [latex]{f}^{-1}\left(x\right)=\frac{7x - 3}{1-x}[/latex]

27. [latex]{f}^{-1}\left(x\right)=\frac{5x - 4}{4x+3}[/latex]

29. [latex]{f}^{-1}\left(x\right)=\sqrt{x+1}-1[/latex]

31. [latex]{f}^{-1}\left(x\right)=\sqrt{x+6}+3[/latex]

33. [latex]{f}^{-1}\left(x\right)=\sqrt{4-x}[/latex]
Graph of f(x)=4- x^2 and its inverse, f^(-1)(x)= sqrt(4-x).

35. [latex]{f}^{-1}\left(x\right)=\sqrt{x}+4[/latex]
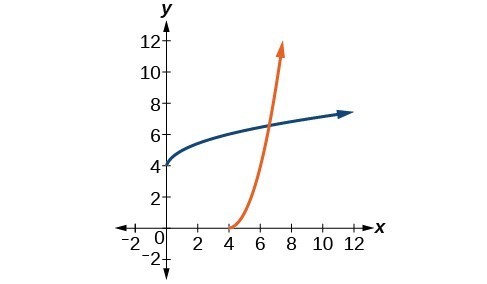
Graph of f(x)= (x-4)^2 and its inverse, f^(-1)(x)= sqrt(x)+4.

37. [latex]{f}^{-1}\left(x\right)=\sqrt[3]{1-x}[/latex]
Graph of f(x)= 1-x^3 and its inverse, f^(-1)(x)= (1-x)^(1/3).

39. [latex]{f}^{-1}\left(x\right)=\sqrt{x+8}+3[/latex]
Graph of f(x)= x^2-6x+1 and its inverse, f^(-1)(x)= sqrt(x+8)+3.

41. [latex]{f}^{-1}\left(x\right)=\sqrt{\frac{1}{x}}[/latex]
Graph of f(x)= 1/x^2 and its inverse, f^(-1)(x)= sqrt(1/x).

43. [latex]\left[-2,1\right)\cup \left[3,\infty \right)[/latex]
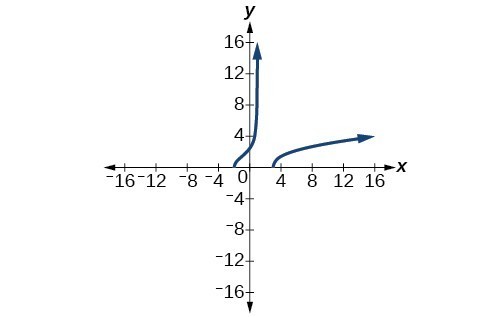
Graph of f(x)= sqrt((x+2)(x-3)/(x-1)).

45. [latex]\left[-4,2\right)\cup \left[5,\infty \right)[/latex]
Graph of f(x)= sqrt((x^2-x-20)/(x-2)).

47. [latex]\left(-2, 0\right); \left(4, 2\right); \left(22, 3\right)[/latex]
Graph of f(x)= x^3-x-2.

49. [latex]\left(-4, 0\right); \left(0, 1\right); \left(10, 2\right)[/latex]
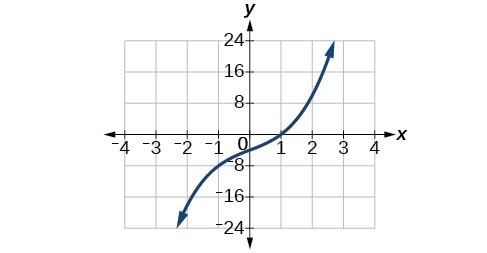
Graph of f(x)= x^3+3x-4.

51. [latex]\left(-3, -1\right); \left(1, 0\right); \left(7, 1\right)[/latex]
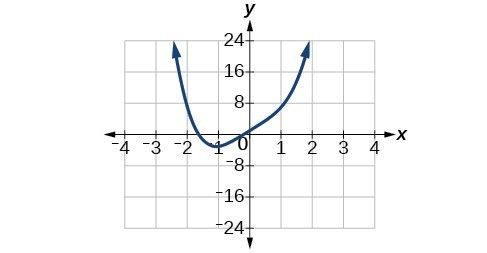
Graph of f(x)= x^4+5x+1.

53. [latex]{f}^{-1}\left(x\right)=\sqrt{x+\frac{{b}^{2}}{4}}-\frac{b}{2}[/latex]

55. [latex]{f}^{-1}\left(x\right)=\frac{{x}^{3}-b}{a}[/latex]

57. [latex]t\left(h\right)=\sqrt{\frac{200-h}{4.9}}[/latex], 5.53 seconds

59. [latex]r\left(V\right)=\sqrt[3]{\frac{3V}{4\pi }}[/latex], 3.63 feet

61. [latex]n\left(C\right)=\frac{100C - 25}{.6-C}[/latex], 250 mL

63. [latex]r\left(V\right)=\sqrt{\frac{V}{6\pi }}[/latex], 3.99 meters

65. [latex]r\left(V\right)=\sqrt{\frac{V}{4\pi }}[/latex], 1.99 inches