Learning Objectives
- Quadratic Functions
- Roots or zeros of a quadratic function
- Characteristics of a parabola
- vertex
- axis of symmetry
- x/y-intercepts
- Classifying solutions to quadratic equations
- The discriminant
Curved antennas, such as the ones shown in the photo, are commonly used to focus microwaves and radio waves to transmit television and telephone signals, as well as satellite and spacecraft communication. The cross-section of the antenna is in the shape of a parabola, which can be described by a quadratic function.

An array of satellite dishes. (credit: Matthew Colvin de Valle, Flickr)
Characteristics of Parabolas
The graph of a quadratic function is a U-shaped curve called a parabola. One important feature of the graph is that it has an extreme point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. In either case, the vertex is a turning point on the graph. The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry.
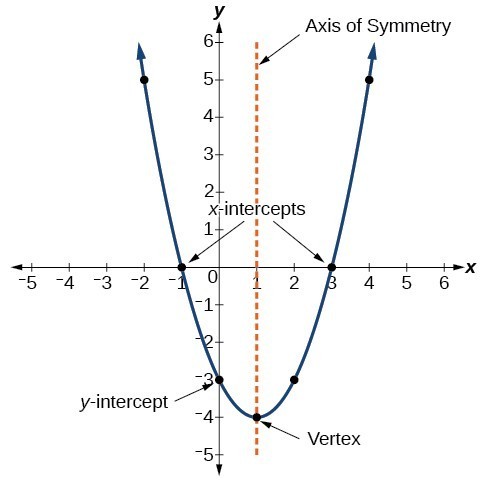
The y-intercept is the point at which the parabola crosses the y-axis. The x-intercepts are the points at which the parabola crosses the x-axis. If they exist, the x-intercepts represent the zeros, or roots, of the quadratic function, the values of x at which y = 0.
Example: Identifying the Characteristics of a Parabola
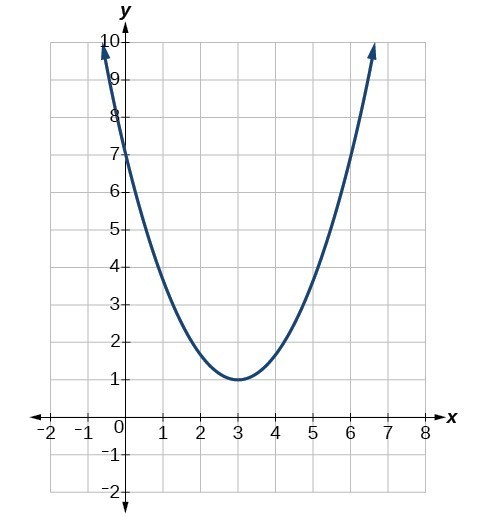
Determine the vertex, axis of symmetry, zeros, and y-intercept of the parabola shown below.
Try It
General and Standard Forms of Quadratic Functions
The general form of a quadratic function presents the function in the form
[latex]f\left(x\right)=a{x}^{2}+bx+c[/latex]
where a, b, and c are real numbers and [latex]a\ne 0[/latex]. If [latex]a>0[/latex], the parabola opens upward. If [latex]a<0[/latex], the parabola opens downward. We can use the general form of a parabola to find the equation for the axis of symmetry. The axis of symmetry is defined by [latex]x=-\frac{b}{2a}[/latex]. If we use the quadratic formula, [latex]x=\frac{-b\pm \sqrt{{b}^{2}-4ac}}{2a}[/latex], to solve [latex]a{x}^{2}+bx+c=0[/latex] for the x-intercepts, or zeros, we find the value of x halfway between them is always [latex]x=-\frac{b}{2a}[/latex], the equation for the axis of symmetry.
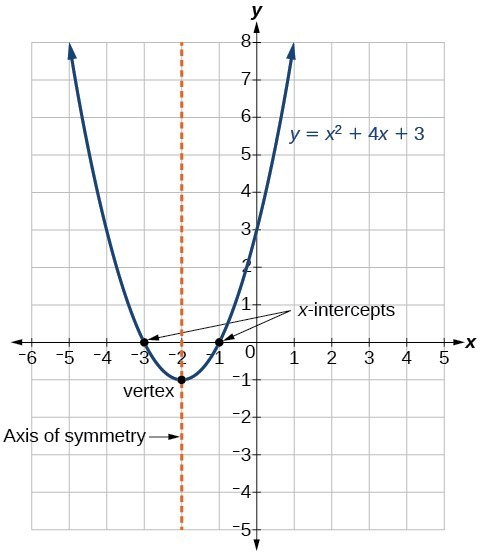
The figure below shows the graph of the quadratic function written in general form as [latex]y={x}^{2}+4x+3[/latex]. In this form, [latex]a=1,\text{ }b=4[/latex], and [latex]c=3[/latex]. Because [latex]a>0[/latex], the parabola opens upward. The axis of symmetry is [latex]x=-\frac{4}{2\left(1\right)}=-2[/latex]. This also makes sense because we can see from the graph that the vertical line [latex]x=-2[/latex] divides the graph in half. The vertex always occurs along the axis of symmetry. For a parabola that opens upward, the vertex occurs at the lowest point on the graph, in this instance, [latex]\left(-2,-1\right)[/latex]. The x-intercepts, those points where the parabola crosses the x-axis, occur at [latex]\left(-3,0\right)[/latex] and [latex]\left(-1,0\right)[/latex].
The standard form of a quadratic function presents the function in the form
[latex]f\left(x\right)=a{\left(x-h\right)}^{2}+k[/latex]
where [latex]\left(h,\text{ }k\right)[/latex] is the vertex. Because the vertex appears in the standard form of the quadratic function, this form is also known as the vertex form of a quadratic function.
Try It
Use the sliders for h and k to examine how changing the location of the vertex (h,k) of a parabola also changes the axis of symmetry, labeled as x = h. Notice that when you move k independently of h, you are only moving the vertical location of the vertex.
https://www.desmos.com/calculator/q3e3ymnpnn
The vertex of a parabola is either the maximum or minimum value of the parabola.If [latex]a>0[/latex], the parabola opens upward and the vertex is a minimum. If [latex]a<0[/latex], the parabola opens downward, and the vertex is a maximum. Use the textbox below to determine whether the vertex of the parabola in the interactive above is a minimum or a maximum.
Given a quadratic function in general form, find the vertex of the parabola.
One reason we may want to identify the vertex of the parabola is that this point will inform us where the maximum or minimum value of the output occurs, (k), and where it occurs, (x). If we are given the general form of a quadratic function:
[latex]f(x)=ax^2+bx+c[/latex]
We can define the vertex, [latex](h,k)[/latex], by doing the following:
- Identify a, b, and c.
- Find h, the x-coordinate of the vertex, by substituting a and b into [latex]h=-\frac{b}{2a}[/latex].
- Find k, the y-coordinate of the vertex, by evaluating [latex]k=f\left(h\right)=f\left(-\frac{b}{2a}\right)[/latex]
Example: Finding the Vertex of a Quadratic Function
Find the vertex of the quadratic function [latex]f\left(x\right)=2{x}^{2}-6x+7[/latex]. Rewrite the quadratic in standard form (vertex form).
Try It
Given the equation [latex]g\left(x\right)=13+{x}^{2}-6x[/latex], write the equation in general form and then in standard form.
In this section, we will investigate quadratic functions further, including solving problems involving area and projectile motion. Working with quadratic functions can be less complex than working with higher degree polynomial functions, so they provide a good opportunity for a detailed study of function behavior.
Classifying Solutions to Quadratic Equations
Much as we did in the application problems above, we also need to find intercepts of quadratic equations for graphing parabolas. Recall that we find the y-intercept of a quadratic by evaluating the function at an input of zero, and we find the x-intercepts at locations where the output is zero. Notice that the number of x-intercepts can vary depending upon the location of the graph.
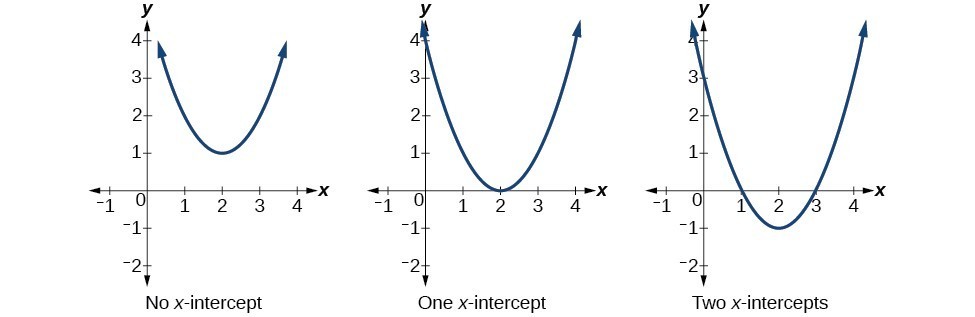
Number of x-intercepts of a parabola
Mathematicians also define x-intercepts as roots of the quadratic function.
How To: Given a quadratic function [latex]f\left(x\right)[/latex], find the y– and x-intercepts.
- Evaluate [latex]f\left(0\right)[/latex] to find the y-intercept.
- Solve the quadratic equation [latex]f\left(x\right)=0[/latex] to find the x-intercepts.
Example: Finding the y– and x-Intercepts of a Parabola
Find the y– and x-intercepts of the quadratic [latex]f\left(x\right)=3{x}^{2}+5x - 2[/latex].
In Example: Finding the y– and x-Intercepts of a Parabola, the quadratic was easily solved by factoring. However, there are many quadratics that cannot be factored. We can solve these quadratics by first rewriting them in standard form.
How To: Given a quadratic function, find the x-intercepts by rewriting in standard form.
- Substitute a and b into [latex]h=-\frac{b}{2a}[/latex].
- Substitute x = h into the general form of the quadratic function to find k.
- Rewrite the quadratic in standard form using h and k.
- Solve for when the output of the function will be zero to find the x-intercepts.
Example: Finding the Roots of a Parabola
Find the x-intercepts of the quadratic function [latex]f\left(x\right)=2{x}^{2}+4x - 4[/latex].
Try It
Find the y-intercept for the function [latex]g\left(x\right)=13+{x}^{2}-6x[/latex].
Complex Roots
Now you will hopefully begin to understand why we introduced complex numbers at the beginning of this module. Consider the following function: [latex]f(x)=x^2+2x+3[/latex], and it’s graph below:
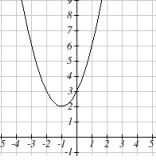
Does this function have roots? It’s probably obvious that this function does not cross the x-axis, therefore it doesn’t have any x-intercepts. Recall that the x-intercepts of a function are found by setting the function equal to zero:
[latex]x^2+2x+3=0[/latex]
In the next example, we will solve this equation. You will see that there are roots, but they are not x-intercepts because the function does not contain (x,y) pairs that are on the x-axis. We call these complex roots.
By setting the function equal to zero and using the quadratic formula to solve, you will see that the roots contain complex numbers:
[latex]x^2+2x+3=0[/latex]
Example
Find the x-intercepts of the quadratic function. [latex]f(x)=x^2+2x+3[/latex]
The Discriminant
The quadratic formula not only generates the solutions to a quadratic equation, it tells us about the nature of the solutions. When we consider the discriminant, or the expression under the radical, [latex]{b}^{2}-4ac[/latex], it tells us whether the solutions are real numbers or complex numbers, and how many solutions of each type to expect. In turn, we can then determine whether a quadratic function has real or complex roots. The table below relates the value of the discriminant to the solutions of a quadratic equation.
| Value of Discriminant | Results |
|---|---|
| [latex]{b}^{2}-4ac=0[/latex] | One repeated rational solution |
| [latex]{b}^{2}-4ac>0[/latex], perfect square | Two rational solutions |
| [latex]{b}^{2}-4ac>0[/latex], not a perfect square | Two irrational solutions |
| [latex]{b}^{2}-4ac<0[/latex] | Two complex solutions |
A General Note: The Discriminant
For [latex]a{x}^{2}+bx+c=0[/latex], where [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are real numbers, the discriminant is the expression under the radical in the quadratic formula: [latex]{b}^{2}-4ac[/latex]. It tells us whether the solutions are real numbers or complex numbers and how many solutions of each type to expect.
Example
Use the discriminant to find the nature of the solutions to the following quadratic equations:
- [latex]{x}^{2}+4x+4=0[/latex]
- [latex]8{x}^{2}+14x+3=0[/latex]
- [latex]3{x}^{2}-5x - 2=0[/latex]
- [latex]3{x}^{2}-10x+15=0[/latex]
We have seen that a quadratic equation may have two real solutions, one real solution, or two complex solutions.
Let’s summarize how the discriminant affects the evaluation of [latex]\sqrt{{{b}^{2}}-4ac}[/latex], and how it helps to determine the solution set.
- If [latex]b^{2}-4ac>0[/latex], then the number underneath the radical will be a positive value. You can always find the square root of a positive, so evaluating the quadratic formula will result in two real solutions (one by adding the positive square root, and one by subtracting it).
- If [latex]b^{2}-4ac=0[/latex], then you will be taking the square root of 0, which is 0. Since adding and subtracting 0 both give the same result, the “[latex]\pm[/late]" portion of the formula doesn't matter. There will be one real repeated solution.
- If [latex]b^{2}-4ac<0[/latex], then the number underneath the radical will be a negative value. Since you cannot find the square root of a negative number using real numbers, there are no real solutions. However, you can use imaginary numbers. You will then have two complex solutions, one by adding the imaginary square root and one by subtracting it.
Example
Use the discriminant to determine how many and what kind of solutions the quadratic equation [latex]x^{2}-4x+10=0[/latex] has.
Important Terms
- axis of symmetry
- a vertical line drawn through the vertex of a parabola around which the parabola is symmetric; it is defined by [latex]x=-\frac{b}{2a}[/latex].
- general form of a quadratic function
- the function that describes a parabola, written in the form [latex]f\left(x\right)=a{x}^{2}+bx+c[/latex], where a, b, and c are real numbers and [latex]a\ne 0[/latex].
- standard form of a quadratic function
- the function that describes a parabola, written in the form [latex]f\left(x\right)=a{\left(x-h\right)}^{2}+k[/latex], where [latex]\left(h,\text{ }k\right)[/latex] is the vertex.
- discriminant
- the value under the radical in the quadratic formula, [latex]b^2-4ac[/latex], which tells whether the quadratic has real or complex roots
- vertex
- the point at which a parabola changes direction, corresponding to the minimum or maximum value of the quadratic function
- zeros
- in a given function, the values of x at which y = 0, also called roots
Candela Citations
- Revision and Adaptation. Provided by: Lumen Learning. License: CC BY: Attribution
- Interactive: Transform Quadratic 1. Provided by: Lumen Learning (with Desmos). Located at: https://www.desmos.com/calculator/fpatj6tbcn. License: CC BY: Attribution
- Interactive: Transform Quadratic 2. Provided by: Lumen Learning (with Desmos). Located at: https://www.desmos.com/calculator/5g3xfhkklq. License: CC BY: Attribution
- Interactive: Transform Quadratic 3. Provided by: Lumen Learning (with Desmos). Located at: https://www.desmos.com/calculator/ha6gh59rq7. License: CC BY: Attribution
- Interactive: Tranform Quadratic 4. Provided by: Lumen Learning (with Desmos). Located at: https://www.desmos.com/calculator/pimelalx4i. License: CC BY: Attribution
- Vertex and Axis of Symmetry Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/q3e3ymnpnn. License: Public Domain: No Known Copyright
- College Algebra. Authored by: Abramson, Jay et al.. Provided by: OpenStax. Located at: http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2. License: CC BY: Attribution. License Terms: Download for free at http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2
- Question ID 120303, 120300. Authored by: Lumen Learning. License: CC BY: Attribution. License Terms: IMathAS Community License CC-BY + GPL
- Question ID# 147099. Authored by: Day,Alyson. License: CC BY: Attribution
- Question ID 35145. Authored by: Jim Smart. License: CC BY: Attribution. License Terms: IMathAS Community License CC-BY + GPL

