Why It Matters: Roots and Rational Exponents

Why Learn About Roots and Rational Exponents?

Crazy eyed cat in the fortheound with a person sleeping in the background.

Go to sleep!

Joan has a cat who likes to come and go at night. He has been keeping her up, asking to go out and come back in, so she wants to devise a way for him to get in and out of her apartment without her needing to open the door for him. After watching the following video on YouTube one day, she decides she will get her brother, Jacob, to help her build a cat ladder so Hobbes can let her sleep at night.

Jacob is pretty clever, so she thinks he can help her with the complicated parts.

Joan’s window is located [latex]12[/latex] feet above the ground, and the edge of the building is [latex]5[/latex] feet from the edge of her window, so Joan needs to figure out how long of a ladder to make so it is confined within this space.

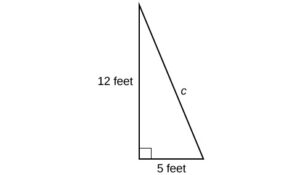
A right triangle with a base of 5 feet, a height of 12 feet, and a hypotenuse labeled c

Even though Joan feels pretty confident about some of the things she has learned in her math class, she is not sure how to find the length for the ladder. She asks her math teacher, and she says that Joan can use the Pythagorean Theorem to find the length of the ladder she needs.

Joan looks up the Pythagorean Theorem and finds that it is an equation that describes the relationship between the lengths of the sides of a right triangle, such as the one a ladder will make with the side of her apartment building. It looks like this:

[latex]{a}^{2}+{b}^{2}={c}^{2}[/latex]

Joan learns that [latex]c^2[/latex] represents the square of the length of the longest side of the right triangle, so in her case this is the length of ladder that she will need. She gets to work trying to solve the equation:

[latex]\begin{array}{ccc}\hfill {a}^{2}+{b}^{2}& =& {c}^{2}\hfill \\ \hfill {5}^{2}+{12}^{2}& =& {c}^{2}\hfill \\ \hfill 169& =& {c}^{2}\hfill \end{array}[/latex]

Joan gets this far and realizes she does not understand how to solve this kind of equation. She needs to find out the length that, when squared, is [latex]169[/latex], to determine which ladder to choose.

What she does not realize is that she needs to find a square root.

In this module, we will investigate methods of finding solutions to problems such as this one. We will revisit Joan later and see if she and Jacob were successful in making a cat ladder for Hobbes.

MODULE 7 Learning Objectives

7.1: Introduction to Radicals

  • Find the square roots of a perfect square
  • Simplify a square root written with the radical symbol
    • When the radicand is a perfect square
    • When the radicand is not a perfect square
    • When the root has a coefficient
  • Approximate a square root
  • Simplify other roots

7.2: Adding and Subtracting Radical Expressions

  • Identify like radicals
  • Simplify an expression by adding and subtracting like radicals
  • Add and subtract radicals that must be simplified first

7.3: Multiplying and Dividing Roots

  • Find the product of two radical terms
  • Multiply a radical and a sum or difference of radicals
  • Multiply binomials containing radicals
  • Simplify the square of a sum or difference of radicals
  • Divide radical expressions

7.4: Solving Equations Containing Square Roots

  • Solve an equation containing a single square root by squaring both sides of the equation
  • Identify extraneous solutions

7.5: Further Exploration with Radicals

  • Use the Pythagorean Theorem to solve applications involving right triangles
  • Find the domain of a radical function