Learning Objectives
By the end of this module, you will be able to:
- Relate cell potentials to free energy changes
- Use the Nernst equation to determine cell potentials at nonstandard conditions
- Perform calculations that involve converting between cell potentials, free energy changes, and equilibrium constants
We will now extend electrochemistry by determining the relationship between [latex]{E}_{\text{cell}}^{\circ }[/latex] and the thermodynamics quantities such as ΔG° (Gibbs free energy) and K (the equilibrium constant). In galvanic cells, chemical energy is converted into electrical energy, which can do work. The electrical work is the product of the charge transferred multiplied by the potential difference (voltage):
[latex]\text{electrical work}=\text{volts}\times \left(\text{charge in coulombs}\right)=\text{J}[/latex]
The charge on 1 mole of electrons is given by Faraday’s constant (F)
[latex]F=\frac{6.022\times{10}^{23}\text{e}^{-}}{\text{mol}}\times\frac{1.602\times{10}^{-19}\text{C}}{\text{e}^{-}}=9.648\times{10}^{4}\frac{\text{C}}{\text{mol}}=9.684\times {10}^{4}\frac{\text{J}}{\text{V}\cdot\text{mol}}[/latex]
[latex]\text{total charge}=\left(\text{number of moles of e}^{-}\right)\times F=nF[/latex]
In this equation, n is the number of moles of electrons for the balanced oxidation-reduction reaction. The measured cell potential is the maximum potential the cell can produce and is related to the electrical work (wele) by
[latex]{E}_{\text{cell}}=\frac{-{w}_{\text{ele}}}{nF}\,\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\,{w}_{\text{ele}}=-nF{E}_{\text{cell}}[/latex]
The negative sign for the work indicates that the electrical work is done by the system (the galvanic cell) on the surroundings. In an earlier chapter, the free energy was defined as the energy that was available to do work. In particular, the change in free energy was defined in terms of the maximum work (wmax), which, for electrochemical systems, is wele.
[latex]\Delta G={w}_{\text{max}}={w}_{\text{ele}}[/latex]
[latex]\Delta G=-nF{E}_{\text{cell}}[/latex]
We can verify the signs are correct when we realize that n and F are positive constants and that galvanic cells, which have positive cell potentials, involve spontaneous reactions. Thus, spontaneous reactions, which have ΔG < 0, must have Ecell > 0. If all the reactants and products are in their standard states, this becomes
[latex]\Delta G^{\circ }=-nF{E}_{\text{cell}}^{\circ }[/latex]
This provides a way to relate standard cell potentials to equilibrium constants, since
[latex]\Delta G^{\circ }=-RT\ln{K}[/latex]
[latex]-nF{E}_{\text{cell}}^{\circ }=-RT\ln{K}\,\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\,{E}_{\text{cell}}^{\circ }=\frac{RT}{nF}\ln{K}[/latex]
Most of the time, the electrochemical reactions are run at standard temperature (298.15 K). Collecting terms at this temperature yields
[latex]{E}_{\text{cell}}^{\circ }=\frac{RT}{nF}\ln{K}=\frac{\left(8.314\frac{\text{J}}{\text{K}\cdot \text{mol}}\right)\left(298.15K\right)}{n\times \text{96,485 C/V}\cdot \text{mol}}\ln{K}=\frac{\text{0.0257 V}}{n}\ln{K}[/latex]
where n is the number of moles of electrons. For historical reasons, the logarithm in equations involving cell potentials is often expressed using base 10 logarithms (log), which changes the constant by a factor of 2.303:
[latex]{E}_{\text{cell}}^{\circ }=\frac{0.0\text{592 V}}{n}\log{K}[/latex]
Thus, if ΔG°, K, or [latex]{E}_{\text{cell}}^{\circ }[/latex] is known or can be calculated, the other two quantities can be readily determined. The relationships are shown graphically in Figure 1.
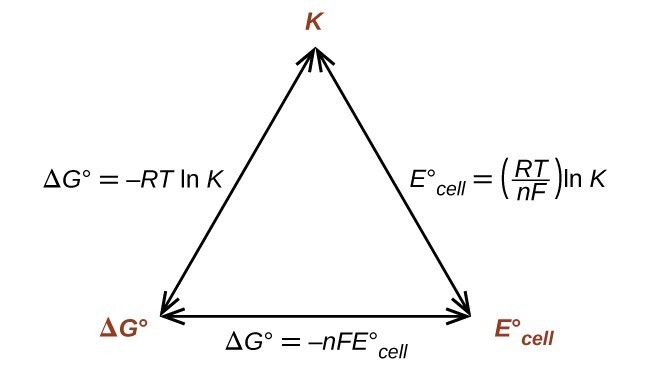
Figure 1. The relationships between ΔG°, K, and Eºcell. Given any one of the three quantities, the other two can be calculated, so any of the quantities could be used to determine whether a process was spontaneous.
Given any one of the quantities, the other two can be calculated.
Example 1: Equilibrium Constants, Standard Cell Potentials, and Standard Free Energy Changes
What is the standard free energy change and equilibrium constant for the following reaction at 25 °C?
[latex]2{\text{Ag}}^{\text{+}}\left(aq\right)+\text{Fe}\left(s\right)\rightleftharpoons \text{2Ag}\left(s\right)+{\text{Fe}}^{2+}\left(aq\right)[/latex]
Check Your Learning
What is the standard free energy change and the equilibrium constant for the following reaction at room temperature? Is the reaction spontaneous?
[latex]\text{Sn}\left(s\right)+2{\text{Cu}}^{2+}\left(aq\right)\rightleftharpoons {\text{Sn}}^{2+}\left(aq\right)+2{\text{Cu}}^{\text{+}}\left(aq\right)[/latex]
Now that the connection has been made between the free energy and cell potentials, nonstandard concentrations follow. Recall that
[latex]\Delta G=\Delta G^{\circ }+RT\ln{Q}[/latex]
where Q is the reaction quotient (see the chapter on equilibrium fundamentals). Converting to cell potentials:
[latex]-nF{E}_{\text{cell}}=-nF{E}_{\text{cell}}^{\circ }+RT\ln{Q}\,\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\,{E}_{\text{cell}}={E}_{\text{cell}}^{\circ }-\frac{RT}{nF}\ln{Q}[/latex]
This is the Nernst equation. At standard temperature (298.15 K), it is possible to write the above equations as
[latex]{E}_{\text{cell}}={E}_{\text{cell}}^{\circ }-\frac{0.0\text{257 V}}{n}\ln{Q}\,\,\,\,\,\,\,\,\text{or}\,\,\,\,\,\,\,\,{E}_{\text{cell}}={E}_{\text{cell}}^{\circ }-\frac{\text{0.0592 V}}{n}\log{Q}[/latex]
If the temperature is not 273.15 K, it is necessary to recalculate the value of the constant. With the Nernst equation, it is possible to calculate the cell potential at nonstandard conditions. This adjustment is necessary because potentials determined under different conditions will have different values.
Example 2: Cell Potentials at Nonstandard Conditions
Consider the following reaction at room temperature:
[latex]\text{Co}\left(s\right)+{\text{Fe}}^{2+}\left(aq,1.94M\right)\longrightarrow {\text{Co}}^{2+}\left(aq\text{, 0.15}M\right)+\text{Fe}\left(s\right)[/latex]
Is the process spontaneous?
Check Your Learning
What is the cell potential for the following reaction at room temperature?
[latex]\text{Al}\left(s\right)\mid {\text{Al}}^{3+}\left(aq,0.15M\right)\parallel {\text{Cu}}^{2+}\left(aq,0.025M\right)\mid \text{Cu}\left(s\right)[/latex]
What are the values of n and Q for the overall reaction? Is the reaction spontaneous under these conditions?
Finally, we will take a brief look at a special type of cell called a concentration cell. In a concentration cell, the electrodes are the same material and the half-cells differ only in concentration. Since one or both compartments is not standard, the cell potentials will be unequal; therefore, there will be a potential difference, which can be determined with the aid of the Nernst equation.
Example 3: Concentration Cells
What is the cell potential of the concentration cell described by
[latex]\text{Zn}\left(s\right)\mid {\text{Zn}}^{2+}\left(aq\text{, 0.10}M\right)\parallel {\text{Zn}}^{2+}\left(aq\text{, 0.50}M\right)\mid \text{Zn}\left(s\right)[/latex]
Check Your Learning
What value of Q for the previous concentration cell would result in a voltage of 0.10 V? If the concentration of zinc ion at the cathode was 0.50 M, what was the concentration at the anode?
Key Concepts and Summary
Electrical work (wele) is the negative of the product of the total charge (Q) and the cell potential (Ecell). The total charge can be calculated as the number of moles of electrons (n) times the Faraday constant (F = 96,485 C/mol e−). Electrical work is the maximum work that the system can produce and so is equal to the change in free energy. Thus, anything that can be done with or to a free energy change can also be done to or with a cell potential. The Nernst equation relates the cell potential at nonstandard conditions to the logarithm of the reaction quotient. Concentration cells exploit this relationship and produce a positive cell potential using half-cells that differ only in the concentration of their solutes.
Key Equations
- [latex]{E}_{\text{cell}}^{\circ }=\frac{RT}{nF}\ln{K}[/latex]
- [latex]{E}_{\text{cell}}^{\circ }=\frac{0.02\text{57 V}}{n}\ln{K}=\frac{0.0\text{592 V}}{n}\log{K}\left(\text{at 298.15}K\right)[/latex]
- [latex]{E}_{\text{cell}}={E}_{\text{cell}}^{\circ }-\frac{RT}{nF}\ln{Q}\text{(Nernst equation)}[/latex]
- [latex]{E}_{\text{cell}}={E}_{\text{cell}}^{\circ }-\frac{0.02\text{57 V}}{n}\ln{Q}={E}_{\text{cell}}^{\circ }-\frac{0.05\text{92 V}}{n}\log{Q}\left(\text{at 298.15}K\right)[/latex]
- ΔG = −nFEcell
- [latex]\Delta {G}^{\circ }=-nF{E}_{\text{cell}}^{\circ }[/latex]
- [latex]{w}_{\text{ele}}={w}_{\text{max}}=-nF{E}_{\text{cell}}[/latex]
Exercises
- For the standard cell potentials given here, determine the ΔG° for the cell in kJ.
- 0.000 V, n = 2
- +0.434 V, n = 2
- −2.439 V, n = 1
- For the ΔG° values given here, determine the standard cell potential for the cell.
- 12 kJ/mol, n = 3
- −45 kJ/mol, n = 1
- Determine the standard cell potential and the cell potential under the stated conditions for the electrochemical reactions described here. State whether each is spontaneous or nonspontaneous under each set of conditions at 298.15 K.
- [latex]\text{Hg}\left(l\right)+{\text{S}}^{2-}\left(aq\text{, 0.10}M\right)+2{\text{Ag}}^{\text{+}}\left(aq\text{, 0.25}M\right)\longrightarrow 2\text{Ag}\left(s\right)+\text{HgS}\left(s\right)[/latex]
- The galvanic cell made from a half-cell consisting of an aluminum electrode in 0.015 M aluminum nitrate solution and a half-cell consisting of a nickel electrode in 0.25 M nickel(II) nitrate solution.
- The cell made of a half-cell in which 1.0 M aqueous bromine is oxidized to 0.11 M bromide ion and a half-cell in which aluminum ion at 0.023 M is reduced to aluminum metal. Assume the standard reduction potential for Br2(l) is the same as that of Br2(aq).
- Determine ΔG and ΔG° for each of the reactions in the previous problem.
- Use the data in Standard Electrode (Half-Cell) Potentials to determine the equilibrium constant for the following reactions. Assume 298.15 K if no temperature is given.
- [latex]\text{AgCl}\left(s\right)\rightleftharpoons {\text{Ag}}^{\text{+}}\left(aq\right)+{\text{Cl}}^{-}\left(aq\right)[/latex]
- [latex]\text{CdS}\left(s\right)\rightleftharpoons {\text{Cd}}^{2+}\left(aq\right)+{\text{S}}^{2-}\left(aq\right)\text{at 377 K}[/latex]
- [latex]{\text{Hg}}^{2+}\left(aq\right)+4{\text{Br}}^{-}\left(aq\right)\rightleftharpoons {\left[{\text{HgBr}}_{4}\right]}^{2-}\left(aq\right)[/latex]
- [latex]{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\text{at 25}^\circ C[/latex]
Glossary
concentration cell: galvanic cell in which the two half-cells are the same except for the concentration of the solutes; spontaneous when the overall reaction is the dilution of the solute
electrical work (wele): negative of total charge times the cell potential; equal to wmax for the system, and so equals the free energy change (ΔG)
Faraday’s constant (F): charge on 1 mol of electrons; F = 96,485 C/mol e−
Nernst equation: equation that relates the logarithm of the reaction quotient (Q) to nonstandard cell potentials; can be used to relate equilibrium constants to standard cell potentials
Candela Citations
- Chemistry. Provided by: OpenStax College. Located at: http://openstaxcollege.org. License: CC BY: Attribution. License Terms: Download for free at https://openstaxcollege.org/textbooks/chemistry/get