14.4 Relative Strengths of Acids and Bases

Learning Objectives

By the end of this module, you will be able to:

Assess the relative strengths of acids and bases according to their ionization constants
Rationalize trends in acid–base strength in relation to molecular structure
Carry out equilibrium calculations for weak acid–base systems

We can rank the strengths of acids by the extent to which they ionize in aqueous solution. The reaction of an acid with water is given by the general expression:

[latex]\text{HA}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{A}}^{-}\left(aq\right)[/latex]

Water is the base that reacts with the acid HA, A⁻ is the conjugate base of the acid HA, and the hydronium ion is the conjugate acid of water. A strong acid yields 100% (or very nearly so) of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] and A⁻ when the acid ionizes in water; Table 1 lists several strong acids. A weak acid gives small amounts of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] and A⁻.

Table 1. Some Common Strong acids and Strong Bases
Strong Acids	Strong Bases
HClO₄ perchloric acid	LiOH lithium hydroxide
HCl hydrochloric acid	NaOH sodium hydroxide
HBr hydrobromic acid	KOH potassium hydroxide
HI hydroiodic acid	Ca(OH)₂ calcium hydroxide
HNO₃ nitric acid	Sr(OH)₂ strontium hydroxide
H₂SO₄ sulfuric acid	Ba(OH)₂ barium hydroxide

The relative strengths of acids may be determined by measuring their equlibrium constants in aqueous solutions. In solutions of the same concentration, stronger acids ionize to a greater extent, and so yield higher concentrations of hydronium ions than do weaker acids. The equilibrium constant for an acid is called the acid-ionization constant, K_a. For the reaction of an acid HA:

[latex]\text{HA}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{A}}^{-}\left(aq\right)[/latex],

we write the equation for the ionization constant as:

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{A}}^{-}\right]}{\text{[HA]}}[/latex]

where the concentrations are those at equilibrium. Although water is a reactant in the reaction, it is the solvent as well, so we do not include [H₂O] in the equation. The larger the K_a of an acid, the larger the concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] and A⁻ relative to the concentration of the nonionized acid, HA. Thus a stronger acid has a larger ionization constant than does a weaker acid. The ionization constants increase as the strengths of the acids increase. (A table of ionization constants of weak acids appears in Ionization Constants of Weak Acids, with a partial listing in Table 1.)

The following data on acid-ionization constants indicate the order of acid strength CH₃CO₂H < HNO₂ < [latex]{\text{HSO}}_{4}{}^{-}:[/latex]

[latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right){K}_{\text{a}}=1.8\times {10}^{-5}[/latex]
[latex]{\text{HNO}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{NO}}_{2}{}^{-}\left(aq\right){K}_{\text{a}}=4.6\times {10}^{-4}[/latex]
[latex]{\text{HSO}}_{4}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(aq\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{SO}}_{4}{}^{2-}\left(aq\right){K}_{\text{a}}=1.2\times {10}^{-2}[/latex]

Another measure of the strength of an acid is its percent ionization. The percent ionization of a weak acid is the ratio of the concentration of the ionized acid to the initial acid concentration, times 100:

[latex]\text{% ionization}=\frac{{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}_{\text{eq}}}{{\left[\text{HA}\right]}_{0}}\times 100[/latex]

Because the ratio includes the initial concentration, the percent ionization for a solution of a given weak acid varies depending on the original concentration of the acid, and actually decreases with increasing acid concentration.

Example 1: Calculation of Percent Ionization from pH

Calculate the percent ionization of a 0.125-M solution of nitrous acid (a weak acid), with a pH of 2.09.

Show Answer

Check Your Learning

Calculate the percent ionization of a 0.10-M solution of acetic acid with a pH of 2.89.

Show Answer

We can rank the strengths of bases by their tendency to form hydroxide ions in aqueous solution. The reaction of a Brønsted-Lowry base with water is given by:

[latex]\text{B}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{HB}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

Water is the acid that reacts with the base, HB⁺ is the conjugate acid of the base B, and the hydroxide ion is the conjugate base of water. A strong base yields 100% (or very nearly so) of OH⁻ and HB⁺ when it reacts with water; Table 1 lists several strong bases. A weak base yields a small proportion of hydroxide ions. Soluble ionic hydroxides such as NaOH are considered strong bases because they dissociate completely when dissolved in water.

Watch this simulation of strong and weak acids and bases at the molecular level.

As we did with acids, we can measure the relative strengths of bases by measuring their base-ionization constant, (K_b) in aqueous solutions. In solutions of the same concentration, stronger bases ionize to a greater extent, and so yield higher hydroxide ion concentrations than do weaker bases. A stronger base has a larger ionization constant than does a weaker base. For the reaction of a base, B:

[latex]\text{B}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{HB}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex],

we write the equation for the ionization constant as:

[latex]{K}_{\text{b}}=\frac{\left[{\text{HB}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[\text{B}\right]}[/latex]

where the concentrations are those at equilibrium. Again, we do not include [H₂O] in the equation because water is the solvent. The chemical reactions and ionization constants of the three bases shown are:

[latex]{\text{NO}}_{2}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{HNO}}_{2}\left(aq\right)+{\text{OH}}^{-}\left(aq\right){K}_{\text{b}}=2.22\times {10}^{-11}[/latex]
[latex]{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{OH}}^{-}\left(aq\right){K}_{\text{b}}=5.6\times {10}^{-10}[/latex]
[latex]{\text{NH}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{NH}}_{4}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right){K}_{\text{b}}=1.8\times {10}^{-5}[/latex]

A table of ionization constants of weak bases appears in Ionization Constants of Weak Bases (with a partial list in Table 2). As with acids, percent ionization can be measured for basic solutions, but will vary depending on the base ionization constant and the initial concentration of the solution.

Consider the ionization reactions for a conjugate acid-base pair, HA − A⁻:

[latex]\text{HA}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{A}}^{-}\left(aq\right){K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{A}}^{-}\right]}{\left[\text{HA}\right]}[/latex]
[latex]{\text{A}}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{OH}}^{-}\left(aq\right)+\text{HA}\left(aq\right){K}_{\text{b}}=\frac{\left[\text{HA}\right]\left[\text{OH}\right]}{\left[{\text{A}}^{-}\right]}[/latex]

Adding these two chemical equations yields the equation for the autoionization for water:

[latex]\cancel{\text{HA}\left(aq\right)}+\text{H}_{2}\text{O}\left(l\right)+\cancel{\text{A}^{-}\left(aq\right)}+\text{H}_{2}\text{O}\left(l\right)\rightleftharpoons\text{H}_{3}\text{O}^{+}\left(aq\right)+\cancel{\text{A}^{-}\left(aq\right)}+\text{OH}^{-}\left(aq\right)+\cancel{\text{HA}\left(aq\right)}[/latex]
[latex]{\text{2H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

As shown in the previous chapter on equilibrium, the K expression for a chemical equation derived from adding two or more other equations is the mathematical product of the other equations’ K expressions. Multiplying the mass-action expressions together and cancelling common terms, we see that:

[latex]{K}_{\text{a}}\times {K}_{\text{b}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{A}}^{-}\right]}{\text{[HA]}}\times \frac{\text{[HA]}\left[{\text{OH}}^{-}\right]}{\left[{\text{A}}^{-}\right]}=\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]={K}_{\text{w}}[/latex]

For example, the acid ionization constant of acetic acid (CH₃COOH) is 1.8 × 10⁻⁵, and the base ionization constant of its conjugate base, acetate ion (CH₃COO⁻), is 5.6 × 10⁻¹⁰. The product of these two constants is indeed equal to K_w:

[latex]{K}_{\text{a}}\times {K}_{\text{b}}=\left(1.8\times {10}^{-5}\right)\times \left(5.6\times {10}^{-10}\right)=1.0\times {10}^{-14}={K}_{\text{w}}[/latex]

The extent to which an acid, HA, donates protons to water molecules depends on the strength of the conjugate base, A⁻, of the acid. If A⁻ is a strong base, any protons that are donated to water molecules are recaptured by A⁻. Thus there is relatively little A⁻ and [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] in solution, and the acid, HA, is weak. If A⁻ is a weak base, water binds the protons more strongly, and the solution contains primarily A⁻ and [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] —the acid is strong. Strong acids form very weak conjugate bases, and weak acids form stronger conjugate bases (Figure 1).

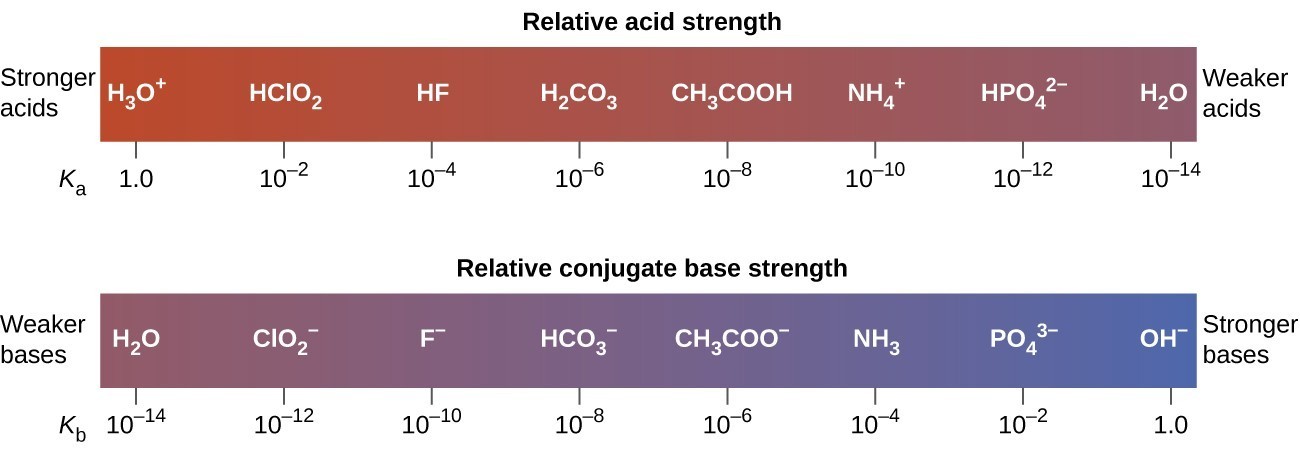
The diagram shows two horizontal bars. The first, labeled, “Relative acid strength,” at the top is red on the left and gradually changes to purple on the right. The red end at the left is labeled, “Stronger acids.” The purple end at the right is labeled, “Weaker acids.” Just outside the bar to the lower left is the label, “K subscript a.” The bar is marked off in increments with a specific acid listed above each increment. The first mark is at 1.0 with H subscript 3 O superscript positive sign. The second is ten raised to the negative two with H C l O subscript 2. The third is ten raised to the negative 4 with H F. The fourth is ten raised to the negative 6 with H subscript 2 C O subscript 3. The fifth is ten raised to a negative 8 with C H subscript 3 C O O H. The sixth is ten raised to the negative ten with N H subscript 4 superscript positive sign. The seventh is ten raised to a negative 12 with H P O subscript 4 superscript 2 negative sign. The eighth is ten raised to the negative 14 with H subscript 2 O. Similarly the second bar, which is labeled “Relative conjugate base strength,” is purple at the left end and gradually becomes blue at the right end. Outside the bar to the left is the label, “Weaker bases.” Outside the bar to the right is the label, “Stronger bases.” Below and to the left of the bar is the label, “K subscript b.” The bar is similarly marked at increments with bases listed above each increment. The first is at ten raised to the negative 14 with H subscript 2 O above it. The second is ten raised to the negative 12 C l O subscript 2 superscript negative sign. The third is ten raised to the negative ten with F superscript negative sign. The fourth is ten raised to a negative eight with H C O subscript 3 superscript negative sign. The fifth is ten raised to the negative 6 with C H subscript 3 C O O superscript negative sign. The sixth is ten raised to the negative 4 with N H subscript 3. The seventh is ten raised to the negative 2 with P O subscript 4 superscript three negative sign. The eighth is 1.0 with O H superscript negative sign.

Figure 1. This diagram shows the relative strengths of conjugate acid-base pairs, as indicated by their ionization constants in aqueous solution.

Figure 2 lists a series of acids and bases in order of the decreasing strengths of the acids and the corresponding increasing strengths of the bases. The acid and base in a given row are conjugate to each other.

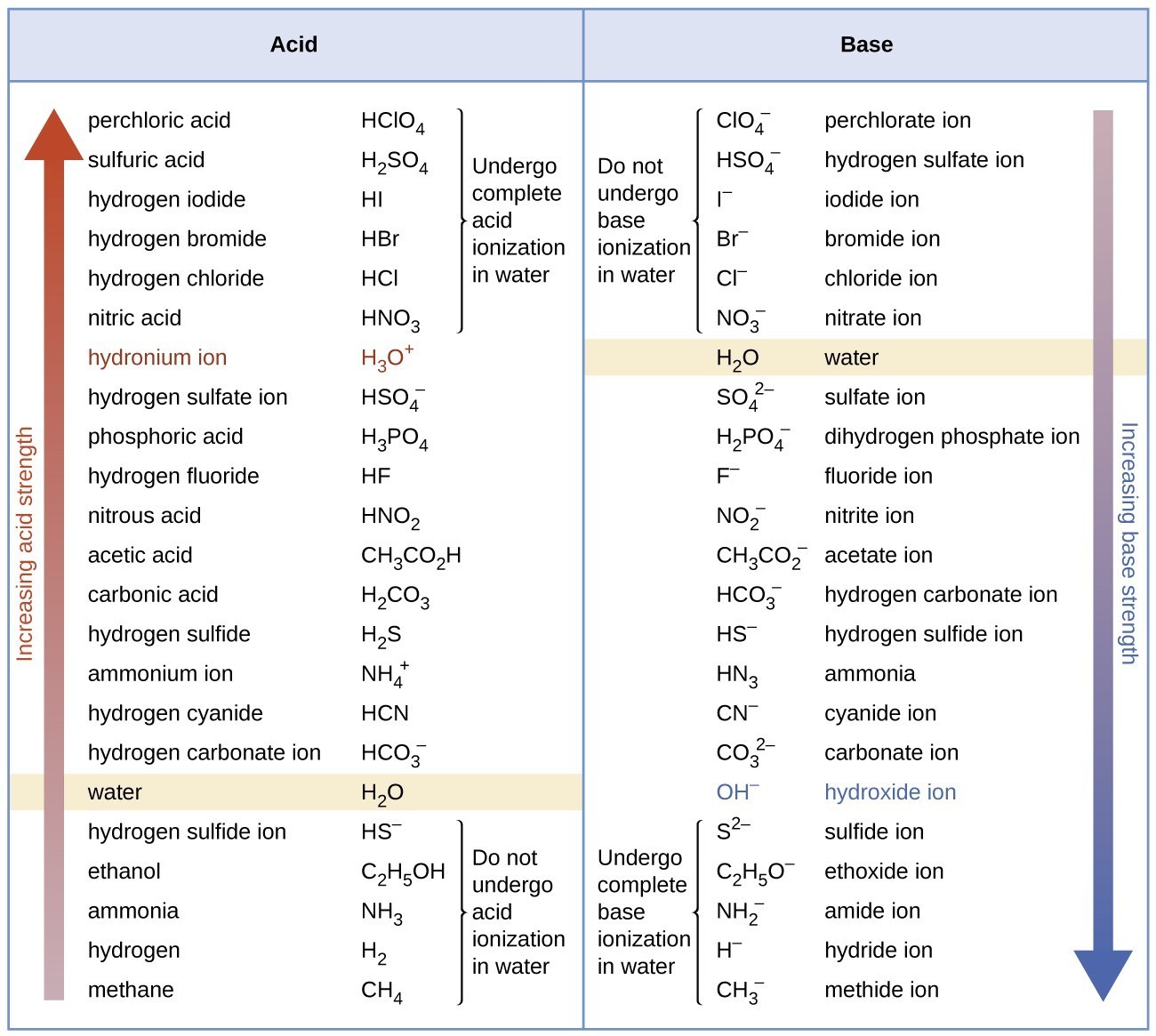
This figure includes a table separated into a left half which is labeled “Acids” and a right half labeled “Bases.” A red arrow points up the left side, which is labeled “Increasing acid strength.” Similarly, a blue arrow points downward along the right side, which is labeled “Increasing base strength.” Names of acids and bases are listed next to each arrow toward the center of the table, followed by chemical formulas. Acids listed top to bottom are sulfuric acid, H subscript 2 S O subscript 4, hydrogen iodide, H I, hydrogen bromide, H B r, hydrogen chloride, H C l, nitric acid, H N O subscript 3, hydronium ion ( in pink text) H subscript 3 O superscript plus, hydrogen sulfate ion, H S O subscript 4 superscript negative, phosphoric acid, H subscript 3 P O subscript 4, hydrogen fluoride, H F, nitrous acid, H N O subscript 2, acetic acid, C H subscript 3 C O subscript 2 H, carbonic acid H subscript 2 C O subscript 3, hydrogen sulfide, H subscript 2 S, ammonium ion, N H subscript 4 superscript +, hydrogen cyanide, H C N, hydrogen carbonate ion, H C O subscript 3 superscript negative, water (shaded in beige) H subscript 2 O, hydrogen sulfide ion, H S superscript negative, ethanol, C subscript 2 H subscript 5 O H, ammonia, N H subscript 3, hydrogen, H subscript 2, methane, and C H subscript 4. The acids at the top of the listing from sulfuric acid through nitric acid are grouped with a bracket to the right labeled “Undergo complete acid ionization in water.” Similarly, the acids at the bottom from hydrogen sulfide ion through methane are grouped with a bracket and labeled, “Do not undergo acid ionization in water.” The right half of the figure lists bases and formulas. From top to bottom the bases listed are hydrogen sulfate ion, H S O subscript 4 superscript negative, iodide ion, I superscript negative, bromide ion, B r superscript negative, chloride ion, C l superscript negative, nitrate ion, N O subscript 3 superscript negative, water (shaded in beige), H subscript 2 O, sulfate ion, S O subscript 4 superscript 2 negative, dihydrogen phosphate ion, H subscript 2 P O subscript 4 superscript negative, fluoride ion, F superscript negative, nitrite ion, N O subscript 2 superscript negative, acetate ion, C H subscript 3 C O subscript 2 superscript negative, hydrogen carbonate ion, H C O subscript 3 superscript negative, hydrogen sulfide ion, H S superscript negative, ammonia, N H subscript 3, cyanide ion, C N superscript negative, carbonate ion, C O subscript 3 superscript 2 negative, hydroxide ion (in blue), O H superscript negative, sulfide ion, S superscript 2 negative, ethoxide ion, C subscript 2 H subscript 5 O superscript negative, amide ion N H subscript 2 superscript negative, hydride ion, H superscript negative, and methide ion C H subscript 3 superscript negative. The bases at the top, from perchlorate ion through nitrate ion are group with a bracket which is labeled “Do not undergo base ionization in water.” Similarly, the lower 5 in the listing, from sulfide ion through methide ion are grouped and labeled “Undergo complete base ionization in water.”

Figure 2. The chart shows the relative strengths of conjugate acid-base pairs.

The first six acids in Figure 2 are the most common strong acids. These acids are completely dissociated in aqueous solution. The conjugate bases of these acids are weaker bases than water. When one of these acids dissolves in water, their protons are completely transferred to water, the stronger base.

Those acids that lie between the hydronium ion and water in Figure 2 form conjugate bases that can compete with water for possession of a proton. Both hydronium ions and nonionized acid molecules are present in equilibrium in a solution of one of these acids. Compounds that are weaker acids than water (those found below water in the column of acids) in Figure 3 exhibit no observable acidic behavior when dissolved in water. Their conjugate bases are stronger than the hydroxide ion, and if any conjugate base were formed, it would react with water to re-form the acid.

The extent to which a base forms hydroxide ion in aqueous solution depends on the strength of the base relative to that of the hydroxide ion, as shown in the last column in Figure 2. A strong base, such as one of those lying below hydroxide ion, accepts protons from water to yield 100% of the conjugate acid and hydroxide ion. Those bases lying between water and hydroxide ion accept protons from water, but a mixture of the hydroxide ion and the base results. Bases that are weaker than water (those that lie above water in the column of bases) show no observable basic behavior in aqueous solution.

Example 2: The Product K_a ×K_b = K_w

Use the K_b for the nitrite ion, [latex]{\text{NO}}_{2}{}^{-}[/latex], to calculate the K_a for its conjugate acid.

Show Answer

Check Your Learning

We can determine the relative acid strengths of [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex] and HCN by comparing their ionization constants. The ionization constant of HCN is given in Ionization Constants of Weak Acids as 4 × 10⁻¹⁰. The ionization constant of [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex] is not listed, but the ionization constant of its conjugate base, NH₃, is listed as 1.8 × 10⁻⁵. Determine the ionization constant of [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex], and decide which is the stronger acid, HCN or [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex].

Show Answer

The Ionization of Weak Acids and Weak Bases

Many acids and bases are weak; that is, they do not ionize fully in aqueous solution. A solution of a weak acid in water is a mixture of the nonionized acid, hydronium ion, and the conjugate base of the acid, with the nonionized acid present in the greatest concentration. Thus, a weak acid increases the hydronium ion concentration in an aqueous solution (but not as much as the same amount of a strong acid).

Acetic acid, CH₃CO₂H, is a weak acid. When we add acetic acid to water, it ionizes to a small extent according to the equation:

giving an equilibrium mixture with most of the acid present in the nonionized (molecular) form. This equilibrium, like other equilibria, is dynamic; acetic acid molecules donate hydrogen ions to water molecules and form hydronium ions and acetate ions at the same rate that hydronium ions donate hydrogen ions to acetate ions to reform acetic acid molecules and water molecules. We can tell by measuring the pH of an aqueous solution of known concentration that only a fraction of the weak acid is ionized at any moment (Figure 3). The remaining weak acid is present in the nonionized form.

For acetic acid, at equilibrium:

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right]}{\left[{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\right]}=1.8\times {10}^{-5}[/latex]

This image shows two bottles containing clear colorless solutions. Each bottle contains a single p H indicator strip. The strip in the bottle on the left is red, and a similar red strip is placed on a filter paper circle in front of the bottle on surface on which the bottles are resting. Similarly, the second bottle on the right contains and orange strip and an orange strip is placed in front of it on a filter paper circle. Between the two bottles is a pack of p Hydrion papers with a p H color scale on its cover.

Figure 3. pH paper indicates that a 0.1 M solution of HCl (beaker on left) has a pH of 1. The acid is fully ionized and [H₃O⁺] = 0.1 M. A 0.1-M solution of CH₃CO₂H (beaker on right) is has a pH of 3 ([H₃O⁺] = 0.001 M) because the weak acid CH₃CO₂H is only partially ionized. In this solution, [H₃O⁺] < [CH₃CO₂H]. (credit: modification of work by Sahar Atwa)

Table 2. Ionization Constants of Some Weak Acids
Ionization Reaction	K_a at 25 °C
[latex]{\text{HSO}}_{4}{}^{-}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{SO}}_{4}{}^{2-}[/latex]	1.2 × 10⁻²
[latex]\text{HF}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{F}}^{-}[/latex]	7.2 × 10⁻⁴
[latex]{\text{HNO}}_{2}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{NO}}_{2}{}^{-}[/latex]	4.5 × 10⁻⁴
[latex]\text{HNCO}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{NCO}}^{-}[/latex]	3.46 × 10⁻⁴
[latex]{\text{HCO}}_{2}\text{H}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{HCO}}_{2}{}^{-}[/latex]	1.8 × 10⁻⁴
[latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}[/latex]	1.8 × 10⁻⁵
[latex]\text{HCIO}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{CIO}}^{-}[/latex]	3.5 × 10⁻⁸
[latex]\text{HBrO}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{BrO}}^{-}[/latex]	2 × 10⁻⁹
[latex]\text{HCN}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}+{\text{CN}}^{-}[/latex]	4 × 10⁻¹⁰

Table 2 gives the ionization constants for several weak acids; additional ionization constants can be found in Ionization Constants of Weak Acids.

At equilibrium, a solution of a weak base in water is a mixture of the nonionized base, the conjugate acid of the weak base, and hydroxide ion with the nonionized base present in the greatest concentration. Thus, a weak base increases the hydroxide ion concentration in an aqueous solution (but not as much as the same amount of a strong base).

For example, a solution of the weak base trimethylamine, (CH₃)₃N, in water reacts according to the equation

[latex]{\left({\text{CH}}_{3}\right)}_{3}\text{N}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex],

giving an equilibrium mixture with most of the base present as the nonionized amine. This equilibrium is analogous to that described for weak acids.

We can confirm by measuring the pH of an aqueous solution of a weak base of known concentration that only a fraction of the base reacts with water (Figure 4). The remaining weak base is present as the unreacted form. The equilibrium constant for the ionization of a weak base, K_b, is called the ionization constant of the weak base, and is equal to the reaction quotient when the reaction is at equilibrium. For trimethylamine, at equilibrium:

[latex]{K}_{\text{b}}=\frac{\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\left({\text{CH}}_{3}\right)}_{3}\text{N}\right]}[/latex]

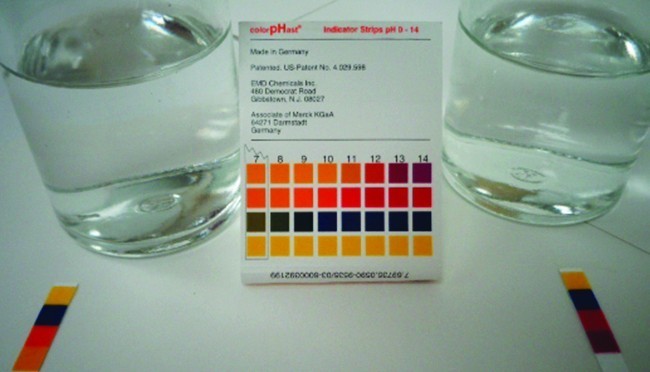
This photo shows two glass containers filled with a transparent liquid. In between the containers is a p H strip indicator guide. There are p H strips placed in front of each glass container. The liquid in the container on the left appears to have a p H of 10 or 11. The liquid in the container on the right appears to have a p H of about 13 or 14.

Figure 4. pH paper indicates that a 0.1 M solution of NH₃ (left) is weakly basic. The solution has a pOH of 3 ([OH⁻] = 0.001 M) because the weak base NH₃ only partially reacts with water. A 0.1 M solution of NaOH (right) has a pOH of 1 because NaOH is a strong base. (credit: modification of work by Sahar Atwa)

The ionization constants of several weak bases are given in Table 3 and in Ionization Constants of Weak Bases.

Table 3. Ionization Constants of Some Weak Bases
Ionization Reaction	K_b at 25 °C
[latex]{\left({\text{CH}}_{3}\right)}_{2}\text{NH}+{\text{H}}_{2}\text{O}\rightleftharpoons {\left({\text{CH}}_{3}\right)}_{2}{\text{NH}}_{2}{}^{\text{+}}+{\text{OH}}^{-}[/latex]	7.4 × 10⁻⁴
[latex]{\text{CH}}_{3}{\text{NH}}_{2}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}+{\text{OH}}^{-}[/latex]	4.4 × 10⁻⁴
[latex]{\left({\text{CH}}_{3}\right)}_{3}\text{N}+{\text{H}}_{2}\text{O}\rightleftharpoons {\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}+{\text{OH}}^{-}[/latex]	7.4 × 10⁻⁵
[latex]{\text{NH}}_{3}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{NH}}_{4}{}^{\text{+}}+{\text{OH}}^{-}[/latex]	1.8 × 10⁻⁵
[latex]{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}+{\text{H}}_{2}\text{O}\rightleftharpoons {\text{C}}_{6}{\text{N}}_{5}{\text{NH}}_{3}{}^{\text{+}}+{\text{OH}}^{-}[/latex]	4.6 × 10⁻¹⁰

Example 3: Determination of K_a from Equilibrium Concentrations

Acetic acid is the principal ingredient in vinegar (Figure 5); that’s why it tastes sour. At equilibrium, a solution contains [CH₃CO₂H] = 0.0787 M and [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right]=0.00118M[/latex]. What is the value of K_a for acetic acid?

An image shows the label of a bottle of distilled white vinegar. The label states that the contents have been reduced with water to 5 percent acidity.

Figure 5. Vinegar is a solution of acetic acid, a weak acid. (credit: modification of work by “HomeSpot HQ”/Flickr)

Show Answer

Check Your Learning

What is the equilibrium constant for the ionization of the [latex]{\text{HSO}}_{4}{}^{-}[/latex] ion, the weak acid used in some household cleansers:

[latex]{\text{HSO}}_{4}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{SO}}_{4}{}^{2-}\left(aq\right)[/latex]

In one mixture of NaHSO₄ and Na₂SO₄ at equilibrium, [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 0.027 M; [latex]\left[{\text{HSO}}_{4}{}^{-}\right]=0.29M[/latex]; and [latex]\left[{\text{SO}}_{4}{}^{2-}\right]=0.13M[/latex].

Show Answer

Example 4: Determination of K_b from Equilibrium Concentrations

Caffeine, C₈H₁₀N₄O₂ is a weak base. What is the value of K_b for caffeine if a solution at equilibrium has [C₈H₁₀N₄O₂] = 0.050 M, [latex]\left[{\text{C}}_{8}{\text{H}}_{10}{\text{N}}_{4}{\text{O}}_{2}{\text{H}}^{\text{+}}\right][/latex] = 5.0 × 10⁻³M, and [OH⁻] = 2.5 × 10⁻³M?

Show Answer

Check Your Learning

What is the equilibrium constant for the ionization of the [latex]{\text{HPO}}_{4}{}^{2-}[/latex] ion, a weak base:

[latex]{\text{HPO}}_{4}{}^{2-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{2}{\text{PO}}_{4}{}^{-}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

In a solution containing a mixture of NaH₂PO₄ and Na₂HPO₄ at equilibrium, [OH⁻] = 1.3 × 10⁻⁶M; [latex]\left[{\text{H}}_{2}{\text{PO}}_{4}{}^{-}\right]=0.042M[/latex]; and [latex]\left[{\text{HPO}}_{4}{}^{2-}\right]=0.341M[/latex].

Show Answer

Example 5: Determination of K_a or K_b from pH

The pH of a 0.0516-M solution of nitrous acid, HNO₂, is 2.34. What is its K_a?

[latex]{\text{HNO}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{NO}}_{2}{}^{-}\left(aq\right)[/latex]

Show Answer

We determine an equilibrium constant starting with the initial concentrations of HNO₂, [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], and [latex]{\text{NO}}_{2}{}^{-}[/latex] as well as one of the final concentrations, the concentration of hydronium ion at equilibrium. (Remember that pH is simply another way to express the concentration of hydronium ion.)

We can solve this problem with the following steps in which x is a change in concentration of a species in the reaction:

We can summarize the various concentrations and changes as shown here (the concentration of water does not appear in the expression for the equilibrium constant, so we do not need to consider its concentration):

To get the various values in the ICE (Initial, Change, Equilibrium) table, we first calculate [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex], the equilibrium concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], from the pH:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]={10}^{-2.34}=0.0046M[/latex]

The change in concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], [latex]{x}_{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}[/latex], is the difference between the equilibrium concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], which we determined from the pH, and the initial concentration, [latex]{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}_{\text{i}}[/latex]. The initial concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] is its concentration in pure water, which is so much less than the final concentration that we approximate it as zero (~0).

The change in concentration of [latex]{\text{NO}}_{2}{}^{-}[/latex] is equal to the change in concentration of [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex]. For each 1 mol of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] that forms, 1 mol of [latex]{\text{NO}}_{2}{}^{-}[/latex] forms. The equilibrium concentration of HNO₂ is equal to its initial concentration plus the change in its concentration.

Now we can fill in the ICE table with the concentrations at equilibrium, as shown here:

Finally, we calculate the value of the equilibrium constant using the data in the table:

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{NO}}_{2}{}^{-}\right]}{\left[{\text{HNO}}_{2}\right]}=\frac{\left(0.0046\right)\left(0.0046\right)}{\left(0.0470\right)}=4.5\times {10}^{-4}[/latex]

Check Your Learning

The pH of a solution of household ammonia, a 0.950-M solution of NH_3, is 11.612. What is K_b for NH₃.

Show Answer

Example 6: Equilibrium Concentrations in a Solution of a Weak Acid

Formic acid, HCO₂H, is the irritant that causes the body’s reaction to ant stings (Figure 6).

A photograph is shown of a large black ant on the end of a human finger.

Figure 6. The pain of an ant’s sting is caused by formic acid. (credit: John Tann)

What is the concentration of hydronium ion and the pH in a 0.534-M solution of formic acid?

[latex]{\text{HCO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{HCO}}_{2}{}^{-}\left(aq\right){K}_{\text{a}}=1.8\times {10}^{-4}[/latex]

Show Answer

Determine x and equilibrium concentrations. The equilibrium expression is:[latex]{\text{HCO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{HCO}}_{2}{}^{-}\left(aq\right)[/latex]
The concentration of water does not appear in the expression for the equilibrium constant, so we do not need to consider its change in concentration when setting up the ICE table.The table shows initial concentrations (concentrations before the acid ionizes), changes in concentration, and equilibrium concentrations follows (the data given in the problem appear in color):
Solve for x and the equilibrium concentrations. At equilibrium: [latex]{K}_{\text{a}}=1.8\times {10}^{-4}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{HCO}}_{2}{}^{-}\right]}{\left[{\text{HCO}}_{2}\text{H}\right]}[/latex]
[latex]=\frac{\left(x\right)\left(x\right)}{0.534-x}=1.8\times {10}^{-4}[/latex]

Now solve for x. Because the initial concentration of acid is reasonably large and K_a is very small, we assume that x << 0.534, which permits us to simplify the denominator term as (0.534 − x) = 0.534. This gives:

[latex]{K}_{\text{a}}=1.8\times {10}^{-4}=\frac{{x}^{\text{2}}}{0.534}[/latex]

Solve for x as follows:

[latex]{x}^{\text{2}}=0.534\times \left(1.8\times {10}^{-4}\right)=9.6\times {10}^{-5}[/latex]
[latex]x=\sqrt{9.6\times {10}^{-5}}[/latex]
[latex]=9.8\times {10}^{-3}[/latex]

To check the assumption that x is small compared to 0.534, we calculate:

[latex]\frac{x}{0.534}=\frac{9.8\times{10}^{-3}}{0.534}=1.8\times{10}^{-2}\left(1.8\%\text{ of 0.534}\right)[/latex]

x is less than 5% of the initial concentration; the assumption is valid.

We find the equilibrium concentration of hydronium ion in this formic acid solution from its initial concentration and the change in that concentration as indicated in the last line of the table:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=~0+x=0+9.8\times {10}^{-3}M[/latex].
[latex]=9.8\times {10}^{-3}M[/latex]

The pH of the solution can be found by taking the negative log of the [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex], so:

[latex]-\text{log}\left(9.8\times {10}^{-3}\right)=2.01[/latex]

Check Your Learning

Only a small fraction of a weak acid ionizes in aqueous solution. What is the percent ionization of acetic acid in a 0.100-M solution of acetic acid, CH₃CO₂H?

(Hint: Determine [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] at equilibrium.) Recall that the percent ionization is the fraction of acetic acid that is ionized × 100, or [latex]\frac{\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right]}{{\left[{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\right]}_{\text{initial}}}\times 100[/latex].

Show Answer

Example 7 shows that the concentration of products produced by the ionization of a weak base can be determined by the same series of steps used with a weak acid.

Example 7: Equilibrium Concentrations in a Solution of a Weak Base

Find the concentration of hydroxide ion in a 0.25-M solution of trimethylamine, a weak base:

Show Answer

Check Your Learning

(a) Show that the calculation in Step 2 of this example gives an x of 4.3 × 10⁻³ and the calculation in Step 3 shows K_b = 7.4 × 10⁻⁵.
(b) Find the concentration of hydroxide ion in a 0.0325-M solution of ammonia, a weak base with a K_b of 1.76 × 10⁻⁵. Calculate the percent ionization of ammonia, the fraction ionized × 100, or [latex]\frac{\left[{\text{NH}}_{4}{}^{\text{+}}\right]}{\left[{\text{NH}}_{3}\right]}\times 100[/latex]

Show Answer

Some weak acids and weak bases ionize to such an extent that the simplifying assumption that x is small relative to the initial concentration of the acid or base is inappropriate. As we solve for the equilibrium concentrations in such cases, we will see that we cannot neglect the change in the initial concentration of the acid or base, and we must solve the equilibrium equations by using the quadratic equation.

Example 8: Equilibrium Concentrations in a Solution of a Weak Acid

Sodium bisulfate, NaHSO₄, is used in some household cleansers because it contains the [latex]{\text{HSO}}_{4}{}^{-}[/latex] ion, a weak acid. What is the pH of a 0.50-M solution of [latex]{\text{HSO}}_{4}{}^{-}?[/latex]

[latex]{\text{HSO}}_{4}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{SO}}_{4}{}^{2-}\left(aq\right){K}_{\text{a}}=1.2\times {10}^{-2}[/latex]

Show Answer

We need to determine the equilibrium concentration of the hydronium ion that results from the ionization of [latex]{\text{HSO}}_{4}{}^{-}[/latex] so that we can use [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] to determine the pH. As in the previous examples, we can approach the solution by the following steps:

Three rectangles are shown with right pointing arrows between them. The first is labeled “Determine x and the equilibrium concentrations.” The second is labeled “Solve for x and the equilibrium concentrations. The third is labeled “Check the math.”

Determine x and equilibrium concentrations. This table shows the changes and concentrations:
Solve for x and the concentrations. As we begin solving for x, we will find this is more complicated than in previous examples. As we discuss these complications we should not lose track of the fact that it is still the purpose of this step to determine the value of x.At equilibrium:
[latex]{K}_{\text{a}}=1.2\times {10}^{-2}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{SO}}_{4}{}^{2-}\right]}{\left[{\text{HSO}}_{4}{}^{-}\right]}=\frac{\left(x\right)\left(x\right)}{0.50-x}[/latex]
If we assume that x is small and approximate (0.50 − x) as 0.50, we find:
[latex]x=7.7\times {10}^{-2}[/latex]
When we check the assumption, we calculate:
[latex]\frac{x}{{\left[{\text{HSO}}_{4}{}^{-}\right]}_{\text{i}}}[/latex][latex]\frac{x}{0.50}=\frac{7.7\times {10}^{-2}}{0.50}=0.15\left(15\%\right)[/latex]
The value of x is not less than 5% of 0.50, so the assumption is not valid. We need the quadratic formula to find x.The equation:
[latex]{K}_{\text{a}}=1.2\times {10}^{-2}=\frac{\left(x\right)\left(x\right)}{0.50-x}[/latex]
gives
[latex]6.0\times {10}^{-3}-1.2\times {10}^{-2}x={x}^{\text{2+}}[/latex]
or
[latex]{x}^{\text{2+}}+1.2\times {10}^{-2}x - 6.0\times {10}^{-3}=0[/latex]
This equation can be solved using the quadratic formula. For an equation of the form
[latex]a{x}^{\text{2+}}+bx+c=0[/latex],
x is given by the equation:
[latex]x=\frac{-b\pm \sqrt{{b}^{\text{2+}}-4ac}}{2a}[/latex]
In this problem, a = 1, b = 1.2 × 10⁻³, and c = −6.0 × 10⁻³.Solving for x gives a negative root (which cannot be correct since concentration cannot be negative) and a positive root:
[latex]x=7.2\times {10}^{-2}[/latex]
Now determine the hydronium ion concentration and the pH:
[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=~0+x=0+7.2\times {10}^{-2}M[/latex][latex]=7.2\times {10}^{-2}M[/latex]
The pH of this solution is:
[latex]\text{pH}=\text{-log}\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\text{-log}7.2\times {10}^{-2}=1.14[/latex]

Check Your Learning

Show that the quadratic formula gives x = 7.2 × 10⁻².
Calculate the pH in a 0.010-M solution of caffeine, a weak base: [latex]{\text{C}}_{8}{\text{H}}_{10}{\text{N}}_{4}{\text{O}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{8}{\text{H}}_{10}{\text{N}}_{4}{\text{O}}_{2}{\text{H}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right){K}_{\text{b}}=2.5\times {10}^{-4}[/latex]

(Hint: It will be necessary to convert [OH⁻] to [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] or pOH to pH toward the end of the calculation.)

Show Answer

The Relative Strengths of Strong Acids and Bases

Strong acids, such as HCl, HBr, and HI, all exhibit the same strength in water. The water molecule is such a strong base compared to the conjugate bases Cl⁻, Br⁻, and I⁻ that ionization of these strong acids is essentially complete in aqueous solutions. In solvents less basic than water, we find HCl, HBr, and HI differ markedly in their tendency to give up a proton to the solvent. For example, when dissolved in ethanol (a weaker base than water), the extent of ionization increases in the order HCl < HBr < HI, and so HI is demonstrated to be the strongest of these acids. The inability to discern differences in strength among strong acids dissolved in water is known as the leveling effect of water.

Water also exerts a leveling effect on the strengths of strong bases. For example, the oxide ion, O²⁻, and the amide ion, [latex]{\text{NH}}_{2}{}^{-}[/latex], are such strong bases that they react completely with water:

[latex]{\text{O}}^{2-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\longrightarrow {\text{OH}}^{-}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]
[latex]{\text{NH}}_{2}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\longrightarrow {\text{NH}}_{3}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

Thus, O²⁻ and [latex]{\text{NH}}_{2}{}^{-}[/latex] appear to have the same base strength in water; they both give a 100% yield of hydroxide ion.

Effect of Molecular Structure on Acid-Base Strength

In the absence of any leveling effect, the acid strength of binary compounds of hydrogen with nonmetals (A) increases as the H-A bond strength decreases down a group in the periodic table. For group 7A, the order of increasing acidity is HF < HCl < HBr < HI. Likewise, for group 6A, the order of increasing acid strength is H₂O < H₂S < H₂Se < H₂Te.

Across a row in the periodic table, the acid strength of binary hydrogen compounds increases with increasing electronegativity of the nonmetal atom because the polarity of the H-A bond increases. Thus, the order of increasing acidity (for removal of one proton) across the second row is CH₄ < NH₃ < H₂O < HF; across the third row, it is SiH₄ < PH₃ < H₂S < HCl (see Figure 7).

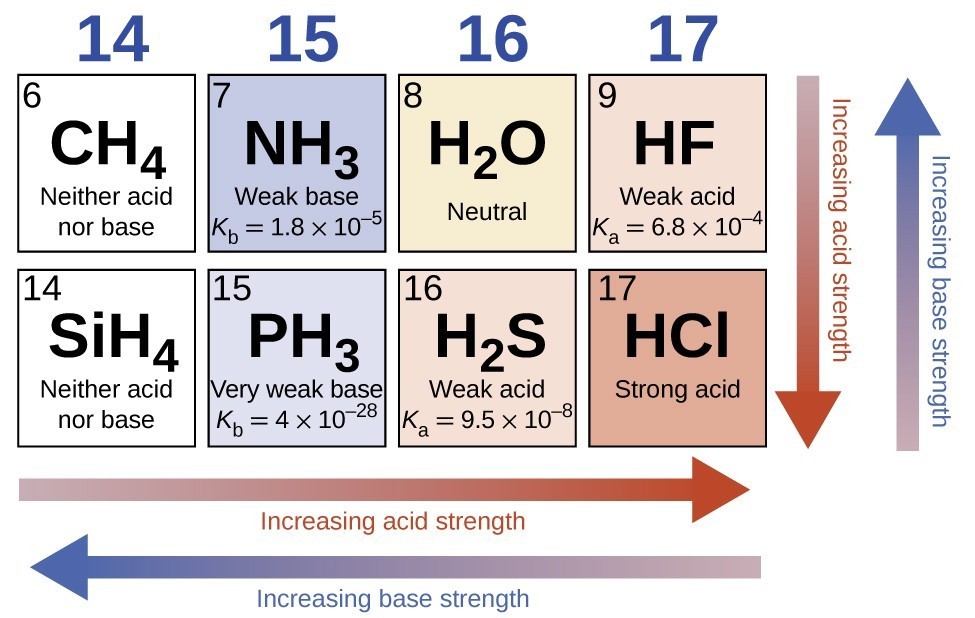
This diagram has two rows and four columns. Red arrows point left across the bottom of the figure and down at the right side and are labeled “Increasing acid strength.” Blue arrows point left across the bottom and up at the right side of the figure and are labeled “Increasing base strength.” The first column is labeled 14 at the top and two white squares are beneath it. The first has the number 6 in the upper left corner and the formula C H subscript 4 in the center along with designation Neither acid nor base. The second square contains the number 14 in the upper left corner, the formula C H subscript 4 at the center and the designation Neither acid nor base. The second column is labeled 15 at the top and two blue squares are beneath it. The first has the number 7 in the upper left corner and the formula N H subscript 3 in the center along with the designation Weak base and K subscript b equals 1.8 times 10 superscript negative 5. The second square contains the number 15 in the upper left corner, the formula P H subscript 3 at the center and the designation Very weak base and K subscript b equals 4 times 10 superscript negative 28. The third column is labeled 16 at the top and two squares are beneath it. The first is shaded tan and has the number 8 in the upper left corner and the formula H subscript 2 O in the center along with the designation neutral. The second square is shaded pink, contains the number 16 in the upper left corner, the formula H subscript 2 S at the center and the designation Weak acid and K subscript a equals 9.5 times 10 superscript negative 8. The fourth column is labeled 17 at the top and two squares are beneath it. The first is shaded pink, has the number 9 in the upper left corner and the formula H F in the center along with the designation Weak acid and K subscript a equals 6.8 times 10 superscript negative 4. The second square is shaded a deeper pink, contains the number 17 in the upper left corner, the formula H C l at the center, and the designation Strong acid.

Figure 7. As you move from left to right and down the periodic table, the acid strength increases. As you move from right to left and up, the base strength increases.

Compounds containing oxygen and one or more hydroxyl (OH) groups can be acidic, basic, or amphoteric, depending on the position in the periodic table of the central atom E, the atom bonded to the hydroxyl group. Such compounds have the general formula O_nE(OH)_m, and include sulfuric acid, O₂S(OH)₂, sulfurous acid, OS(OH)₂, nitric acid, O₂NOH, perchloric acid, O₃ClOH, aluminum hydroxide, Al(OH)₃, calcium hydroxide, Ca(OH)₂, and potassium hydroxide, KOH:
A diagram is shown that includes a central atom designated with the letter E. Single bonds extend above, below, left, and right of the E. An O atom is bonded to the right of the E, and an arrow points to the bond labeling it, “Bond a.” An H atom is single bonded to the right of the O atom. An arrow pointing to this bond connects it to the label, “Bond b.”

If the central atom, E, has a low electronegativity, its attraction for electrons is low. Little tendency exists for the central atom to form a strong covalent bond with the oxygen atom, and bond a between the element and oxygen is more readily broken than bond b between oxygen and hydrogen. Hence bond a is ionic, hydroxide ions are released to the solution, and the material behaves as a base—this is the case with Ca(OH)₂ and KOH. Lower electronegativity is characteristic of the more metallic elements; hence, the metallic elements form ionic hydroxides that are by definition basic compounds.

If, on the other hand, the atom E has a relatively high electronegativity, it strongly attracts the electrons it shares with the oxygen atom, making bond a relatively strongly covalent. The oxygen-hydrogen bond, bond b, is thereby weakened because electrons are displaced toward E. Bond b is polar and readily releases hydrogen ions to the solution, so the material behaves as an acid. High electronegativities are characteristic of the more nonmetallic elements. Thus, nonmetallic elements form covalent compounds containing acidic −OH groups that are called oxyacids.

Increasing the oxidation number of the central atom E also increases the acidity of an oxyacid because this increases the attraction of E for the electrons it shares with oxygen and thereby weakens the O-H bond. Sulfuric acid, H₂SO₄, or O₂S(OH)₂ (with a sulfur oxidation number of +6), is more acidic than sulfurous acid, H₂SO₃, or OS(OH)₂ (with a sulfur oxidation number of +4). Likewise nitric acid, HNO₃, or O₂NOH (N oxidation number = +5), is more acidic than nitrous acid, HNO₂, or ONOH (N oxidation number = +3). In each of these pairs, the oxidation number of the central atom is larger for the stronger acid (Figure 8).

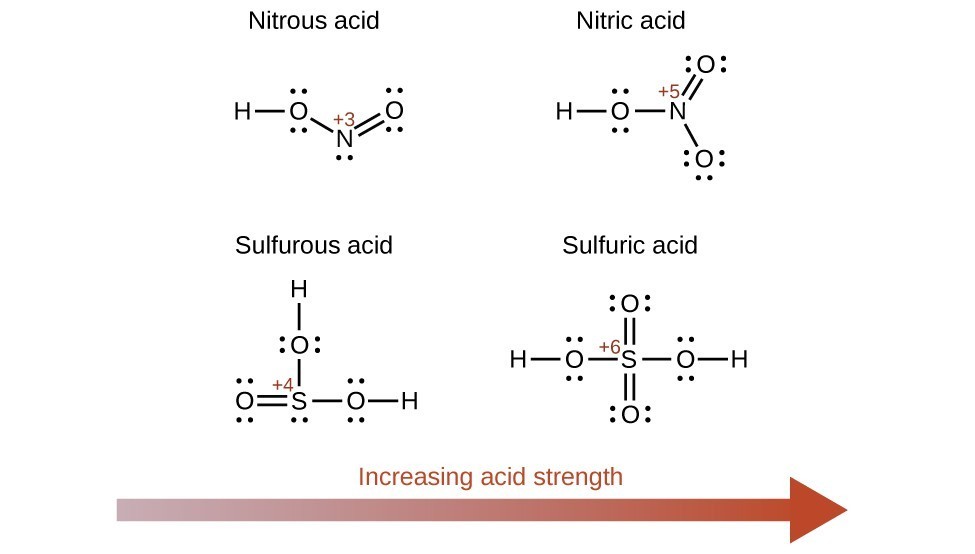
A diagram is shown that includes four structural formulas for acids. A red, right pointing arrow is placed beneath the structures which is labeled “Increasing acid strength.” At the top left, the structure of Nitrous acid is provided. It includes an H atom to which an O atom with two unshared electron pairs is connected with a single bond to the right. A single bond extends to the right and slightly below to a N atom with one unshared electron pair. A double bond extends up and to the right from this N atom to an O atom which has two unshared electron pairs. To the upper right is a structure for Nitric acid. This structure differs from the previous structure in that the N atom is directly to the right of the first O atom and a second O atom with three unshared electron pairs is connected with a single bond below and to the right of the N atom which has no unshared electron pairs. At the lower left, an O atom with two unshared electron pairs is double bonded to its right to an S atom with a single unshared electron pair. An O atom with two unshared electron pairs is bonded above and an H atom is single bonded to this O atom. To the right of the S atom is a single bond to another O atom with two unshared electron pairs to which an H atom is single bonded. This structure is labeled “Sulfurous acid.” A similar structure which is labeled “Sulfuric acid” is placed in the lower right region of the figure. This structure differs in that an H atom is single bonded to the left of the first O atom, leaving it with two unshared electron pairs and a fourth O atom with two unshared electron pairs is double bonded beneath the S atom, leaving it with no unshared electron pairs.

Figure 8. As the oxidation number of the central atom E increases, the acidity also increases.

Hydroxy compounds of elements with intermediate electronegativities and relatively high oxidation numbers (for example, elements near the diagonal line separating the metals from the nonmetals in the periodic table) are usually amphoteric. This means that the hydroxy compounds act as acids when they react with strong bases and as bases when they react with strong acids. The amphoterism of aluminum hydroxide, which commonly exists as the hydrate Al(H₂O)₃(OH)₃, is reflected in its solubility in both strong acids and strong bases. In strong bases, the relatively insoluble hydrated aluminum hydroxide, Al(H₂O)₃(OH)₃, is converted into the soluble ion, [latex]{\left[\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{2}{\left(\text{OH}\right)}_{4}\right]}^{-}[/latex], by reaction with hydroxide ion:

[latex]\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{3}{\left(\text{OH}\right)}_{3}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\rightleftharpoons {\text{H}}_{2}\text{O}\left(l\right)+{\left[\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{2}{\left(\text{OH}\right)}_{4}\right]}^{-}\left(aq\right)[/latex]

In this reaction, a proton is transferred from one of the aluminum-bound H₂O molecules to a hydroxide ion in solution. The Al(H₂O)₃(OH)₃ compound thus acts as an acid under these conditions. On the other hand, when dissolved in strong acids, it is converted to the soluble ion [latex]{\left[\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{6}\right]}^{3+}[/latex] by reaction with hydronium ion:

[latex]{\text{3H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{3}{\left(\text{OH}\right)}_{3}\left(aq\right)\rightleftharpoons \text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{3+}\left(aq\right)+{\text{3H}}_{2}\text{O}\left(l\right)[/latex]

In this case, protons are transferred from hydronium ions in solution to Al(H₂O)₃(OH)₃, and the compound functions as a base.

Key Concepts and Summary

The strengths of Brønsted-Lowry acids and bases in aqueous solutions can be determined by their acid or base ionization constants. Stronger acids form weaker conjugate bases, and weaker acids form stronger conjugate bases. Thus strong acids are completely ionized in aqueous solution because their conjugate bases are weaker bases than water. Weak acids are only partially ionized because their conjugate bases are strong enough to compete successfully with water for possession of protons. Strong bases react with water to quantitatively form hydroxide ions. Weak bases give only small amounts of hydroxide ion. The strengths of the binary acids increase from left to right across a period of the periodic table (CH₄ < NH₃ < H₂O < HF), and they increase down a group (HF < HCl < HBr < HI). The strengths of oxyacids that contain the same central element increase as the oxidation number of the element increases (H₂SO₃ < H₂SO₄). The strengths of oxyacids also increase as the electronegativity of the central element increases [H₂SeO₄ < H₂SO₄].

Key Equations

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{A}}^{-}\right]}{\left[\text{HA}\right]}[/latex]
[latex]{K}_{\text{b}}=\frac{\left[{\text{HB}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[\text{B}\right]}[/latex]
K_a × K_b = 1.0 × 10⁻¹⁴ = K_w
[latex]\text{Percent ionization}=\frac{{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}_{\text{eq}}}{{\left[\text{HA}\right]}_{0}}\times 100[/latex]

Exercises

Explain why the neutralization reaction of a strong acid and a weak base gives a weakly acidic solution.
Explain why the neutralization reaction of a weak acid and a strong base gives a weakly basic solution.
Use this list of important industrial compounds (and Figure 2) to answer the following questions regarding: CaO, Ca(OH)₂, CH₃CO₂H, CO_2, HCl, H₂CO₃, HF, HNO₂, HNO₃, H₃PO₄, H₂SO₄, NH₃, NaOH, Na₂CO₃.
1. Identify the strong Brønsted-Lowry acids and strong Brønsted-Lowry bases.
2. List those compounds in (a) that can behave as Brønsted-Lowry acids with strengths lying between those of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] and H₂O.
3. List those compounds in (a) that can behave as Brønsted-Lowry bases with strengths lying between those of H₂O and OH⁻.
The odor of vinegar is due to the presence of acetic acid, CH₃CO₂H, a weak acid. List, in order of descending concentration, all of the ionic and molecular species present in a 1-M aqueous solution of this acid.
Household ammonia is a solution of the weak base NH₃ in water. List, in order of descending concentration, all of the ionic and molecular species present in a 1-M aqueous solution of this base.
Explain why the ionization constant, K_a, for H₂SO₄ is larger than the ionization constant for H₂SO₃.
Explain why the ionization constant, K_a, for HI is larger than the ionization constant for HF.
Gastric juice, the digestive fluid produced in the stomach, contains hydrochloric acid, HCl. Milk of Magnesia, a suspension of solid Mg(OH)₂ in an aqueous medium, is sometimes used to neutralize excess stomach acid. Write a complete balanced equation for the neutralization reaction, and identify the conjugate acid-base pairs.
Nitric acid reacts with insoluble copper(II) oxide to form soluble copper(II) nitrate, Cu(NO₃)₂, a compound that has been used to prevent the growth of algae in swimming pools. Write the balanced chemical equation for the reaction of an aqueous solution of HNO₃ with CuO.
What is the ionization constant at 25 °C for the weak acid [latex]{\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}[/latex], the conjugate acid of the weak base CH₃NH₂, K_b = 4.4 × 10⁻⁴.
What is the ionization constant at 25 °C for the weak acid [latex]{\left({\text{CH}}_{3}\right)}_{2}{\text{NH}}_{2}{}^{\text{+}}[/latex], the conjugate acid of the weak base (CH₃)₂NH, K_b = 7.4 × 10⁻⁴?
Which base, CH₃NH₂ or (CH₃)₂NH, is the strongest base? Which conjugate acid, [latex]{\left({\text{CH}}_{3}\right)}_{2}{\text{NH}}_{2}{}^{\text{+}}[/latex] or (CH₃)₂NH, is the strongest acid?
Which is the stronger acid, [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex] or HBrO?
Which is the stronger base, (CH₃)₃N or [latex]{\text{H}}_{2}{\text{BO}}_{3}{}^{-}?[/latex]
Predict which acid in each of the following pairs is the stronger and explain your reasoning for each.
1. H₂O or HF
2. B(OH)₃ or Al(OH)₃
3. [latex]{\text{HSO}}_{3}^{-}[/latex] or [latex]{\text{HSO}}_{4}{}^{-}[/latex]
4. NH₃ or H₂S
5. H₂O or H₂Te
Predict which compound in each of the following pairs of compounds is more acidic and explain your reasoning for each.
1. [latex]{\text{HSO}}_{4}^{-}[/latex] or [latex]{\text{HSeO}}_{4}^{-}[/latex]
2. NH₃ or H₂O
3. PH₃ or HI
4. NH₃ or PH₃
5. H₂S or HBr
Rank the compounds in each of the following groups in order of increasing acidity or basicity, as indicated, and explain the order you assign.
1. acidity: HCl, HBr, HI
2. basicity: H₂O, OH⁻, H⁻, Cl⁻
3. basicity: Mg(OH)₂, Si(OH)₄, ClO₃(OH) (Hint: Formula could also be written as HClO₄).
4. acidity: HF, H₂O, NH₃, CH₄
Rank the compounds in each of the following groups in order of increasing acidity or basicity, as indicated, and explain the order you assign.
1. acidity: NaHSO₃, NaHSeO₃, NaHSO₄
2. basicity: [latex]{\text{BrO}}_{2}{}^{-}[/latex], [latex]{\text{ClO}}_{2}{}^{-}[/latex], [latex]{\text{IO}}_{2}{}^{-}[/latex]
3. acidity: HOCl, HOBr, HOI
4. acidity: HOCl, HOClO, HOClO₂, HOClO₃
5. basicity: [latex]{\text{NH}}_{2}{}^{-}[/latex], HS⁻, HTe⁻, [latex]{\text{PH}}_{2}{}^{-}[/latex]
6. basicity: BrO⁻, [latex]{\text{BrO}}_{2}{}^{-}[/latex], [latex]{\text{BrO}}_{3}{}^{-}[/latex], [latex]{\text{BrO}}_{4}{}^{-}[/latex]
Both HF and HCN ionize in water to a limited extent. Which of the conjugate bases, F⁻ or CN⁻, is the stronger base? See Table 3.
The active ingredient formed by aspirin in the body is salicylic acid, C₆H₄OH(CO₂H). The carboxyl group (−CO₂H) acts as a weak acid. The phenol group (an OH group bonded to an aromatic ring) also acts as an acid but a much weaker acid. List, in order of descending concentration, all of the ionic and molecular species present in a 0.001-M aqueous solution of C₆H₄OH(CO₂H).
What do we represent when we write: [latex]{\text{CH}}_{3}{\text{CO}}_{2}\text{H}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\left(aq\right)?[/latex]
Explain why equilibrium calculations are not necessary to determine ionic concentrations in solutions of certain strong electrolytes such as NaOH and HCl. Under what conditions are equilibrium calculations necessary as part of the determination of the concentrations of all ions of some other strong electrolytes in solution?
Are the concentrations of hydronium ion and hydroxide ion in a solution of an acid or a base in water directly proportional or inversely proportional? Explain your answer.
What two common assumptions can simplify calculation of equilibrium concentrations in a solution of a weak acid?
What two common assumptions can simplify calculation of equilibrium concentrations in a solution of a weak base?
Which of the following will increase the percent of NH₃ that is converted to the ammonium ion in water (Hint: Use LeChâtelier’s principle.)?
1. addition of NaOH
2. addition of HCl
3. addition of NH₄Cl
Which of the following will increase the percent of HF that is converted to the fluoride ion in water?
1. addition of NaOH
2. addition of HCl
3. addition of NaF
What is the effect on the concentrations of [latex]{\text{NO}}_{2}{}^{-}[/latex], HNO₂, and OH⁻ when the following are added to a solution of KNO₂ in water:
1. HCl
2. HNO₂
3. NaOH
4. NaCl
5. KNO
The equation for the equilibrium is: [latex]{\text{NO}}_{2}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{HNO}}_{2}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]
What is the effect on the concentration of hydrofluoric acid, hydronium ion, and fluoride ion when the following are added to separate solutions of hydrofluoric acid?
1. HCl
2. KF
3. NaCl
4. KOH
5. HF
The equation for the equilibrium is: [latex]\text{HF}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{F}}^{-}\left(aq\right)[/latex]
Why is the hydronium ion concentration in a solution that is 0.10 M in HCl and 0.10 M in HCOOH determined by the concentration of HCl?
From the equilibrium concentrations given, calculate K_a for each of the weak acids and K_b for each of the weak bases.
1. CH₃CO₂H: [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 1.34 × 10⁻³M;
  [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] = 1.34 × 10⁻³M;
  [CH₃CO₂H] = 9.866 × 10⁻²M;
2. ClO⁻: [OH⁻] = 4.0 × 10⁻⁴M;
  [HClO] = 2.38 × 10⁻⁵M;
  [ClO⁻] = 0.273 M;
3. HCO₂H: [HCO₂H] = 0.524 M;
  [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 9.8 × 10⁻³M;
  [latex]\left[{\text{HCO}}_{2}{}^{-}\right][/latex] = 9.8 × 10⁻³M;
4. [latex]{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}:[/latex] [latex]\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex] = 0.233 M;
  [C₆H₅NH₂] = 2.3 × 10⁻³M;
  [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 2.3 × 10⁻³M
From the equilibrium concentrations given, calculate K_a for each of the weak acids and K_b for each of the weak bases.
1. NH₃: [OH⁻] = 3.1 × 10⁻³M;
  [latex]\left[{\text{NH}}_{4}{}^{\text{+}}\right][/latex] = 3.1 × 10⁻³M;
  [NH₃] = 0.533 M;
2. HNO₂: [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 0.011 M;
  [latex]\left[{\text{NO}}_{2}{}^{-}\right][/latex] = 0.0438 M;
  [HNO₂] = 1.07 M;
3. (CH₃)₃N: [(CH₃)₃N] = 0.25 M;
  [latex]\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right][/latex] = 4.3 × 10⁻³M;
  [OH⁻] = 4.3 × 10⁻³M;
4. [latex]{\text{NH}}_{4}{}^{\text{+}}:[/latex] [latex]\left[{\text{NH}}_{4}{}^{\text{+}}\right][/latex] = 0.100 M;
  [NH₃] = 7.5 × 10⁻⁶M;
  [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 7.5 × 10⁻⁶M
Determine K_b for the nitrite ion, [latex]{\text{NO}}_{2}{}^{-}[/latex]. In a 0.10-M solution this base is 0.0015% ionized.
Determine K_a for hydrogen sulfate ion, [latex]{\text{HSO}}_{4}{}^{-}[/latex]. In a 0.10-M solution the acid is 29% ionized.
Calculate the ionization constant for each of the following acids or bases from the ionization constant of its conjugate base or conjugate acid:
1. F⁻
2. [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex]
3. [latex]{\text{AsO}}_{4}{}^{3-}[/latex]
4. [latex]{\left({\text{CH}}_{3}\right)}_{2}{\text{NH}}_{2}{}^{\text{+}}[/latex]
5. [latex]{\text{NO}}_{2}{}^{-}[/latex]
6. [latex]{\text{HC}}_{2}{\text{O}}_{4}{}^{-}[/latex] (as a base)
Calculate the ionization constant for each of the following acids or bases from the ionization constant of its conjugate base or conjugate acid:
1. HTe⁻ (as a base)
2. [latex]{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}[/latex]
3. [latex]{\text{HAsO}}_{4}{}^{3-}[/latex] (as a base)
4. [latex]{\text{HO}}_{2}{}^{-}[/latex] (as a base)
5. [latex]{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}[/latex]
6. [latex]{\text{HSO}}_{3}{}^{-}[/latex] (as a base)
For which of the following solutions must we consider the ionization of water when calculating the pH or pOH?
1. 3 × 10⁻⁸M HNO₃
2. 0.10 g HCl in 1.0 L of solution
3. 0.00080 g NaOH in 0.50 L of solution
4. 1 × 10⁻⁷M Ca(OH)₂
5. 0.0245 M KNO₃
Even though both NH₃ and C₆H₅NH₂ are weak bases, NH₃ is a much stronger acid than C₆H₅NH₂. Which of the following is correct at equilibrium for a solution that is initially 0.10 M in NH₃ and 0.10 M in C₆H₅NH₂?
1. [latex]\left[{\text{OH}}^{-}\right]=\left[{\text{NH}}_{4}{}^{\text{+}}\right][/latex]
2. [latex]\left[{\text{NH}}_{4}{}^{\text{+}}\right]=\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex]
3. [latex]\left[{\text{OH}}^{-}\right]=\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex]
4. [NH₃] = [C₆H₅NH₂]
5. both a and b are correct

Show Selected Answers

2. The salt ionizes in solution, but the anion slightly reacts with water to form the weak acid. This reaction also forms OH⁻, which causes the solution to be basic. An example is NaCN. The CN⁻ reacts with water as follows: [latex]{\text{CN}}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons \text{HCN}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

4. [H₂O] > [CH₃CO₂H] > [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] ≈ [latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] > [OH⁻]

6. The oxidation state of the sulfur in H₂SO₄ is greater than the oxidation state of the sulfur in H₂SO₃.

8. [latex]\begin{array}{cccccc}\text{Mg}{\left(\text{OH}\right)}_{2}\left(s\right)+& \text{HCl}\left(aq\right)& \longrightarrow & {\text{Mg}}^{2+}\left(aq\right)+& 2{\text{Cl}}^{-}\left(aq\right)+& {\text{2H}}_{2}\text{O}\left(l\right)\\ \text{BB}& \text{BA}& & \text{CB}& \text{CA}& \end{array}[/latex]

10. K_w = K_a × K_b

[latex]{K}_{\text{a}}=\frac{{K}_{\text{w}}}{{K}_{\text{b}}}=\frac{1.0\times {10}^{-14}}{4.4\times {10}^{-4}}=2.3\times {10}^{-11}[/latex]

12. The strongest base or strongest acid is the one with the larger K_b or K_a, respectively. In these two examples, they are (CH₃)₂NH and [latex]{\text{CH}}_{3}{\text{NH}}_{3}{}^{\text{+}}[/latex].

14. Look up Ionization Constants of Weak Bases the value of K_b for (CH₃)₃N and the value of K_a for H₃BO₃. From the latter, calculate the value of K_b for [latex]{\text{H}}_{2}{\text{BO}}_{3}{}^{-}[/latex]. Then compare values:

K_b(CH₃)₃N = 7.4 × 10⁻⁵

[latex]{K}_{\text{a}}\left({\text{H}}_{3}{\text{BO}}_{3}\right)=5.8\times {10}^{-10}=\frac{{K}_{\text{w}}}{{K}_{\text{b}}}[/latex], [latex]{K}_{\text{b}}=\frac{1.0\times {10}^{-14}}{5.8\times {10}^{-10}}=1.7\times {10}^{-5}[/latex]

A comparison shows that the larger K_b is that of triethylamine.

16. The more acidic compounds are as follows:

[latex]{\text{HSO}}_{4}{}^{-}[/latex]; higher electronegativity of the central ion.
H₂O; NH₃ is a base and water is neutral, or decide on the basis of K_a values.
HI; PH₃ is weaker than HCl; HCl is weaker than HI. Thus, PH₃ is weaker than HI.
PH₃; in binary compounds of hydrogen with nonmetals, the acidity increases for the element lower in a group.
HBr; in a period, the acidity increases from left to right; in a group, it increases from top to bottom. Br is to the left and below S, so HBr is the stronger acid.

18. The correct ordered lists are as follows:

NaHSeO₃ < NaHSO₃ < NaHSO₄; in polyoxy acids, the more electronegative central element—S, in this case—forms the stronger acid. The larger number of oxygen atoms on the central atom (giving it a higher oxidation state) also creates a greater release of hydrogen atoms, resulting in a stronger acid. As a salt, the acidity increases in the same manner.
[latex]{\text{ClO}}_{2}{}^{-}<{\text{BrO}}_{2}{}^{-}<{\text{IO}}_{2}{}^{-}[/latex]; the basicity of the anions in a series of acids will be the opposite of the acidity in their oxyacids. The acidity increases as the electronegativity of the central atom increases. Cl is more electronegative than Br, and I is the least electronegative of the three.
HOI < HOBr < HOCl; in a series of the same form of oxyacids, the acidity increases as the electronegativity of the central atom increases. Cl is more electronegative than Br, and I is the least electronegative of the three.
HOCl < HOClO < HOClO₂ < HOClO₃; in a series of oxyacids of the same central element, the acidity increases as the number of oxygen atoms increases (or as the oxidation state of the central atom increases).
[latex]{\text{HTe}}^{-}<{\text{HS}}^{-}<<{\text{PH}}_{2}{}^{-}<{\text{NH}}_{2}{}^{-}[/latex]; [latex]{\text{PH}}_{2}{}^{-}[/latex] and [latex]{\text{NH}}_{2}{}^{-}[/latex] are anions of weak bases, so they act as strong bases toward H⁺. [latex]{\text{HTe}}^{-}[/latex] and HS⁻ are anions of weak acids, so they have less basic character. In a periodic group, the more electronegative element has the more basic anion.
[latex]{\text{BrO}}_{4}{}^{-}<{\text{BrO}}_{3}{}^{-}<{\text{BrO}}_{2}{}^{-}<{\text{BrO}}^{-}[/latex]; with a larger number of oxygen atoms (that is, as the oxidation state of the central ion increases), the corresponding acid becomes more acidic and the anion consequently less basic.

20. [latex]\left[\text{H}_{2}\text{O}\right]\gt\left[\text{C}_{6}\text{H}_{4}\text{OH}\left(\text{CO}_{2}\text{H}\right)\right]\gt\left[\text{H}^{+}\right]\text{O}\gt\left[\text{C}_{6}\text{H}_{4}\text{OH}\left(\text{CO}_{2}\right)^{-}\right]\gt\left[\text{C}_{6}\text{H}_{4}\text{O}\left(\text{CO}_{2}\text{H}\right)^{-}\right]\gt\left[\text{OH}^{-}\right][/latex]

22. Strong electrolytes are 100% ionized, and, as long as the component ions are neither weak acids nor weak bases, the ionic species present result from the dissociation of the strong electrolyte. Equilibrium calculations are necessary when one (or more) of the ions is a weak acid or a weak base.

24. (1) Assume that the change in initial concentration of the acid as the equilibrium is established can be neglected, so this concentration can be assumed constant and equal to the initial value of the total acid concentration. (2) Assume we can neglect the contribution of water to the equilibrium concentration of [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex].

26. The equilibrium is [latex]{\text{NH}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{NH}}_{4}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

The addition of NaOH adds OH⁻ to the system and, according to Le Châtelier’s principle, the equilibrium will shift to the left. Thus, the percent of converted NH₃ will decrease.
The addition of HCl will add [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] ions, which will then react with the OH⁻ ions. Thus, the equilibrium will shift to the right, and the percent will increase.
The addition of NH₄Cl adds [latex]{\text{NH}}_{4}{}^{\text{+}}[/latex] ions, shifting the equilibrium to the left. Thus, the percent will decrease.

28. The effects are as follows:

Adding HCl will add [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex] ions, which will then react with the OH⁻ ions, lowering their concentration. The equilibrium will shift to the right, increasing the concentration of HNO₂, and decreasing the concentration of [latex]{\text{NO}}_{2}{}^{-}[/latex] ions.
Adding HNO₂ increases the concentration of HNO₂ and shifts the equilibrium to the left, increasing the concentration of [latex]{\text{NO}}_{2}{}^{-}[/latex] ions and decreasing the concentration of OH⁻ ions.
Adding NaOH adds OH⁻ ions, which shifts the equilibrium to the left, increasing the concentration of [latex]{\text{NO}}_{2}{}^{-}[/latex] ions and decreasing the concentrations of HNO₂.
Adding NaCl has no effect on the concentrations of the ions.
Adding KNO₂ adds [latex]{\text{NO}}_{2}{}^{-}[/latex] ions and shifts the equilibrium to the right, increasing the HNO₂ and OH⁻ ion concentrations.

30. The equations of the occurring chemical processes are:

[latex]\text{HCl}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\longrightarrow {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{Cl}}^{-}\left(aq\right)[/latex]

[latex]{\text{CH}}_{3}\text{COOH}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CH}}_{3}{\text{COO}}^{-}\left(aq\right)[/latex]

This is a case in which the solution contains a mixture of acids of different ionization strengths. In solution, the HCO₂H exists primarily as HCO₂H molecules because the ionization of the weak acid is suppressed by the strong acid. Therefore, the HCO₂H contributes a negligible amount of hydronium ions to the solution. The stronger acid, HCl, is the dominant producer of hydronium ions because it is completely ionized. In such a solution, the stronger acid determines the concentration of hydronium ions, and the ionization of the weaker acid is fixed by the [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] produced by the stronger acid.

32. K_a and K_b are as follows:

The reaction is [latex]{\text{NH}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{OH}}^{-}\left(aq\right)+{\text{NH}}_{4}{}^{\text{+}}\left(aq\right)[/latex][latex]{K}_{\text{b}}=\frac{\left[{\text{NH}}_{4}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{NH}}_{3}\right]}=\frac{\left(3.1\times {10}^{-3}\right)\left(3.1\times {10}^{-3}\right)}{\left(0.533\right)}=1.8\times {10}^{-5}[/latex]
The reaction is [latex]{\text{HNO}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{NO}}_{2}{}^{-}\left(aq\right)[/latex]
[latex]{K}_{\text{a}}=\frac{\left[{\text{NO}}_{2}{}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{HNO}}_{2}\right]}=\frac{\left(0.0438\right)\left(0.011\right)}{\left(1.07\right)}=4.5\times {10}^{-4}[/latex]
The reaction is [latex]{\left({\text{CH}}_{3}\right)}_{3}\text{N}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{OH}}^{-}\left(aq\right)+{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\left(aq\right)[/latex]
[latex]{K}_{\text{b}}=\frac{\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\left({\text{CH}}_{3}\right)}_{3}\text{N}\right]}=\frac{\left(4.3\times {10}^{-3}\right)\left(4.3\times {10}^{-3}\right)}{\left(0.25\right)}=7.4\times {10}^{-5}[/latex]
The reaction is [latex]{\text{NH}}_{4}{}^{\text{+}}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{NH}}_{3}\left(aq\right)[/latex]
[latex]{K}_{\text{a}}=\frac{\left[{\text{NH}}_{3}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{NH}}_{4}{}^{\text{+}}\right]}=\frac{\left(7.5\times {10}^{-6}\right)\left(7.5\times {10}^{-6}\right)}{\left(0.100\right)}=5.6\times {10}^{-10}[/latex]

34. The reaction is [latex]{\text{HSO}}_{4}{}^{-}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{SO}}_{4}{}^{2-}\left(aq\right)[/latex].

The concentrations at equilibrium are:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = [latex]\left[{\text{SO}}_{4}{}^{2-}\right][/latex] = (0.29)(0.10 M) = 0.029 M
[latex]\left[{\text{HSO}}_{4}{}^{-}\right][/latex] = 0.10 M − 0.029 M = 0.071 M
[latex]{K}_{\text{a}}=\frac{\left[{\text{SO}}_{4}{}^{2-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{HSO}}_{4}{}^{-}\right]}=\frac{\left(0.029\right)\left(0.029\right)}{\left(0.071\right)}=1.2\times {10}^{-2}[/latex]

36. The ionization constants are as follows:

[latex]{K}_{\text{b}}=\frac{{K}_{\text{w}}}{{K}_{\text{a}}}=\frac{\left(1.00\times {10}^{-14}\right)}{2.3\times {10}^{-3}}=4.3\times {10}^{-12}[/latex]
[latex]{K}_{\text{a}}=\frac{{K}_{\text{w}}}{{K}_{\text{b}}}=\frac{\left(1.00\times {10}^{-14}\right)}{7.4\times {10}^{-5}}=1.4\times {10}^{-10}[/latex]
[latex]{K}_{\text{b}}=\frac{{K}_{\text{w}}}{{K}_{\text{a}}}=\frac{\left(1.00\times {10}^{-14}\right)}{1\times {10}^{-7}}=1\times {10}^{-7}[/latex]
[latex]{K}_{\text{b}}=\frac{{K}_{\text{w}}}{{K}_{\text{a}}}=\frac{\left(1.00\times {10}^{-14}\right)}{2.4\times {10}^{-12}}=4.2\times {10}^{-3}[/latex]
[latex]{K}_{\text{b}}=\frac{{K}_{\text{w}}}{{K}_{\text{a}}}=\frac{\left(1.00\times {10}^{-14}\right)}{2.4\times {10}^{-12}}=4.2\times {10}^{-3}[/latex]
[latex]{K}_{\text{b}}=\frac{{K}_{\text{w}}}{{K}_{\text{a}}}=\frac{\left(1.00\times {10}^{-14}\right)}{1.2\times {10}^{-2}}=8.3\times {10}^{-13}[/latex]

38. The reactions are:

[latex]\begin{array}{l}{\text{NH}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{NH}}_{4}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\\ {\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\end{array}[/latex]

Because NH₃ is much stronger than C₆H₅NH₂, it dissociates more. As the initial concentrations of both bases are the same, at equilibrium, [NH₃] < [C₆H₅NH₂], [latex]\left[{\text{NH}}_{4}{}^{\text{+}}\right][/latex] > [latex]\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex], and [OH⁻] > [latex]\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex]. Therefore, (a) is the correct statement. The contribution to the total [OH⁻] at equilibrium from C₆H₅NH₂ is negligible compared to HN₃. Therefore [latex]\left[{\text{OH}}^{-}\right]=\left[{\text{NH}}_{4}{}^{\text{+}}\right][/latex].

Exercises: Calculating Equilibrium Concenration

Calculate the equilibrium concentration of the nonionized acids and all ions in a solution that is 0.25 M in HCO₂H and 0.10 M in HClO.
Calculate the equilibrium concentration of the nonionized acids and all ions in a solution that is 0.134 M in HNO₂ and 0.120 M in HBrO.
Calculate the equilibrium concentration of the nonionized bases and all ions in a solution that is 0.25 M in CH₃NH₂ and 0.10 M in C₅H₅N (K_b = 1.7 × 10⁻⁹).
Calculate the equilibrium concentration of the nonionized bases and all ions in a solution that is 0.115 M in NH₃ and 0.100 M in C₆H₅NH₂.
Using the K_a values in Ionization Constants of Weak Acids, place [latex]\text{Al}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{3+}[/latex] in the correct location in Figure 3.
Calculate the concentration of all solute species in each of the following solutions of acids or bases. Assume that the ionization of water can be neglected, and show that the change in the initial concentrations can be neglected. Ionization constants can be found in Ionization Constants of Weak Acids and Ionization Constants of Weak Bases.
1. 0.0092 M HClO, a weak acid
2. 0.0784 M C₆H₅NH₂, a weak base
3. 0.0810 M HCN, a weak acid
4. 0.11 M (CH₃)₃N, a weak base
5. 0.120 M [latex]\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{2+}[/latex] a weak acid, K_a = 1.6 × 10⁻⁷
Propionic acid, C₂H₅CO₂H (K_a = 1.34 × 10⁻⁵), is used in the manufacture of calcium propionate, a food preservative. What is the hydronium ion concentration in a 0.698-M solution of C₂H₅CO₂H?
White vinegar is a 5.0% by mass solution of acetic acid in water. If the density of white vinegar is 1.007 g/cm³, what is the pH?
The ionization constant of lactic acid, CH₃CH(OH)CO₂H, an acid found in the blood after strenuous exercise, is 1.36 × 10⁻⁴. If 20.0 g of lactic acid is used to make a solution with a volume of 1.00 L, what is the concentration of hydronium ion in the solution?
Nicotine, C₁₀H₁₄N₂, is a base that will accept two protons (K₁ = 7 × 10⁻⁷, K₂ = 1.4 × 10⁻¹¹). What is the concentration of each species present in a 0.050-M solution of nicotine?
The pH of a 0.20-M solution of HF is 1.92. Determine K_a for HF from these data.
The pH of a 0.15-M solution of [latex]{\text{HSO}}_{4}{}^{-}[/latex] is 1.43. Determine K_a for [latex]{\text{HSO}}_{4}{}^{-}[/latex] from these data.
The pH of a 0.10-M solution of caffeine is 11.16. Determine K_b for caffeine from these data:
The pH of a solution of household ammonia, a 0.950 M solution of NH_3, is 11.612. Determine K_b for NH₃ from these data.

Show Answer to Question 40

Question 40

The reactions and equilibrium constants are:

[latex]\begin{array}{l}{\text{HNO}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{NO}}_{2}{}^{-}\left(aq\right){K}_{\text{a}}=4.5\times {10}^{-4}\\ \text{HBrO}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{BrO}}^{-}\left(aq\right){K}_{\text{a}}=2\times {10}^{-9}\end{array}[/latex]

As K_a is much larger for HNO₂ than for HBrO, the first equilibrium will dominate. The equilibrium expression is [latex]{K}_{\text{a}}=\frac{\left[{\text{NO}}_{2}{}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{HNO}}_{2}\right]}=4.5\times {10}^{-5}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.134 − x) ≈ 0.134 gives: [latex]\frac{\left[{\text{NO}}_{2}{}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[{\text{HNO}}_{2}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.134-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.134}=4.5\times {10}^{-4}[/latex]

Solving for x gives 7.77 × 10⁻³M. Because this value is 5.8% of 0.134, our assumption is incorrect. Therefore, we must use the quadratic formula. Using the data gives the following equation: x² + 4.5 × 10⁻⁷x − 6.03 × 10⁻⁵ = 0

Using the quadratic formula gives (a = 1, b = 4.5 × 10⁻⁴, and c = −6.03 × 10⁻⁵)

[latex]\begin{array}{l}{ }x=\frac{-b\pm \sqrt{{b}^{\text{2+}}-4ac}}{2a}=\frac{-\left(4.5\times {10}^{-4}\right)\pm \sqrt{{\left(4.5\times {10}^{-4}\right)}^{\text{2+}}-4\left(1\right)\left(-6.03\times {10}^{-5}\right)}}{2\left(1\right)}\\ =\frac{-\left(4.5\times {10}^{-4}\right)\pm \left(1.55\times {10}^{-2}\right)}{2}=7.54\times {10}^{-3}M\left(\text{positive root}\right)\end{array}[/latex]

The equilibrium concentrations are therefore:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\left[{\text{NO}}_{2}{}^{-}\right]=7.54\times {10}^{-3}=7.5\times {10}^{-3}M[/latex]

[HNO₂] = 0.134 − 7.54 × 10⁻³ = 0.1264 = 0.126

[OH⁻] can be calculated using K_w:

[latex]\left[{\text{OH}}^{-}\right]=\frac{{K}_{\text{w}}}{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}=\frac{1.0\times {10}^{-14}}{7.54\times {10}^{-3}}=1.33\times {10}^{-12}=1.3\times {10}^{-12}M[/latex]

Finally, use the other equilibrium to find the other concentrations. Assume for [HBrO] that (0.120 − x) ≈ 0.124 M.

[latex]{K}_{\text{a}}=\frac{\left[{\text{BrO}}^{-}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[\text{HBrO}\right]}=2\times {10}^{-9}\approx \frac{\left[{\text{BrO}}^{-}\right]\left(7.54\times {10}^{-3}\right)}{0.120}[/latex]

Solving for [BrO⁻] gives:

[BrO⁻] = 3.2 × 10⁻⁸ = 3.2 × 10⁻⁸M
[HBrO] = 0.120 − 3.2 × 10⁻⁸ = 0.120 M

Show Answer to Question 42

Question 42

The reactions and equilibrium constants are:

[latex]\begin{array}{l}\\ {\text{NH}}_{3}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{OH}}^{-}\left(aq\right)+{\text{NH}}_{4}{}^{\text{+}}\left(aq\right){K}_{\text{b}}=1.8\times {10}^{-5}\\ {\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{OH}}^{-}\left(aq\right)+{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\left(aq\right){K}_{\text{b}}=4.6\times {10}^{-10}\end{array}[/latex]

As K_b is much larger for NH₃ than for C₆H₅NH_2, the first equilibrium will dominate. The equilibrium expression is [latex]{K}_{\text{b}}=\frac{\left[{\text{NH}}_{4}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{NH}}_{3}\right]}=1.8\times {10}^{-5}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.115 − x) ≈ 0.115 gives:

[latex]\frac{\left[{\text{NH}}_{4}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{NH}}_{3}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.115-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.115}=1.8\times {10}^{-5}[/latex]

Solving for x gives 1.44 × 10⁻³M. Because this value is less than 5% of 0.115 M, our assumption is correct. The equilibrium concentrations are therefore:

[OH⁻] = [latex]\left[{\text{NO}}_{4}{}^{\text{+}}\right][/latex] = 1.44 × 10⁻³ = 0.0014 M

[NH₃] = 0.115 − 0.00144 = 0.1136 = 0.144 M

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] can be calculated using K_w:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\frac{{K}_{\text{w}}}{\left[{\text{OH}}^{-}\right]}=\frac{1.0\times {10}^{-14}}{0.00144}=6.94\times {10}^{-12}=6.9\times {10}^{-12}M[/latex]

Finally, use the other equilibrium to find the other concentrations. Assume for [C₆H₅NH₂] that (0.100 − x) ≈ 0.100 M:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\frac{{K}_{\text{w}}}{\left[{\text{OH}}^{-}\right]}=\frac{1.0\times {10}^{-14}}{0.00144}=6.94\times {10}^{-12}=6.9\times {10}^{-12}M[/latex]

Solving for [latex]\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex] gives:

[latex]\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right][/latex] = 3.9 × 10⁻⁸M

[C₆H₅NH₂] = 0.100 − 3.19 × 10⁻⁸ = 0.100 M

Show Answer to Question 44

Question 44

Part A

The reaction is: [latex]\text{HClO}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{ClO}}^{-}\left(aq\right)[/latex]

The equilibrium expression is:

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{ClO}}^{-}\right]}{\left[\text{HClO}\right]}=3.5\times {10}^{-8}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.0092 − x) ≈ 0.0092 gives:

[latex]\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{ClO}}^{-}\right]}{\left[\text{HClO}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.0092-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.0092}=3.5\times {10}^{-8}[/latex]

Solving for x gives 1.79 × 10⁻⁵M. This value is less than 5% of 0.0092, so the assumption that it can be neglected is valid. Thus, the concentrations of solute species at equilibrium are:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = [ClO] = 1.8 × 10⁻⁵M

[HClO] = 0.0092 − 1.79 × 10⁻⁵ = 0.00918 = 0.00092 M

[latex]\left[{\text{OH}}^{-}\right]=\frac{{K}_{\text{w}}}{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}=\frac{1.0\times {10}^{-14}}{1.79\times {10}^{-5}}=5.6\times {10}^{-10}M[/latex];

Part B

The reaction is:

[latex]{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]

The equilibrium expression is:

[latex]{K}_{\text{a}}=\frac{\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}\right]}=4.6\times {10}^{-10}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.0784 − x) ≈ 0.0784 gives:

[latex]\frac{\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{3}{}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{C}}_{6}{\text{H}}_{5}{\text{NH}}_{2}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.0784-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.0784}=4.6\times {10}^{-10}[/latex]

Solving for x gives 6.01 × 10⁻⁶M. This value is less than 5% of 0.0784, so the assumption that it can be neglected is valid. Thus, the concentrations of solute species at equilibrium are:

[latex]\left[{\text{CH}}_{3}{\text{CO}}_{2}{}^{-}\right][/latex] = [OH⁻] = 6.0 × 10⁻⁶M

[C₆H₅NH₂] = 0.0784 − 6.01 × 10⁻⁶ = 0.007839 = 0.00784

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\frac{{K}_{\text{w}}}{\left[{\text{OH}}^{-}\right]}=\frac{1.0\times {10}^{-14}}{6.01\times {10}^{-6}}=1.7\times {10}^{-9}M[/latex];

Part C

The reaction is [latex]\text{HCN}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)+{\text{CN}}^{-}\left(aq\right)[/latex].

The equilibrium expression is:

[latex]{K}_{\text{a}}=\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{CN}}^{-}\right]}{\left[\text{HCN}\right]}=4\times {10}^{-10}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.0810 − x) ≈ 0.0810 gives:

[latex]\frac{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]\left[{\text{CN}}^{-}\right]}{\left[\text{HCN}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.0810-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.0810}=4\times {10}^{-10}[/latex]

Solving for x gives 5.69 × 10⁻⁶M. This value is less than 5% of 0.0810, so the assumption that it can be neglected is valid. Thus, the concentrations of solute species at equilibrium are:

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = [CN⁻] = 5.7 × 10⁻⁶M

[HCN] = 0.0810 − 5.69 × 10⁻⁶ = 0.08099 = 0.0810 M

[latex]\left[{\text{OH}}^{-}\right]=\frac{{K}_{\text{w}}}{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}=\frac{1.0\times {10}^{-14}}{5.69\times {10}^{-6}}=1.8\times {10}^{-9}M[/latex];

Part D

The reaction is:

The equilibrium expression is:

[latex]{K}_{\text{b}}=\frac{\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\left({\text{CH}}_{3}\right)}_{3}\text{N}\right]}=7.4\times {10}^{-5}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.11 − x) ≈ 0.11 gives:

[latex]\frac{\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\left({\text{CH}}_{3}\right)}_{3}\text{N}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.11-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.11}=7.4\times {10}^{-5}[/latex]

Solving for x gives 2.85 × 10⁻³M. This value is less than 5% of 0.11, so the assumption that it can be neglected is valid. Thus, the concentrations of solute species at equilibrium are:

[latex]\left[{\left({\text{CH}}_{3}\right)}_{3}{\text{NH}}^{\text{+}}\right][/latex] = [OH⁻] = 2.9 × 10⁻³M

[(CH₃)₃N] = 0.11 − 2.85 × 10⁻³ = 0.107 = 0.11 M

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\frac{{K}_{\text{w}}}{\left[{\text{OH}}^{-}\right]}=\frac{1.0\times {10}^{-14}}{2.85\times {10}^{-3}}=3.5\times {10}^{-12}M[/latex];

Part E

The reaction is:

[latex]\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{2+}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons \text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{5}{\left(\text{OH}\right)}^{\text{+}}\left(aq\right)+{\text{H}}_{3}{\text{O}}^{\text{+}}\left(aq\right)[/latex]

The equilibrium expression is:

[latex]{K}_{\text{a}}=\frac{\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{5}{\left(\text{OH}\right)}^{\text{+}}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{2+}\right]}=1.6\times {10}^{-7}[/latex]

The initial and equilibrium concentrations for this system can be written as follows:

Substituting the equilibrium concentrations into the equilibrium expression and making the assumption that (0.120 − x) ≈ 0.120 gives:

[latex]\frac{\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{5}{\left(\text{OH}\right)}^{\text{+}}\right]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}{\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{2+}\right]}=\frac{\left(x\right)\left(x\right)}{\left(0.120-x\right)}\approx \frac{\left(x\right)\left(x\right)}{0.120}=1.6\times {10}^{-7}[/latex]

Solving for x gives 1.39 × 10⁻⁴M. This value is less than 5% of 0.120, so the assumption that it can be neglected is valid. Thus, the concentrations of solute species at equilibrium are:

[latex]\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{5}{\left(\text{OH}\right)}^{\text{+}}\right][/latex] = [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 1.4 × 10⁻⁴M

[latex]\left[\text{Fe}{\left({\text{H}}_{2}\text{O}\right)}_{6}{}^{2+}\right][/latex] = 0.120 − 1.39 × 10⁻⁴ = 0.1199 = 0.120 M

[latex]\left[{\text{OH}}^{-}\right]=\frac{{K}_{\text{w}}}{\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]}=\frac{1.0\times {10}^{-14}}{1.39\times {10}^{-4}}=7.2\times {10}^{-11}M[/latex]

Show Answer to Question 46

Show Answer to Question 48

Question 48

[latex]\begin{array}{l}{ }{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\left({K}_{\text{b1}}=7\times {10}^{-7}\right)\\ {\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)\left({K}_{\text{b2}}=1.4\times {10}^{-11}\right)\end{array}[/latex]

First set up a concentration table:

Substituting the equilibrium concentrations into the equilibrium equation and making the assumption that (0.050 − x) = 0.050, we get:

[latex]\begin{array}{c}{K}_{\text{b1}}=\frac{\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}\right]}=7\times {10}^{-7}\\ =\frac{\left(x\right)\left(x\right)}{\left(0.050-x\right)}=\frac{{x}^{\text{2+}}}{0.050}=7\times {10}^{-7}\end{array}[/latex]

Solving for x gives 1.87 × 10⁻⁴ = 2 × 10⁻⁴M = [OH⁻]

Because x is less than 5% of 0.050 and [OH⁻] is greater than 4.5 × 10⁻⁷M, our customary assumptions are justified. We can calculate [C₁₀H₁₄N₂] = 0.050 − x = 0.050 − 2 × 10⁻⁴ = 0.048 M; [OH⁻] = [latex]\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\right][/latex] = x = 2 × 10⁻⁴M. Now calculate the concentration of [latex]{\text{C}}_{10}{\text{H}}_{2}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}[/latex] in a solution with [OH⁻] and [latex]\left[{\text{C}}_{10}{\text{H}}_{2}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\right][/latex] equal to 2 × 10⁻⁴M. The equilibrium between these species is [latex]{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\left(aq\right)+{\text{H}}_{2}\text{O}\left(l\right)\rightleftharpoons {\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{\text{2+}}\left(aq\right)+{\text{OH}}^{-}\left(aq\right)[/latex]. We know [C₁₀H₁₄N₂H⁺] and [OH⁻], so we can calculate the concentration of [latex]{\text{C}}_{10}{\text{H}}_{2}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}[/latex] from the equilibrium expression:

[latex]\begin{array}{l}\\ \\ {K}_{\text{b2}}=\frac{\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\right]\left[{\text{OH}}^{-}\right]}{\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\right]}=1.4\times {10}^{-11}\\ =\frac{\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\right]\left[2\times {10}^{-4}\right]}{\left[2\times {10}^{-4}\right]}\\ \left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\right]=1.4\times {10}^{-11}M\end{array}[/latex]

The concentration of OH⁻ produced in this ionization is equal to the concentration of [latex]{\text{C}}_{10}{\text{H}}_{2}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}[/latex], 1.4 × 10⁻¹¹M, which is much smaller than the 2 ×10⁻⁴M produced in the first ionization; therefore, we are justified in neglecting the OH⁻ formed from [latex]{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}[/latex].

We can now calculate the concentration of H₂O⁺ present from the ionization of water:

K_a = 1 × 10⁻¹⁴ = [latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] [OH⁻]

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right]=\frac{1\times {10}^{-14}}{\left[{\text{OH}}^{-}\right]}=\frac{1\times {10}^{-14}}{1.9\times {10}^{-4}}=5.3\times {10}^{-11}M[/latex]

We can now summarize the concentrations of all species in solution as follows:

[C₁₀H₁₄N₂] = 0.049 M

[latex]\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}^{\text{+}}\right][/latex] = 1.9 × 10⁻⁴M

[latex]\left[{\text{C}}_{10}{\text{H}}_{14}{\text{N}}_{2}{\text{H}}_{2}{}^{2+}\right]=1.4\times {10}^{-11}M[/latex]

[OH⁻] = 1.9 × 10⁻⁴M

[latex]\left[{\text{H}}_{3}{\text{O}}^{\text{+}}\right][/latex] = 5.3 × 10⁻¹¹M

Show Answer to Question 50

Show Answer to Question 52

Glossary

acid ionization constant (K_a): equilibrium constant for the ionization of a weak acid

base ionization constant (K_b): equilibrium constant for the ionization of a weak base

leveling effect of water: any acid stronger than [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], or any base stronger than OH⁻ will react with water to form [latex]{\text{H}}_{3}{\text{O}}^{\text{+}}[/latex], or OH⁻, respectively; water acts as a base to make all strong acids appear equally strong, and it acts as an acid to make all strong bases appear equally strong

oxyacid: compound containing a nonmetal and one or more hydroxyl groups

percent ionization: ratio of the concentration of the ionized acid to the initial acid concentration, times 100

Chapter 14: Acid-Base Equilibria

Learning Objectives

Example 1: Calculation of Percent Ionization from pH

Check Your Learning

Example 2: The Product K_a ×K_b = K_w

Check Your Learning

The Ionization of Weak Acids and Weak Bases

Example 3: Determination of K_a from Equilibrium Concentrations

Check Your Learning

Example 4: Determination of K_b from Equilibrium Concentrations

Check Your Learning

Example 5: Determination of K_a or K_b from pH

Check Your Learning

Example 6: Equilibrium Concentrations in a Solution of a Weak Acid

Check Your Learning

Example 7: Equilibrium Concentrations in a Solution of a Weak Base

Check Your Learning

Example 8: Equilibrium Concentrations in a Solution of a Weak Acid

Check Your Learning

The Relative Strengths of Strong Acids and Bases

Effect of Molecular Structure on Acid-Base Strength

Key Concepts and Summary

Key Equations

Exercises

Exercises: Calculating Equilibrium Concenration

Question 40

Question 42

Question 44

Part A

Part B

Part C

Part D

Part E

Question 46

Question 48

Question 50

Question 52

Glossary

Candela Citations

Chapter 14: Acid-Base Equilibria