Learning Outcomes
- Identify polynomials, monomials, binomials, and trinomials
- Determine the degree of polynomials
Polynomials come in many forms. They can vary by how many terms, or monomials, make up the polynomial and they also can vary by the degrees of the monomials in the polynomial. In this section, we will look at different ways that we classify polynomials. First, we will classify polynomials by the number of terms in the polynomial and then we will classify them by the monomial with the largest exponent.
Identify Polynomials, Monomials, Binomials, and Trinomials
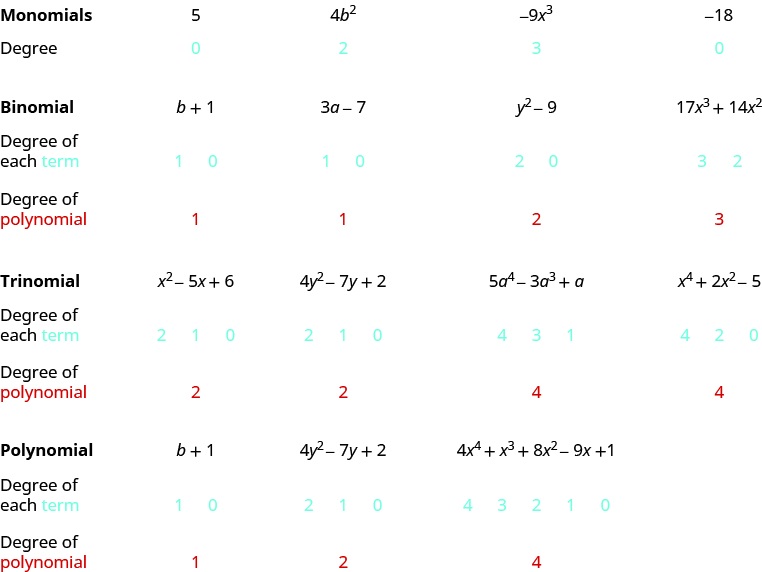
A monomial, or a sum and/or difference of monomials, is called a polynomial. A polynomial containing two terms, such as [latex]2x - 9[/latex], is called a binomial. A polynomial containing three terms, such as [latex]-3{x}^{2}+8x - 7[/latex], is called a trinomial.
Polynomials
polynomial—A monomial, or two or more monomials, combined by addition or subtraction (“poly” means many)
monomial—A polynomial with exactly one term (“mono” means one)
binomial— A polynomial with exactly two terms (“bi” means two)
trinomial—A polynomial with exactly three terms (“tri” means three)
Here are some examples of polynomials:
| Polynomial | [latex]b+1[/latex] | [latex]4{y}^{2}-7y+2[/latex] | [latex]5{x}^{5}-4{x}^{4}+{x}^{3}+8{x}^{2}-9x+1[/latex] |
| Monomial | [latex]5[/latex] | [latex]4{b}^{2}[/latex] | [latex]-9{x}^{3}[/latex] |
| Binomial | [latex]3a - 7[/latex] | [latex]{y}^{2}-9[/latex] | [latex]17{x}^{3}+14{x}^{2}[/latex] |
| Trinomial | [latex]{x}^{2}-5x+6[/latex] | [latex]4{y}^{2}-7y+2[/latex] | [latex]5{a}^{4}-3{a}^{3}+a[/latex] |
Notice that every monomial, binomial, and trinomial is also a polynomial. They are special members of the family of polynomials and so they have special names. We use the words ‘monomial’, ‘binomial’, and ‘trinomial’ when referring to these special polynomials and just call all the rest ‘polynomials’.
example
Determine whether each polynomial is a monomial, binomial, trinomial, or other polynomial:
1. [latex]8{x}^{2}-7x - 9[/latex]
2. [latex]-5{a}^{4}[/latex]
3. [latex]{x}^{4}-7{x}^{3}-6{x}^{2}+5x+2[/latex]
4. [latex]11 - 4{y}^{3}[/latex]
5. [latex]n[/latex]
Solution
| Polynomial | Number of terms | Type | |
|---|---|---|---|
| 1. | [latex]8{x}^{2}-7x - 9[/latex] | [latex]3[/latex] | Trinomial |
| 2. | [latex]-5{a}^{4}[/latex] | [latex]1[/latex] | Monomial |
| 3. | [latex]{x}^{4}-7{x}^{3}-6{x}^{2}+5x+2[/latex] | [latex]5[/latex] | Polynomial |
| 4. | [latex]11 - 4{y}^{3}[/latex] | [latex]2[/latex] | Binomial |
| 5. | [latex]n[/latex] | [latex]1[/latex] | Monomial |
Example
For the following expressions, determine whether they are a polynomial. If so, categorize them as a monomial, binomial, or trinomial.
- [latex]\frac{x-3}{1-x}+x^2[/latex]
- [latex]t^2+2t-3[/latex]
- [latex]x^3+\frac{x}{8}[/latex]
- [latex]\frac{\sqrt{y}}{2}-y-1[/latex]
try it
In the following video, you will be shown more examples of how to identify and categorize polynomials.
Determining the Degree of Polynomials
We can find the degree of a polynomial by identifying the highest power of the variable that occurs in the polynomial. Polynomials can be classified by the degree of the polynomial. The degree of a polynomial is the degree of its highest degree term. So the degree of [latex]2x^{3}+3x^{2}+8x+5[/latex] is 3.
A polynomial is said to be written in standard form when the terms are arranged from the highest degree to the lowest degree. When it is written in standard form it is easy to determine the degree of the polynomial. The term with the highest degree is called the leading term because it is written first in standard form. The coefficient of the leading term is called the leading coefficient.
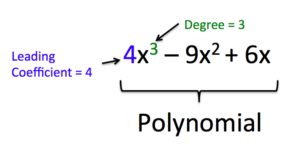
How to: Given a polynomial expression, identify the degree and leading coefficient
- Find the highest power of the variable (usually x) to determine the degree.
- Identify the term containing the highest power of the variable to find the leading term.
- Identify the coefficient of the leading term.
Degree of a Polynomial
The degree of a term is the exponent of its variable.
The degree of a constant is [latex]0[/latex].
The degree of a polynomial is the highest degree of all its terms.
When the coefficient of a polynomial term is [latex]0[/latex], you usually do not write the term at all (because [latex]0[/latex] times anything is [latex]0[/latex], and adding [latex]0[/latex] does not change the value).
A term without a variable is called a constant term, and the degree of that term is [latex]0[/latex]. In the polynomial [latex]3x+13[/latex], we could have written the polynomial as [latex]3x^{1}+13x^{0}[/latex]. Although this is not how we would normally write this, it allows us to see that [latex]13[/latex] is the constant term because its degree is 0 and the degree of [latex]3x[/latex] is 1. The degree of this binomial is 1.
If a polynomial does not have a constant term, like in the polynomial [latex]14x^{3}+3x[/latex] we would say that the constant term is [latex]0[/latex].
Let’s see how this works by looking at several polynomials. We’ll take it step by step, starting with monomials, and then progressing to polynomials with more terms.
Remember: Any base written without an exponent has an implied exponent of [latex]1[/latex].
example
Find the degree of the following polynomials:
1. [latex]4x[/latex]
2. [latex]3{x}^{3}-5x+7[/latex]
3. [latex]-11[/latex]
4. [latex]-6{x}^{2}+9x - 3[/latex]
5. [latex]8x+2[/latex]
Working with polynomials is easier when you list the terms in descending order of degrees. When a polynomial is written this way, it is said to be in standard form. Look back at the polynomials in the previous example. Notice that they are all written in standard form. Get in the habit of writing the term with the highest degree first.
try it
Example
For the following polynomials, identify the degree, the leading term, and the leading coefficient.
- [latex]3+2{x}^{2}-4{x}^{3}[/latex]
- [latex]5{t}^{5}-2{t}^{3}+7t[/latex]
- [latex]6p-{p}^{3}-2[/latex]
In the following video, we will identify the terms, leading coefficient, and degree of a polynomial.
