Curved antennas, such as the ones shown in the photo, are commonly used to focus microwaves and radio waves to transmit television and telephone signals, as well as satellite and spacecraft communication. The cross-section of the antenna is in the shape of a parabola, which can be described by a quadratic function.

An array of satellite dishes. (credit: Matthew Colvin de Valle, Flickr)

Characteristics of Parabolas

The graph of a quadratic function is a U-shaped curve called a parabola. One important feature of the graph is that it has an extreme point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. In either case, the vertex is a turning point on the graph. The graph is also symmetric with a vertical line drawn through the vertex, called the axis of symmetry.

The [latex]y[/latex]-intercept is the point at which the parabola crosses the [latex]y[/latex]-axis. The [latex]x[/latex]-intercepts are the points at which the parabola crosses the [latex]x[/latex]-axis. If they exist, the [latex]x[/latex]-intercepts represent the zeros, or roots, of the quadratic function, the values of [latex]x[/latex] at which [latex]y=0[/latex].

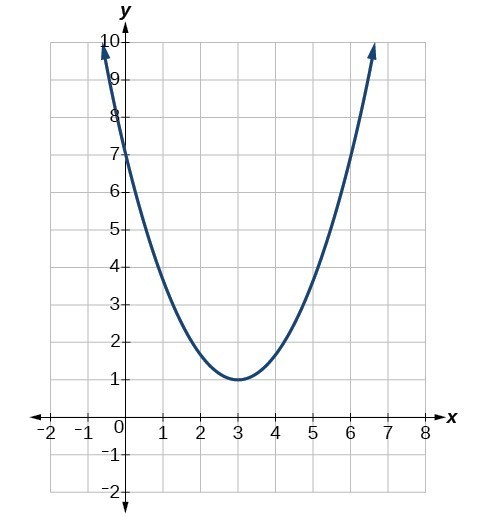
Example: Identifying the Characteristics of a Parabola

Determine the vertex, axis of symmetry, zeros, and y-intercept of the parabola shown below.

Show Solution

The vertex is the turning point of the graph. We can see that the vertex is at [latex](3,1)[/latex]. The axis of symmetry is the vertical line that intersects the parabola at the vertex. So the axis of symmetry is [latex]x=3[/latex]. This parabola does not cross the [latex]x[/latex]-axis, so it has no zeros. It crosses the [latex]y[/latex]-axis at (0, 7) so this is the [latex]y[/latex]-intercept.

Equations of Quadratic Functions

The general form of a quadratic function presents the function in the form

[latex]f\left(x\right)=a{x}^{2}+bx+c[/latex]

where [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are real numbers and [latex]a\ne 0[/latex]. If [latex]a>0[/latex], the parabola opens upward. If [latex]a<0[/latex], the parabola opens downward. We can use the general form of a parabola to find the equation for the axis of symmetry.

The axis of symmetry is defined by [latex]x=-\dfrac{b}{2a}[/latex]. If we use the quadratic formula, [latex]x=\dfrac{-b\pm \sqrt{{b}^{2}-4ac}}{2a}[/latex], to solve [latex]a{x}^{2}+bx+c=0[/latex] for the [latex]x[/latex]-intercepts, or zeros, we find the value of [latex]x[/latex] halfway between them is always [latex]x=-\dfrac{b}{2a}[/latex], the equation for the axis of symmetry.

The figure below shows the graph of the quadratic function written in general form as [latex]y={x}^{2}+4x+3[/latex]. In this form, [latex]a=1,\text{ }b=4[/latex], and [latex]c=3[/latex]. Because [latex]a>0[/latex], the parabola opens upward. The axis of symmetry is [latex]x=-\dfrac{4}{2\left(1\right)}=-2[/latex]. This also makes sense because we can see from the graph that the vertical line [latex]x=-2[/latex] divides the graph in half. The vertex always occurs along the axis of symmetry. For a parabola that opens upward, the vertex occurs at the lowest point on the graph, in this instance, [latex]\left(-2,-1\right)[/latex]. The [latex]x[/latex]-intercepts, those points where the parabola crosses the [latex]x[/latex]-axis, occur at [latex]\left(-3,0\right)[/latex] and [latex]\left(-1,0\right)[/latex].

The standard form of a quadratic function presents the function in the form

[latex]f\left(x\right)=a{\left(x-h\right)}^{2}+k[/latex]

where [latex]\left(h,\text{ }k\right)[/latex] is the vertex. Because the vertex appears in the standard form of the quadratic function, this form is also known as the vertex form of a quadratic function.

Given a quadratic function in general form, find the vertex of the parabola.

One reason we may want to identify the vertex of the parabola is that this point will inform us where the maximum or minimum value of the output occurs, [latex]k[/latex], and where it occurs, [latex]h[/latex]. If we are given the general form of a quadratic function:

[latex]f(x)=ax^2+bx+c[/latex]

We can define the vertex, [latex](h,k)[/latex], by doing the following:

• Identify [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex].
• Find [latex]h[/latex], the [latex]x[/latex]-coordinate of the vertex, by substituting [latex]a[/latex] and [latex]b[/latex] into [latex]h=-\dfrac{b}{2a}[/latex].
• Find [latex]k[/latex], the [latex]y[/latex]-coordinate of the vertex, by evaluating [latex]k=f\left(h\right)=f\left(-\dfrac{b}{2a}\right)[/latex]

Finding the Domain and Range of a Quadratic Function

Any number can be the input value of a quadratic function. Therefore the domain of any quadratic function is all real numbers. Because parabolas have a maximum or a minimum at the vertex, the range is restricted. Since the vertex of a parabola will be either a maximum or a minimum, the range will consist of all [latex]y[/latex]-values greater than or equal to the [latex]y[/latex]-coordinate of the vertex or less than or equal to the [latex]y[/latex]-coordinate at the turning point, depending on whether the parabola opens up or down.

Transformations of Quadratic Functions

The standard form of a quadratic function presents the function in the form

[latex]f\left(x\right)=a{\left(x-h\right)}^{2}+k[/latex]

where [latex]\left(h,k\right)[/latex] is the vertex. Because the vertex appears in the standard form of the quadratic function, this form is also known as the vertex form of a quadratic function.

The standard form is useful for determining how the graph is transformed from the graph of [latex]y={x}^{2}[/latex]. The figure below is the graph of this basic function.

Shift Up and Down by Changing the Value of [latex]k[/latex]

You can represent a vertical (up, down) shift of the graph of [latex]f(x)=x^2[/latex] by adding or subtracting a constant, [latex]k[/latex].

[latex]f(x)=x^2 + k[/latex]

If [latex]k>0[/latex], the graph shifts upward, whereas if [latex]k<0[/latex], the graph shifts downward.

 Shift left and right by changing the value of [latex]h[/latex]

You can represent a horizontal (left, right) shift of the graph of [latex]f(x)=x^2[/latex] by adding or subtracting a constant, [latex]h[/latex], to the variable [latex]x[/latex], before squaring.

[latex]f(x)=(x-h)^2 [/latex]

If [latex]h>0[/latex], the graph shifts toward the right and if [latex]h<0[/latex], the graph shifts to the left.

Stretch or compress by changing the value of [latex]a[/latex].

You can represent a stretch or compression (narrowing, widening) of the graph of [latex]f(x)=x^2[/latex] by multiplying the squared variable by a constant, [latex]a[/latex].

[latex]f(x)=ax^2 [/latex]

The magnitude of [latex]a[/latex] indicates the stretch of the graph. If [latex]|a|>1[/latex], the point associated with a particular [latex]x[/latex]-value shifts farther from the [latex]x[/latex]–axis, so the graph appears to become narrower, and there is a vertical stretch. But if [latex]|a|<1[/latex], the point associated with a particular [latex]x[/latex]-value shifts closer to the [latex]x[/latex]–axis, so the graph appears to become wider, but in fact there is a vertical compression.

The standard form and the general form are equivalent methods of describing the same function. We can see this by expanding out the general form and setting it equal to the standard form.

This is the [latex]x[/latex] coordinate of the vertexr and [latex]x=-\dfrac{b}{2a}[/latex] is the axis of symmetry we defined earlier. Setting the constant terms equal gives us:

In practice, though, it is usually easier to remember that [latex]k[/latex] is the output value of the function when the input is [latex]h[/latex], so [latex]f\left(h\right)=f\left(-\dfrac{b}{2a}\right)=k[/latex].

 Try It

A coordinate grid has been superimposed over the quadratic path of a basketball in the picture below. Find an equation for the path of the ball. Does the shooter make the basket?

(credit: modification of work by Dan Meyer)

Show Solution

The path passes through the origin and has vertex at [latex]\left(-4,\text{ }7\right)[/latex], so [latex]\left(h\right)x=-\frac{7}{16}{\left(x+4\right)}^{2}+7[/latex]. To make the shot, [latex]h\left(-7.5\right)[/latex] would need to be about 4 but [latex]h\left(-7.5\right)\approx 1.64[/latex]; he doesn’t make it.