Learning Outcomes
- Determine whether a function is one-to-one.
- Verify inverse functions.
- Determine the domain and range of an inverse function, and restrict the domain of a function to make it one-to-one.
- Find or evaluate the inverse of a function.
- Use the graph of a one-to-one function to graph its inverse function on the same axes.
A reversible heat pump is a climate-control system that is an air conditioner and a heater in a single device. Operated in one direction, it pumps heat out of a house to provide cooling. Operating in reverse, it pumps heat into the building from the outside, even in cool weather, to provide heating. As a heater, a heat pump is several times more efficient than conventional electrical resistance heating.
If some physical machines can run in two directions, we might ask whether some of the function “machines” we have been studying can also run backwards. Figure 1 provides a visual representation of this question. In this section, we will consider the reverse nature of functions.
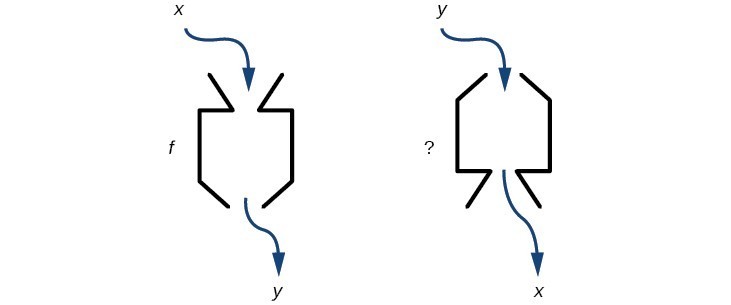
Figure 1. Can a function “machine” operate in reverse?
Determine whether a function is one-to-one
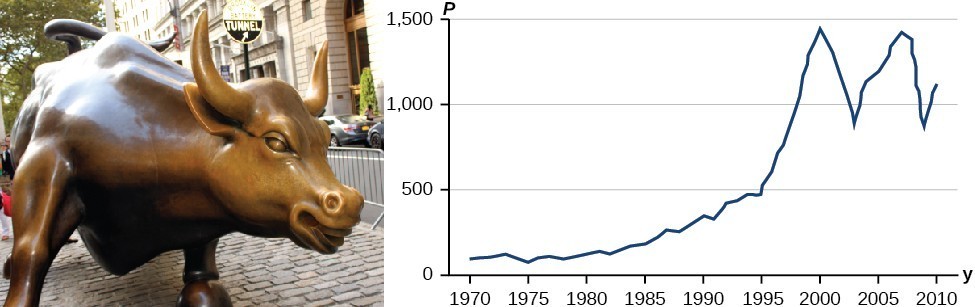
Some functions have a given output value that corresponds to two or more input values. For example, in the following stock chart the stock price was $1000 on five different dates, meaning that there were five different input values that all resulted in the same output value of $1000.
However, some functions have only one input value for each output value, as well as having only one output for each input. We call these functions one-to-one functions. As an example, consider a school that uses only letter grades and decimal equivalents, as listed in.
| Letter grade | Grade point average |
|---|---|
| A | 4.0 |
| B | 3.0 |
| C | 2.0 |
| D | 1.0 |
This grading system represents a one-to-one function, because each letter input yields one particular grade point average output and each grade point average corresponds to one input letter.
To visualize this concept, let’s look again at the two simple functions sketched in (a) and (b) below.
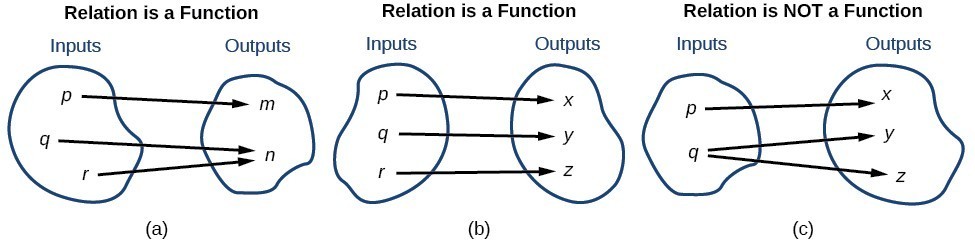
The function in part (a) shows a relationship that is not a one-to-one function because inputs [latex]q[/latex] and [latex]r[/latex] both give output [latex]n[/latex]. The function in part (b) shows a relationship that is a one-to-one function because each input is associated with a single output.
A General Note: One-to-One Function
A one-to-one function is a function in which each output value corresponds to exactly one input value.
Example: Determining Whether a Relationship Is a One-to-One Function
Is the area of a circle a function of its radius? If yes, is the function one-to-one?
Try It
- Is a balance a function of the bank account number?
- Is a bank account number a function of the balance?
- Is a balance a one-to-one function of the bank account number?
Evaluating and Solving Functions
When we have a function in formula form, it is usually a simple matter to evaluate the function. For example, the function [latex]f\left(x\right)=5 - 3{x}^{2}[/latex] can be evaluated by squaring the input value, multiplying by 3, and then subtracting the product from 5.
How To: EVALUATE A FUNCTION Given ITS FORMula.
- Replace the input variable in the formula with the value provided.
- Calculate the result.
Example: Evaluating Functions
Given the function [latex]h\left(p\right)={p}^{2}+2p[/latex], evaluate [latex]h\left(4\right)[/latex].
Example: Evaluating Functions at Specific Values
For the function, [latex]f\left(x\right)={x}^{2}+3x - 4[/latex], evaluate each of the following.
- [latex]f\left(2\right)[/latex]
- [latex]f(a)[/latex]
- [latex]f(a+h)[/latex]
- [latex]\dfrac{f\left(a+h\right)-f\left(a\right)}{h}[/latex]
Try It
Given the function [latex]g\left(m\right)=\sqrt{m - 4}[/latex], evaluate [latex]g\left(5\right)[/latex].
Example: Solving Functions
Given the function [latex]h\left(p\right)={p}^{2}+2p[/latex], solve for [latex]h\left(p\right)=3[/latex].
Try It
Given the function [latex]g\left(m\right)=\sqrt{m - 4}[/latex], solve [latex]g\left(m\right)=2[/latex].
Evaluating Functions Expressed in Formulas
Some functions are defined by mathematical rules or procedures expressed in equation form. If it is possible to express the function output with a formula involving the input quantity, then we can define a function in algebraic form. For example, the equation [latex]2n+6p=12[/latex] expresses a functional relationship between [latex]n[/latex] and [latex]p[/latex]. We can rewrite it to decide if [latex]p[/latex] is a function of [latex]n[/latex].
How To: Given a function in equation form, write its algebraic formula.
- Solve the equation to isolate the output variable on one side of the equal sign, with the other side as an expression that involves only the input variable.
- Use all the usual algebraic methods for solving equations, such as adding or subtracting the same quantity to or from both sides, or multiplying or dividing both sides of the equation by the same quantity.
Example: Finding an Equation of a Function
Express the relationship [latex]2n+6p=12[/latex] as a function [latex]p=f\left(n\right)[/latex], if possible.
Example: Expressing the Equation of a Circle as a Function
Does the equation [latex]{x}^{2}+{y}^{2}=1[/latex] represent a function with [latex]x[/latex] as input and [latex]y[/latex] as output? If so, express the relationship as a function [latex]y=f\left(x\right)[/latex].
Try It
If [latex]x - 8{y}^{3}=0[/latex], express [latex]y[/latex] as a function of [latex]x[/latex].
Q & A
Are there relationships expressed by an equation that do represent a function but which still cannot be represented by an algebraic formula?
Yes, this can happen. For example, given the equation [latex]x=y+{2}^{y}[/latex], if we want to express [latex]y[/latex] as a function of [latex]x[/latex], there is no simple algebraic formula involving only [latex]x[/latex] that equals [latex]y[/latex]. However, each [latex]x[/latex] does determine a unique value for [latex]y[/latex], and there are mathematical procedures by which [latex]y[/latex] can be found to any desired accuracy. In this case, we say that the equation gives an implicit (implied) rule for [latex]y[/latex] as a function of [latex]x[/latex], even though the formula cannot be written explicitly.
Evaluating a Function Given in Tabular Form
As we saw above, we can represent functions in tables. Conversely, we can use information in tables to write functions, and we can evaluate functions using the tables. For example, how well do our pets recall the fond memories we share with them? There is an urban legend that a goldfish has a memory of 3 seconds, but this is just a myth. Goldfish can remember up to 3 months, while the beta fish has a memory of up to 5 months. And while a puppy’s memory span is no longer than 30 seconds, the adult dog can remember for 5 minutes. This is meager compared to a cat, whose memory span lasts for 16 hours.
The function that relates the type of pet to the duration of its memory span is more easily visualized with the use of a table. See the table below.
| Pet | Memory span in hours |
|---|---|
| Puppy | 0.008 |
| Adult dog | 0.083 |
| Cat | 16 |
| Goldfish | 2160 |
| Beta fish | 3600 |
At times, evaluating a function in table form may be more useful than using equations. Here let us call the function [latex]P[/latex].
The domain of the function is the type of pet and the range is a real number representing the number of hours the pet’s memory span lasts. We can evaluate the function [latex]P[/latex] at the input value of “goldfish.” We would write [latex]P\left(\text{goldfish}\right)=2160[/latex]. Notice that, to evaluate the function in table form, we identify the input value and the corresponding output value from the pertinent row of the table. The tabular form for function [latex]P[/latex] seems ideally suited to this function, more so than writing it in paragraph or function form.
How To: Given a function represented by a table, identify specific output and input values.
- Find the given input in the row (or column) of input values.
- Identify the corresponding output value paired with that input value.
- Find the given output values in the row (or column) of output values, noting every time that output value appears.
- Identify the input value(s) corresponding to the given output value.
Example: Evaluating and Solving a Tabular Function
Using the table below,
- Evaluate [latex]g\left(3\right)[/latex].
- Solve [latex]g\left(n\right)=6[/latex].
| [latex]n[/latex] | 1 | 2 | 3 | 4 | 5 |
| [latex]g(n)[/latex] | 8 | 6 | 7 | 6 | 8 |
Try It
Using the table from the previous example, evaluate [latex]g\left(1\right)[/latex] .
Finding Function Values from a Graph
Evaluating a function using a graph also requires finding the corresponding output value for a given input value, only in this case, we find the output value by looking at the graph. Solving a function equation using a graph requires finding all instances of the given output value on the graph and observing the corresponding input value(s).
Example: Reading Function Values from a Graph
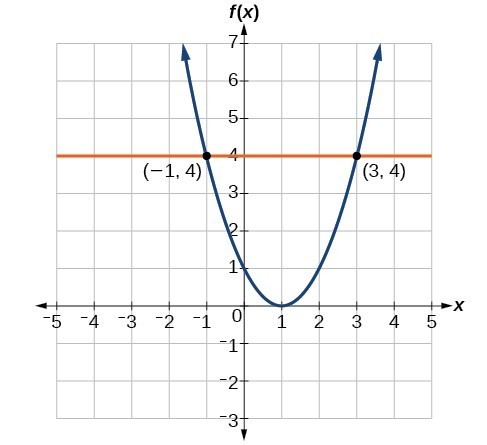
Given the graph below,
- Evaluate [latex]f\left(2\right)[/latex].
- Solve [latex]f\left(x\right)=4[/latex].
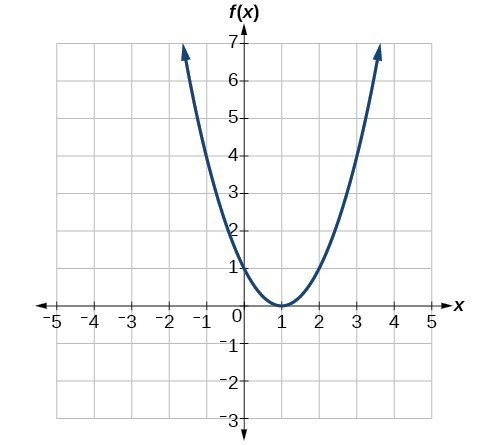
Try It
Using the graph, solve [latex]f\left(x\right)=1[/latex].

Identify Functions Using Graphs
As we have seen in examples above, we can represent a function using a graph. Graphs display many input-output pairs in a small space. The visual information they provide often makes relationships easier to understand. We typically construct graphs with the input values along the horizontal axis and the output values along the vertical axis.
The most common graphs name the input value [latex]x[/latex] and the output value [latex]y[/latex], and we say [latex]y[/latex] is a function of [latex]x[/latex], or [latex]y=f\left(x\right)[/latex] when the function is named [latex]f[/latex]. The graph of the function is the set of all points [latex]\left(x,y\right)[/latex] in the plane that satisfies the equation [latex]y=f\left(x\right)[/latex]. If the function is defined for only a few input values, then the graph of the function is only a few points, where the x-coordinate of each point is an input value and the y-coordinate of each point is the corresponding output value. For example, the black dots on the graph in the graph below tell us that [latex]f\left(0\right)=2[/latex] and [latex]f\left(6\right)=1[/latex]. However, the set of all points [latex]\left(x,y\right)[/latex] satisfying [latex]y=f\left(x\right)[/latex] is a curve. The curve shown includes [latex]\left(0,2\right)[/latex] and [latex]\left(6,1\right)[/latex] because the curve passes through those points.
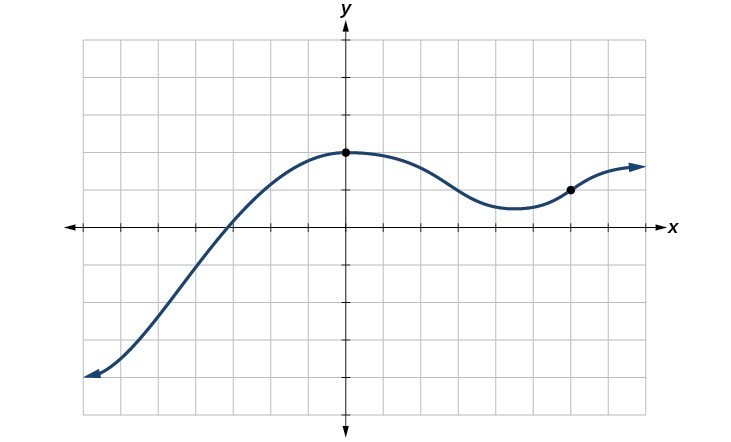
The vertical line test can be used to determine whether a graph represents a function. A vertical line includes all points with a particular [latex]x[/latex] value. The [latex]y[/latex] value of a point where a vertical line intersects a graph represents an output for that input [latex]x[/latex] value. If we can draw any vertical line that intersects a graph more than once, then the graph does not define a function because that [latex]x[/latex] value has more than one output. A function has only one output value for each input value.
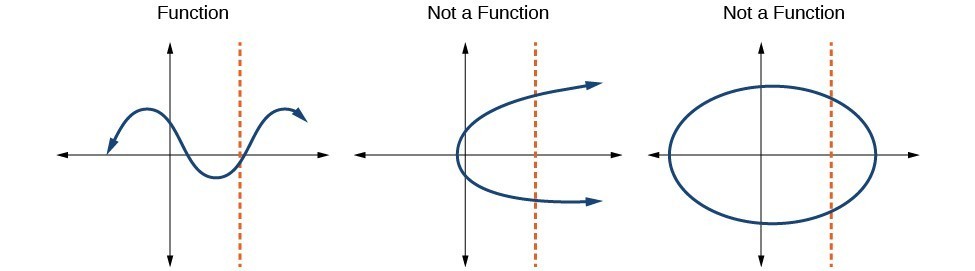
How To: Given a graph, use the vertical line test to determine if the graph represents a function.
- Inspect the graph to see if any vertical line drawn would intersect the curve more than once.
- If there is any such line, the graph does not represent a function.
- If no vertical line can intersect the curve more than once, the graph does represent a function.
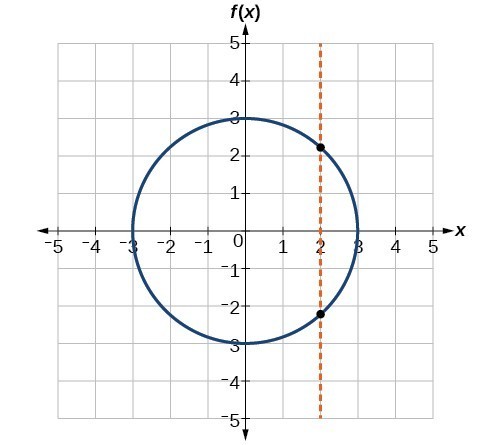
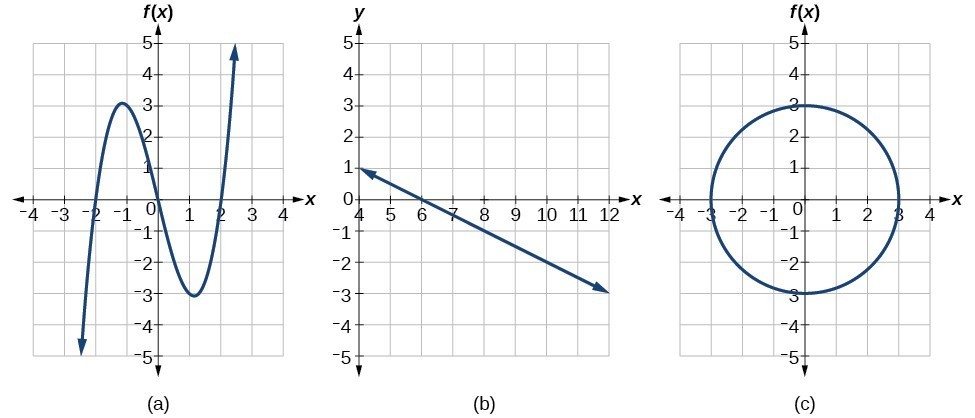
Example: Applying the Vertical Line Test
Which of the graphs represent(s) a function [latex]y=f\left(x\right)?[/latex]
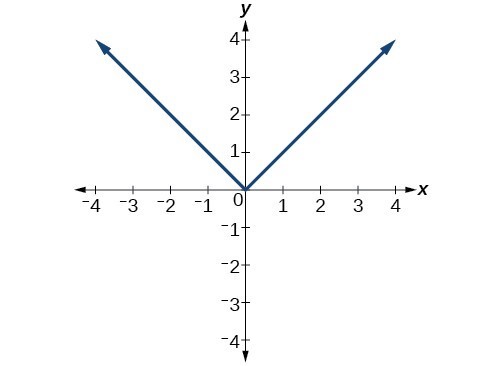
Try It
Does the graph below represent a function?
The Horizontal Line Test
Once we have determined that a graph defines a function, an easy way to determine if it is a one-to-one function is to use the horizontal line test. Draw horizontal lines through the graph. A horizontal line includes all points with a particular [latex]y[/latex] value. The [latex]x[/latex] value of a point where a vertical line intersects a function represents the input for that output [latex]y[/latex] value. If we can draw any horizontal line that intersects a graph more than once, then the graph does not represent a one-to-one function because that [latex]y[/latex] value has more than one input.
How To: Given a graph of a function, use the horizontal line test to determine if the graph represents a one-to-one function.
- Inspect the graph to see if any horizontal line drawn would intersect the curve more than once.
- If there is any such line, the function is not one-to-one.
- If no horizontal line can intersect the curve more than once, the function is one-to-one.
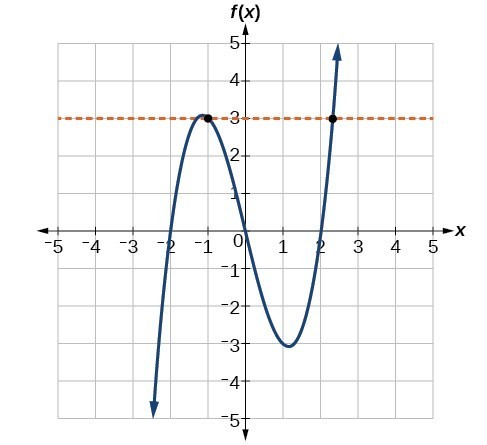
Example: Applying the Horizontal Line Test
Consider the functions (a), and (b)shown in the graphs below.

Are either of the functions one-to-one?
Characteristics of Inverse Functions
Suppose a fashion designer traveling to Milan for a fashion show wants to know what the temperature will be. He is not familiar with the Celsius scale. To get an idea of how temperature measurements are related, he asks his assistant, Betty, to convert 75 degrees Fahrenheit to degrees Celsius. She finds the formula [latex]C=\frac{5}{9}\left(F - 32\right)[/latex] and substitutes 75 for [latex]F[/latex] to calculate [latex]\frac{5}{9}\left(75 - 32\right)\approx {24}^{ \circ} {C}[/latex].
Knowing that a comfortable 75 degrees Fahrenheit is about 24 degrees Celsius, he sends his assistant the week’s weather forecast for Milan, and asks her to convert all of the temperatures to degrees Fahrenheit.
At first, Betty considers using the formula she has already found to complete the conversions. After all, she knows her algebra, and can easily solve the equation for [latex]F[/latex] after substituting a value for [latex]C[/latex]. For example, to convert 26 degrees Celsius, she could write
[latex]\begin{align}&26=\frac{5}{9}\left(F - 32\right) \\[1.5mm] &26\cdot \frac{9}{5}=F - 32 \\[1.5mm] &F=26\cdot \frac{9}{5}+32\approx 79 \end{align}[/latex]
After considering this option for a moment, however, she realizes that solving the equation for each of the temperatures will be awfully tedious. She realizes that since evaluation is easier than solving, it would be much more convenient to have a different formula, one that takes the Celsius temperature and outputs the Fahrenheit temperature.
The formula for which Betty is searching corresponds to the idea of an inverse function, which is a function for which the input of the original function becomes the output of the inverse function and the output of the original function becomes the input of the inverse function.
Given a function [latex]f\left(x\right)[/latex], we represent its inverse as [latex]{f}^{-1}\left(x\right)[/latex], read as “[latex]f[/latex] inverse of [latex]x[/latex].” The raised [latex]-1[/latex] is part of the notation. It is not an exponent; it does not imply a power of [latex]-1[/latex] . In other words, [latex]{f}^{-1}\left(x\right)[/latex] does not mean [latex]\frac{1}{f\left(x\right)}[/latex] because [latex]\frac{1}{f\left(x\right)}[/latex] is the reciprocal of [latex]f[/latex] and not the inverse.
The “exponent-like” notation comes from an analogy between function composition and multiplication: just as [latex]{a}^{-1}a=1[/latex] (1 is the identity element for multiplication) for any nonzero number [latex]a[/latex], so [latex]{f}^{-1}\circ f[/latex] equals the identity function, that is,
[latex]\left({f}^{-1}\circ f\right)\left(x\right)={f}^{-1}\left(f\left(x\right)\right)={f}^{-1}\left(y\right)=x[/latex]
This holds for all [latex]x[/latex] in the domain of [latex]f[/latex]. Informally, this means that inverse functions “undo” each other. However, just as zero does not have a reciprocal, some functions do not have inverses.
Given a function [latex]f\left(x\right)[/latex], we can verify whether some other function [latex]g\left(x\right)[/latex] is the inverse of [latex]f\left(x\right)[/latex] by checking whether either [latex]g\left(f\left(x\right)\right)=x[/latex] or [latex]f\left(g\left(x\right)\right)=x[/latex] is true. We can test whichever equation is more convenient to work with because they are logically equivalent (that is, if one is true, then so is the other.)
For example, [latex]y=4x[/latex] and [latex]y=\frac{1}{4}x[/latex] are inverse functions.
[latex]\left({f}^{-1}\circ f\right)\left(x\right)={f}^{-1}\left(4x\right)=\frac{1}{4}\left(4x\right)=x[/latex]
and
[latex]\left({f}^{}\circ {f}^{-1}\right)\left(x\right)=f\left(\frac{1}{4}x\right)=4\left(\frac{1}{4}x\right)=x[/latex]
A few coordinate pairs from the graph of the function [latex]y=4x[/latex] are (−2, −8), (0, 0), and (2, 8). A few coordinate pairs from the graph of the function [latex]y=\frac{1}{4}x[/latex] are (−8, −2), (0, 0), and (8, 2). If we interchange the input and output of each coordinate pair of a function, the interchanged coordinate pairs would appear on the graph of the inverse function.
A General Note: Inverse Function
For any one-to-one function [latex]f\left(x\right)=y[/latex], a function [latex]{f}^{-1}\left(x\right)[/latex] is an inverse function of [latex]f[/latex] if [latex]{f}^{-1}\left(y\right)=x[/latex]. This can also be written as [latex]{f}^{-1}\left(f\left(x\right)\right)=x[/latex] for all [latex]x[/latex] in the domain of [latex]f[/latex]. It also follows that [latex]f\left({f}^{-1}\left(x\right)\right)=x[/latex] for all [latex]x[/latex] in the domain of [latex]{f}^{-1}[/latex] if [latex]{f}^{-1}[/latex] is the inverse of [latex]f[/latex].
The notation [latex]{f}^{-1}[/latex] is read “[latex]f[/latex] inverse.” Like any other function, we can use any variable name as the input for [latex]{f}^{-1}[/latex], so we will often write [latex]{f}^{-1}\left(x\right)[/latex], which we read as [latex]"f[/latex] inverse of [latex]x[/latex]“.
Keep in mind that [latex]{f}^{-1}\left(x\right)\ne \frac{1}{f\left(x\right)}[/latex] and not all functions have inverses.
Example: Identifying an Inverse Function for a Given Input-Output Pair
If for a particular one-to-one function [latex]f\left(2\right)=4[/latex] and [latex]f\left(5\right)=12[/latex], what are the corresponding input and output values for the inverse function?
Try It
Given that [latex]{h}^{-1}\left(6\right)=2[/latex], what are the corresponding input and output values of the original function [latex]h?[/latex]
How To: Given two functions [latex]f\left(x\right)[/latex] and [latex]g\left(x\right)[/latex], test whether the functions are inverses of each other.
- Determine whether [latex]f\left(g\left(x\right)\right)=x[/latex] and [latex]g\left(f\left(x\right)\right)=x[/latex].
- If both statements are true, then [latex]g={f}^{-1}[/latex] and [latex]f={g}^{-1}[/latex]. If either statement is false, then [latex]g\ne {f}^{-1}[/latex] and [latex]f\ne {g}^{-1}[/latex].
Example: Testing Inverse Relationships Algebraically
If [latex]f\left(x\right)=\dfrac{1}{x+2}[/latex] and [latex]g\left(x\right)=\dfrac{1}{x}-2[/latex], is [latex]g={f}^{-1}?[/latex]
Try It
If [latex]f\left(x\right)={x}^{3}-4[/latex] and [latex]g\left(x\right)=\sqrt[3]{x+4}[/latex], is [latex]g={f}^{-1}?[/latex]
Example: Determining Inverse Relationships for Power Functions
If [latex]f\left(x\right)={x}^{3}[/latex] (the cube function) and [latex]g\left(x\right)=\frac{1}{3}x[/latex], is [latex]g={f}^{-1}?[/latex]
Try It
If [latex]f\left(x\right)={\left(x - 1\right)}^{3}\text{and}g\left(x\right)=\sqrt[3]{x}+1[/latex], is [latex]g={f}^{-1}?[/latex]
Determine the Domain and Range of an Inverse Function
The outputs of the function [latex]f[/latex] are the inputs to [latex]{f}^{-1}[/latex], so the range of [latex]f[/latex] is also the domain of [latex]{f}^{-1}[/latex]. Likewise, because the inputs to [latex]f[/latex] are the outputs of [latex]{f}^{-1}[/latex], the domain of [latex]f[/latex] is the range of [latex]{f}^{-1}[/latex]. We can visualize the situation.

Domain and range of a function and its inverse
When a function has no inverse function, it is possible to create a new function where that new function on a limited domain does have an inverse function. For example, the inverse of [latex]f\left(x\right)=\sqrt{x}[/latex] is [latex]{f}^{-1}\left(x\right)={x}^{2}[/latex], because a square “undoes” a square root; but the square is only the inverse of the square root on the domain [latex]\left[0,\infty \right)[/latex], since that is the range of [latex]f\left(x\right)=\sqrt{x}[/latex].
We can look at this problem from the other side, starting with the square (parent quadratic) function [latex]f\left(x\right)={x}^{2}[/latex]. If we want to construct an inverse to this function, we run into a problem, because for every given output of the quadratic function, there are two corresponding inputs (except when the input is 0). For example, the output 9 from the quadratic function corresponds to the inputs 3 and –3. But an output from a function is an input to its inverse; if this inverse input corresponds to more than one inverse output (input of the original function), then the “inverse” is not a function at all! To put it differently, the quadratic function is not a one-to-one function; it fails the horizontal line test, so it does not have an inverse function. In order for a function to have an inverse, it must be a one-to-one function.
In many cases, if a function is not one-to-one, we can still restrict the function to a part of its domain on which it is one-to-one. For example, we can make a restricted version of the square function [latex]f\left(x\right)={x}^{2}[/latex] with its range limited to [latex]\left[0,\infty \right)[/latex], which is a one-to-one function (it passes the horizontal line test) and which has an inverse (the square-root function).
If [latex]f\left(x\right)={\left(x - 1\right)}^{2}[/latex] on [latex]\left[1,\infty \right)[/latex], then the inverse function is [latex]{f}^{-1}\left(x\right)=\sqrt{x}+1[/latex].
- The domain of [latex]f[/latex] = range of [latex]{f}^{-1}[/latex] = [latex]\left[1,\infty \right)[/latex].
- The domain of [latex]{f}^{-1}[/latex] = range of [latex]f[/latex] = [latex]\left[0,\infty \right)[/latex].
Q & A
Is it possible for a function to have more than one inverse?
No. If two supposedly different functions, say, [latex]g[/latex] and [latex]h[/latex], both meet the definition of being inverses of another function [latex]f[/latex], then you can prove that [latex]g=h[/latex]. We have just seen that some functions only have inverses if we restrict the domain of the original function. In these cases, there may be more than one way to restrict the domain, leading to different inverses. However, on any one domain, the original function still has only one unique inverse.
A General Note: Domain and Range of Inverse Functions
The range of a function [latex]f\left(x\right)[/latex] is the domain of the inverse function [latex]{f}^{-1}\left(x\right)[/latex].
The domain of [latex]f\left(x\right)[/latex] is the range of [latex]{f}^{-1}\left(x\right)[/latex].
How To: Given a function, find the domain and range of its inverse.
- If the function is one-to-one, write the range of the original function as the domain of the inverse, and write the domain of the original function as the range of the inverse.
- If the domain of the original function needs to be restricted to make it one-to-one, then this restricted domain becomes the range of the inverse function.
Example: Finding the Inverses of PARENT Functions
Identify which of the parent functions besides the quadratic function are not one-to-one, and find a restricted domain on which each function is one-to-one, if any. The parent functions are reviewed below. We restrict the domain in such a fashion that the function assumes all y-values exactly once.
| Constant | Identity | Quadratic | Cubic | Reciprocal |
| [latex]f\left(x\right)=c[/latex] | [latex]f\left(x\right)=x[/latex] | [latex]f\left(x\right)={x}^{2}[/latex] | [latex]f\left(x\right)={x}^{3}[/latex] | [latex]f\left(x\right)=\frac{1}{x}[/latex] |
| Reciprocal squared | Cube root | Square root | Absolute value | |
| [latex]f\left(x\right)=\frac{1}{{x}^{2}}[/latex] | [latex]f\left(x\right)=\sqrt[3]{x}[/latex] | [latex]f\left(x\right)=\sqrt{x}[/latex] | [latex]f\left(x\right)=|x|[/latex] |
Try It
The domain of the function [latex]f[/latex] is [latex]\left(1,\infty \right)[/latex] and the range of the function [latex]f[/latex] is [latex]\left(\mathrm{-\infty },-2\right)[/latex]. Find the domain and range of the inverse function.
Define and Graph an Inverse
Once we have a one-to-one function, we can evaluate its inverse at specific inverse function inputs or construct a complete representation of the inverse function in many cases.
Inverting Tabular Functions
Suppose we want to find the inverse of a function represented in table form. Remember that the domain of a function is the range of the inverse and the range of the function is the domain of the inverse. So we need to interchange the domain and range.
Each row (or column) of inputs becomes the row (or column) of outputs for the inverse function. Similarly, each row (or column) of outputs becomes the row (or column) of inputs for the inverse function.
Example: Interpreting the Inverse of a Tabular Function
A function [latex]f\left(t\right)[/latex] is given below, showing distance in miles that a car has traveled in [latex]t[/latex] minutes. Find and interpret [latex]{f}^{-1}\left(70\right)[/latex].
| [latex]t\text{ (minutes)}[/latex] | 30 | 50 | 70 | 90 |
| [latex]f\left(t\right)\text{ (miles)}[/latex] | 20 | 40 | 60 | 70 |
Try It
Using the table below, find and interpret (a) [latex]\text{ }f\left(60\right)[/latex], and (b) [latex]\text{ }{f}^{-1}\left(60\right)[/latex].
| [latex]t\text{ (minutes)}[/latex] | 30 | 50 | 60 | 70 | 90 |
| [latex]f\left(t\right)\text{ (miles)}[/latex] | 20 | 40 | 50 | 60 | 70 |
Evaluating the Inverse of a Function, Given a Graph of the Original Function
The domain of a function can be read by observing the horizontal extent of its graph. We find the domain of the inverse function by observing the vertical extent of the graph of the original function, because this corresponds to the horizontal extent of the inverse function. Similarly, we find the range of the inverse function by observing the horizontal extent of the graph of the original function, as this is the vertical extent of the inverse function. If we want to evaluate an inverse function, we find its input within its domain, which is all or part of the vertical axis of the original function’s graph.
How To: Given the graph of a function, evaluate its inverse at specific points.
- Find the desired input of the inverse function on the [latex]y[/latex]-axis of the given graph.
- Read the inverse function’s output from the [latex]x[/latex]-axis of the given graph.
Example: Evaluating a Function and Its Inverse from a Graph at Specific Points
A function [latex]g\left(x\right)[/latex] is given below. Find [latex]g\left(3\right)[/latex] and [latex]{g}^{-1}\left(3\right)[/latex].

Try It
Using the graph in the previous example, (a) find [latex]{g}^{-1}\left(1\right)[/latex], and (b) estimate [latex]{g}^{-1}\left(4\right)[/latex].
Finding Inverses of Functions Represented by Formulas
Sometimes we will need to know an inverse function for all elements of its domain, not just a few. If the original function is given as a formula—for example, [latex]y[/latex] as a function of [latex]x-[/latex] we can often find the inverse function by solving to obtain [latex]x[/latex] as a function of [latex]y[/latex].
How To: Given a function represented by a formula, find the inverse.
- Verify that [latex]f[/latex] is a one-to-one function.
- Replace [latex]f\left(x\right)[/latex] with [latex]y[/latex].
- Interchange [latex]x[/latex] and [latex]y[/latex].
- Solve for [latex]y[/latex], and rename the function [latex]{f}^{-1}\left(x\right)[/latex].
Example: Inverting the Fahrenheit-to-Celsius Function
Find a formula for the inverse function that gives Fahrenheit temperature as a function of Celsius temperature.
[latex]C=\frac{5}{9}\left(F - 32\right)[/latex]
Try It
Solve for [latex]x[/latex] in terms of [latex]y[/latex] given [latex]y=\frac{1}{3}\left(x - 5\right)[/latex]
Example: Solving to Find an Inverse Function
Find the inverse of the function [latex]f\left(x\right)=\dfrac{2}{x - 3}+4[/latex].
Example: Solving to Find an Inverse with Radicals
Find the inverse of the function [latex]f\left(x\right)=2+\sqrt{x - 4}[/latex].
Try It
What is the inverse of the function [latex]f\left(x\right)=2-\sqrt{x}[/latex]? State the domains of both the function and the inverse function.
Use an online graphing tool to graph the function, its inverse, and [latex]f(x) = x[/latex] to check whether you are correct.
Graph a Function’s Inverse
Now that we can find the inverse of a function, we will explore the graphs of functions and their inverses. Let us return to the quadratic function [latex]f\left(x\right)={x}^{2}[/latex] restricted to the domain [latex]\left[0,\infty \right)[/latex], on which this function is one-to-one, and graph it as below.
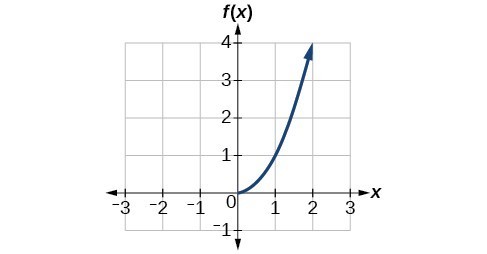
Quadratic function with domain restricted to [0, ∞).
Restricting the domain to [latex]\left[0,\infty \right)[/latex] makes the function one-to-one (it will obviously pass the horizontal line test), so it has an inverse on this restricted domain.
We already know that the inverse of the parent quadratic function is the square root function, that is, [latex]{f}^{-1}\left(x\right)=\sqrt{x}[/latex]. What happens if we graph both [latex]f\text{ }[/latex] and [latex]{f}^{-1}[/latex] on the same set of axes, using the [latex]x\text{-}[/latex] axis for the input to both [latex]f\text{ and }{f}^{-1}?[/latex]
We notice a distinct relationship: The graph of [latex]{f}^{-1}\left(x\right)[/latex] is the graph of [latex]f\left(x\right)[/latex] reflected about the diagonal line [latex]y=x[/latex], which we will call the identity line, shown below.
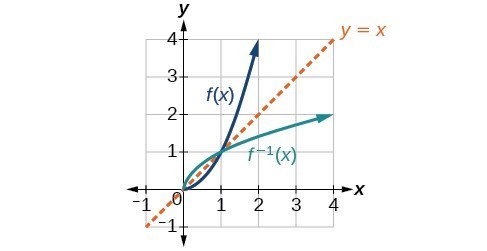
Square and square-root functions on the non-negative domain
This relationship will be observed for all one-to-one functions, because it is a result of the function and its inverse swapping inputs and outputs. This is equivalent to interchanging the roles of the vertical and horizontal axes.
Example: Finding the Inverse of a Function Using Reflection about the Identity Line
Given the graph of [latex]f\left(x\right)[/latex], sketch a graph of [latex]{f}^{-1}\left(x\right)[/latex].

Q & A
Is there any function that is equal to its own inverse?
Yes. If [latex]f={f}^{-1}[/latex], then [latex]f\left(f\left(x\right)\right)=x[/latex], and we can think of several functions that have this property. The identity function does, and so does the reciprocal function, because
[latex]\frac{1}{\frac{1}{x}}=x[/latex]
Any function [latex]f\left(x\right)=c-x[/latex], where [latex]c[/latex] is a constant, is also equal to its own inverse.
Key Concepts
- A graph represents a one-to-one function if any horizontal line drawn on the graph intersects the graph at no more than one point.
- If [latex]g\left(x\right)[/latex] is the inverse of [latex]f\left(x\right)[/latex], then [latex]g\left(f\left(x\right)\right)=f\left(g\left(x\right)\right)=x[/latex].
- Each of the parent functions, except [latex]y=c[/latex] has an inverse. Some need a restricted domain.
- For a function to have an inverse, it must be one-to-one (pass the horizontal line test).
- A function that is not one-to-one over its entire domain may be one-to-one on part of its domain.
- For a tabular function, exchange the input and output rows to obtain the inverse.
- The inverse of a function can be determined at specific points on its graph.
- To find the inverse of a function [latex]y=f\left(x\right)[/latex], switch the variables [latex]x[/latex] and [latex]y[/latex]. Then solve for [latex]y[/latex] as a function of [latex]x[/latex].
- The graph of an inverse function is the reflection of the graph of the original function across the line [latex]y=x[/latex].
Glossary
- horizontal line test
- a method of testing whether a function is one-to-one by determining whether any horizontal line intersects the graph more than once
- independent variable
- an input variable
- inverse function
- for any one-to-one function [latex]f\left(x\right)[/latex], the inverse is a function [latex]{f}^{-1}\left(x\right)[/latex] such that [latex]{f}^{-1}\left(f\left(x\right)\right)=x[/latex] for all [latex]x[/latex] in the domain of [latex]f[/latex]; this also implies that [latex]f\left({f}^{-1}\left(x\right)\right)=x[/latex] for all [latex]x[/latex] in the domain of [latex]{f}^{-1}[/latex]
- one-to-one function
- a function for which each value of the output is associated with a unique input value
Candela Citations
- Revision and Adaptation. Provided by: Lumen Learning. License: CC BY: Attribution
- College Algebra. Authored by: Abramson, Jay et al.. Provided by: OpenStax. Located at: http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2. License: CC BY: Attribution. License Terms: Download for free at http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2
- Ex: Find an Inverse Function From a Table. Authored by: Mathispower4u. Located at: https://youtu.be/TSztRfzmk0M. License: All Rights Reserved. License Terms: Standard YouTube License