Learning Outcomes
- Multiply two or more fractions
- Find the reciprocal of a fraction
- Divide fractions
Key words
- Reciprocal: the inversion of a fraction
- Cancelling: dividing the numerator and denominator by the same common factor
Multiplication
A model may help us to understand multiplication of fractions. We will use fraction tiles to model [latex]\frac{1}{2}\cdot \frac{3}{4}[/latex]. To multiply [latex]\frac{1}{2}[/latex] and [latex]\frac{3}{4}[/latex], think [latex]\frac{1}{2}[/latex] of [latex]\frac{3}{4}[/latex].
Start with fraction tiles for three-fourths. To find one-half of three-fourths, we need to divide them into two equal groups. Since we cannot divide the three [latex]\frac{1}{4}[/latex] tiles evenly into two parts, we use equivalent smaller tiles.

We see [latex]\frac{6}{8}[/latex] is equivalent to [latex]\frac{3}{4}[/latex]. Taking half of the six [latex]\frac{1}{8}[/latex] tiles gives us three [latex]\frac{1}{8}[/latex] tiles, which is [latex]\frac{3}{8}[/latex].
Therefore,
[latex]\frac{1}{2}\cdot \frac{3}{4}=\frac{3}{8}[/latex]
Example
Use a diagram to model [latex]\frac{1}{2}\cdot \frac{3}{4}[/latex]
Solution:
First shade in [latex]\frac{3}{4}[/latex] of the rectangle.

We will take [latex]\frac{1}{2}[/latex] of this [latex]\frac{3}{4}[/latex], so we heavily shade [latex]\Large\frac{1}{2}[/latex] of the shaded region.

Notice that [latex]3[/latex] out of the [latex]8[/latex] pieces are heavily shaded. This means that [latex]\frac{3}{8}[/latex] of the rectangle is heavily shaded.
Therefore, [latex]\frac{1}{2}[/latex] of [latex]\frac{3}{4}[/latex] is [latex]\frac{3}{8}[/latex], or [latex]{\frac{1}{2}\cdot \frac{3}{4}}={\frac{3}{8}}[/latex].
Look at the result we got from the model in the example above. We found that [latex]\frac{1}{2}\cdot \frac{3}{4}=\frac{3}{8}[/latex]. Do you notice that we could have gotten the same answer by multiplying the numerators and multiplying the denominators?
| [latex]\frac{1}{2}\cdot \frac{3}{4}[/latex] | |
| Multiply the numerators, and multiply the denominators. | [latex]\frac{1\cdot 3}{2\cdot 4}[/latex] |
| Simplify. | [latex]\frac{3}{8}[/latex] |
This leads to the definition of fraction multiplication. To multiply fractions, we multiply the numerators and multiply the denominators. Then we write the fraction in simplified form.
Fraction Multiplication
If [latex]a,b,c,\text{ and }d[/latex] are integers where [latex]b\ne 0\text{ and }d\ne 0[/latex], then,
[latex]\frac{a}{b}\cdot \frac{c}{d}=\frac{ac}{bd}[/latex]
Notice that [latex]b, d\ne 0[/latex]. This because we cannot divide by [latex]0[/latex], so if [latex]b[/latex] or [latex]d=0[/latex] then the fractions [latex]\frac{a}{b}[/latex] or [latex]\frac{c}{d}[/latex] would be undefined.
Example
Multiply, and write the answer in simplified form: [latex]\frac{3}{4}\cdot \frac{1}{5}[/latex]
Try It
Multiply, and write the answer in simplified form: [latex]\frac{6}{5}\cdot \frac{1}{8}[/latex]
The following video provides more examples of how to multiply fractions, and simplify the result.
To multiply more than two fractions, we have a similar definition. We still multiply the numerators and multiply the denominators. Then we write the fraction in simplified form.
Multiplying More Than Two Fractions
If [latex]a,b,c,d,e \text{ and }f[/latex] are numbers where [latex]b\ne 0,d\ne 0\text{ and }f\ne 0[/latex], then
[latex]\frac{a}{b}\cdot\frac{c}{d}\cdot\frac{e}{f}=\frac{a\cdot c\cdot e}{b\cdot d\cdot f}[/latex]
Think About It
Multiply [latex]\frac{2}{3}\cdot\frac{1}{4}\cdot\frac{3}{5}[/latex]. Simplify the answer.
What makes this example different than the previous ones? Use the box below to write down a few thoughts about how you would multiply three fractions together.
This technique of dividing out a number on the numerator with the same number on the denominator is often referred to as cancelling.
When multiplying fractions, the properties of positive and negative numbers still apply. It is a good idea to determine the sign of the product as the first step. In the next example, we will multiply two negatives, so the product will be positive.
Example
Multiply, and write the answer in simplified form: [latex]-\frac{5}{8}\left(-\frac{2}{3}\right)[/latex]
Try it
Example
Multiply, and write the answer in simplified form: [latex]-\frac{14}{15}\cdot \frac{20}{21}[/latex]
Try it
The following video shows another example of multiplying fractions that are negative.
When multiplying a fraction by a whole number, it may be helpful to write the whole number as a fraction. Any whole number, [latex]a[/latex], can be written as [latex]\frac{a}{1}[/latex]. For example, [latex]3=\frac{3}{1}[/latex].
example
Multiply, and write the answer in simplified form:
- [latex]{\frac{1}{7}}\cdot 56[/latex]
- [latex]{\frac{12}{5}}\left(-20\right)[/latex]
Try it
Multiply, and write the answer in simplified form: [latex]-\frac{4}{15}\cdot 20[/latex]
Watch the following video to see more examples of how to multiply a fraction and a whole number.
Finding the Reciprocal of a Number
The fractions [latex]\frac{2}{3}[/latex] and [latex]\frac{3}{2}[/latex] are related to each other in a special way. So are [latex]-\frac{10}{7}[/latex] and [latex]-\frac{7}{10}[/latex]. Do you see how? Besides looking like upside-down versions of one another, if we were to multiply these pairs of fractions, the product would be [latex]1[/latex].
[latex]\frac{2}{3}\cdot \frac{3}{2}=1\text{ and }-\frac{10}{7}\left(-\frac{7}{10}\right)=1[/latex]
Such pairs of numbers are called reciprocals.
Reciprocal
The reciprocal of the fraction [latex]\frac{a}{b}[/latex] is [latex]\frac{b}{a}[/latex], where [latex]a\ne 0[/latex] and [latex]b\ne 0[/latex].
A number and its reciprocal have a product of [latex]1[/latex].
[latex]\frac{a}{b}\cdot \frac{b}{a}=1[/latex]
Here are some examples of reciprocals:
| Original number | Reciprocal | Product |
|---|---|---|
| [latex]\dfrac{3}{4}[/latex] | [latex]\dfrac{4}{3}[/latex] | [latex]\dfrac{3}{4}\cdot\dfrac{4}{3}=\dfrac{3\cdot 4}{4\cdot 3}=\dfrac{12}{12}=1[/latex] |
| [latex]\dfrac{1}{2}[/latex] | [latex]\dfrac{2}{1}[/latex] | [latex]\dfrac{1}{2}\cdot\dfrac{2}{1}=\dfrac{1\cdot2}{2\cdot1}=\dfrac{2}{2}=1[/latex] |
| [latex]3=\dfrac{3}{1}[/latex] | [latex]\dfrac{1}{3}[/latex] | [latex]\dfrac{3}{1}\cdot\dfrac{1}{3}=\dfrac{3\cdot 1}{1\cdot 3}=\dfrac{3}{3}=1[/latex] |
| [latex]\dfrac{7}{3}[/latex] | [latex]\dfrac{3}{7}[/latex] | [latex]\dfrac{7}{3}\cdot\dfrac{3}{7}=\dfrac{7\cdot3}{3\cdot7}=\dfrac{21}{21}= 1[/latex] |
To find the reciprocal of a fraction, we invert the fraction. This means that we place the numerator in the denominator and the denominator in the numerator. You can think of it as switching the numerator and denominator: swap the [latex]2[/latex] with the [latex]5[/latex] in [latex]\dfrac{2}{5}[/latex] to get the reciprocal [latex]\dfrac{5}{2}[/latex].
Make sure that if it’s a negative fraction, the reciprocal is also negative. This is because the product of two negative numbers will give you the positive one that you are looking for. To get a positive result when multiplying two numbers, the numbers must have the same sign. So reciprocals must have the same sign.

To find the reciprocal, keep the same sign and invert the fraction.
Example
Find the reciprocal of each number. Then check that the product of each number and its reciprocal is [latex]1[/latex].
- [latex]\frac{4}{9}[/latex]
- [latex]-\frac{1}{6}[/latex]
- [latex]-\frac{14}{5}[/latex]
- [latex]7[/latex]
Solution:
To find the reciprocals, we keep the sign and invert the fractions.
| 1. | |
| Find the reciprocal of [latex]\frac{4}{9}[/latex] | The reciprocal of [latex]\frac{4}{9}[/latex] is [latex]\frac{9}{4}[/latex] |
| Check: | |
| Multiply the number and its reciprocal. | [latex]\frac{4}{9}\cdot \frac{9}{4}[/latex] |
| Multiply numerators and denominators. | [latex]\frac{36}{36}[/latex] |
| Simplify. | [latex]1\quad\checkmark[/latex] |
| 2. | |
| Find the reciprocal of [latex]-\frac{1}{6}[/latex] | [latex]-\frac{6}{1}[/latex] |
| Simplify. | [latex]-6[/latex] |
| Check: | [latex]-\frac{1}{6}\normalsize\cdot \left(-6\right)[/latex] |
| [latex]1\quad\checkmark[/latex] |
| 3. | |
| Find the reciprocal of [latex]-\frac{14}{5}[/latex] | [latex]-\frac{5}{14}[/latex] |
| Check: | [latex]-\frac{14}{5}\cdot \left(-\frac{5}{14}\right)[/latex] |
| [latex]\frac{70}{70}[/latex] | |
| [latex]1\quad\checkmark[/latex] |
| 4. | |
| Find the reciprocal of [latex]7[/latex] | |
| Write [latex]7[/latex] as a fraction. | [latex]\frac{7}{1}[/latex] |
| Write the reciprocal of [latex]\frac{7}{1}[/latex] | [latex]\frac{1}{7}[/latex] |
| Check: | [latex]7\cdot\left(\frac{1}{7}\right)[/latex] |
| [latex]1\quad\checkmark[/latex] |
Try It
 Caution! Division by zero is undefined and so is the reciprocal of any fraction that has a zero in the numerator. For any real number a, [latex]\dfrac{a}{0}[/latex] is undefined. Additionally, the reciprocal of [latex]\dfrac{0}{a}[/latex] will always be undefined.
Caution! Division by zero is undefined and so is the reciprocal of any fraction that has a zero in the numerator. For any real number a, [latex]\dfrac{a}{0}[/latex] is undefined. Additionally, the reciprocal of [latex]\dfrac{0}{a}[/latex] will always be undefined.Division by Zero
You know what it means to divide by [latex]2[/latex] or divide by [latex]10[/latex], but what does it mean to divide a quantity by [latex]0[/latex]? Is this even possible? On the flip side, can you divide [latex]0[/latex] by a number? Consider the fraction
[latex]\dfrac{0}{8}[/latex]
We can read it as, “zero divided by eight.” Since multiplication is the inverse of division, we could rewrite this as a multiplication problem. What number times [latex]8[/latex] equals [latex]0[/latex]?
[latex]\text{?}\cdot{8}=0[/latex]
We can infer that the unknown must be [latex]0[/latex] since that is the only number that will give a result of [latex]0[/latex] when it is multiplied by [latex]8[/latex].
Now let’s consider the reciprocal of [latex]\dfrac{0}{8}[/latex] which would be [latex]\dfrac{8}{0}[/latex]. If we rewrite this as a multiplication problem, we will have “what times [latex]0[/latex] equals [latex]8[/latex]?”
[latex]\text{?}\cdot{0}=8[/latex]
This doesn’t make any sense. There are no numbers that you can multiply by zero to get a result of 8. In fact, any number divided by [latex]0[/latex] is impossible, or better stated, all division by zero is undefined.
Divide Fractions
There are times when you need to use division to solve a problem. For example, if painting one coat of paint on the walls of a room requires [latex]3[/latex] quarts of paint and you have a bucket that contains [latex]6[/latex] quarts of paint, how many coats of paint can you paint on the walls? You divide [latex]6[/latex] by [latex]3[/latex] for an answer of [latex]2[/latex] coats. There will also be times when you need to divide by a fraction. Suppose painting a closet with one coat only required [latex]\dfrac{1}{2}[/latex] quart of paint. How many coats could be painted with the 6 quarts of paint? To find the answer, you need to divide [latex]6[/latex] by the fraction, [latex]\dfrac{1}{2}[/latex].
Dividing is Multiplying by the Reciprocal
For all division, you can turn the operation into multiplication by using the reciprocal. Dividing is the same as multiplying by the reciprocal.
If you have a recipe that needs to be divided in half, you can divide each ingredient by [latex]2[/latex], or you can multiply each ingredient by [latex]\dfrac{1}{2}[/latex] to find the new amount.
If you have [latex]\dfrac{3}{4}[/latex] of a candy bar and need to divide it among [latex]5[/latex] people, each person gets [latex]\dfrac{1}{5}[/latex] of the available candy:
[latex]\dfrac{1}{5}\text{ of }\dfrac{3}{4}=\dfrac{1}{5}\cdot\dfrac{3}{4}=\dfrac{3}{20}[/latex]
Each person gets [latex]\dfrac{3}{20}[/latex] of a whole candy bar.
If you have [latex]\dfrac{3}{2}[/latex] of a pizza left over, how can you divide what is left (the red shaded region) among [latex]6[/latex] people fairly?
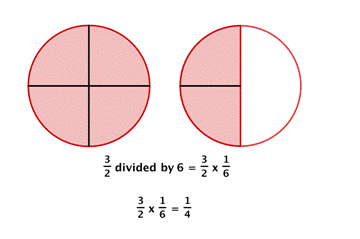
Each person gets one piece, so each person gets [latex]\dfrac{1}{4}[/latex] of a pizza.
Divide a Whole Number by a Fraction
Let’s use money to model [latex]2\div\frac{1}{4}[/latex]. We often read [latex]\frac{1}{4}[/latex] as a ‘quarter’, and we know that a quarter is one-fourth of a dollar as shown in the image below. So we can think of [latex]2\div\frac{1}{4}[/latex] as, “How many quarters are there in two dollars?” One dollar is [latex]4[/latex] quarters, so [latex]2[/latex] dollars would be [latex]8[/latex] quarters. So again, [latex]2\div\dfrac{1}{4}=\dfrac{2}{1}\cdot\dfrac{4}{1}=8[/latex].
Let’s look at another way to model [latex]2\div\frac{1}{4}[/latex].
Example
Divide: [latex]2\div\frac{1}{4}[/latex]
Try It
Divide: [latex]2\div\frac{1}{3}[/latex]
Divide: [latex]3\div\frac{1}{2}[/latex]
Try It
The next video shows more examples of how to divide a whole number by a fraction.
Example
Divide. [latex]9\div\dfrac{1}{2}[/latex]
Divide a Fraction by a Fraction
Sometimes we need to solve a problem that requires dividing a fraction by a fraction. Suppose we want to find the quotient: [latex]\frac{1}{2}\div\frac{1}{6}[/latex]. We need to figure out how many [latex]\frac{1}{6}\text{s}[/latex] there are in [latex]\frac{1}{2}[/latex]. We can use fraction tiles to model this division. We start by lining up the half and sixth fraction tiles as shown below. Notice, there are three [latex]\frac{1}{6}[/latex] tiles in [latex]\frac{1}{2}[/latex], so [latex]\frac{1}{2}\div\frac{1}{6}=3[/latex].

Example
Model: [latex]\frac{1}{4}\div\frac{1}{8}[/latex]
Solution:
We want to determine how many [latex]\frac{1}{8}\text{s}[/latex] are in [latex]\frac{1}{4}[/latex]. Start with one [latex]\frac{1}{4}[/latex] tile. Line up [latex]\frac{1}{8}[/latex] tiles underneath the [latex]\frac{1}{4}[/latex] tile.

There are two [latex]\frac{1}{8}[/latex]s in [latex]\frac{1}{4}[/latex].
So, [latex]\frac{1}{4}\div\frac{1}{8}=2[/latex].
Try It
Model: [latex]\frac{1}{3}\div\frac{1}{6}[/latex]
Model: [latex]\frac{1}{2}\div\frac{1}{4}[/latex]
The following video shows another way to model division of two fractions.
Using fraction tiles, we showed that [latex]\frac{1}{2}\div\frac{1}{6}=3[/latex]. Notice that [latex]\frac{1}{2}\cdot \frac{6}{1}=3[/latex] also. How are [latex]\frac{1}{6}[/latex] and [latex]\frac{6}{1}[/latex] related? They are reciprocals. This leads us to the procedure for fraction division. Suppose we have a pizza that is already cut into [latex]4[/latex] slices. How many [latex]\dfrac{1}{2}[/latex] slices are there?
 |
 |
There are [latex]8[/latex] slices. You can see that dividing [latex]4[/latex] by [latex]\dfrac{1}{2}[/latex] gives the same result as multiplying [latex]4[/latex] by [latex]2[/latex].
What would happen if you needed to divide each slice into thirds?

You would have [latex]12[/latex] slices, which is the same as multiplying [latex]4[/latex] by [latex]3[/latex].
Fraction Division
If [latex]a,b,c,\text{ and }d[/latex] are numbers where [latex]b\ne 0,c\ne 0,\text{ and }d\ne 0[/latex], then
[latex]\frac{a}{b}\div\frac{c}{d}=\frac{a}{b}\cdot \frac{d}{c}[/latex]
To divide fractions, multiply the first fraction by the reciprocal of the second.
We need to say [latex]b\ne 0,c\ne 0\text{ and }d\ne 0[/latex] to be sure we don’t divide by zero.
Dividing with Fractions
- Find the reciprocal of the divisor (the number that follows the division symbol).
- Multiply the dividend (the number before the division symbol) by the reciprocal of the divisor (the number after the division symbol).
Any easy way to remember how to divide fractions is the phrase “keep, change, flip.” This means to KEEP the first number, CHANGE the division sign to multiplication, and then FLIP (use the reciprocal) of the second number.
Example
Divide [latex]\dfrac{2}{3}\div\dfrac{1}{6}[/latex]
Example
Divide [latex]-\dfrac{3}{5}\div\dfrac{2}{3}[/latex]
When solving a division problem by multiplying by the reciprocal, remember to write all whole numbers as improper fractions before doing calculations [latex](\text{i.e. } 5=\dfrac{5}{1}[/latex].
Try It
Example
Divide, and write the answer in simplified form: [latex]\frac{2}{5}\div\left(-\frac{3}{7}\right)[/latex]
Example
Divide, and write the answer in simplified form: [latex]-\frac{3}{4}\div\left(-\frac{7}{8}\right)[/latex]
Try It
The following video shows more examples of dividing fractions that are negative.





