Learning Outcomes
- Use the elimination method without multiplication
- Express the solution of an inconsistent system of equations containing two variables
- Express the solution of a dependent system of equations containing two variables
Key words
- addition property of equality: adding the same term to both sides of an equation results in an equivalent equation
- elimination method: a method that uses the addition property of equality to solve a system of linear equations
- opposites: two terms that are identical in absolute value but opposite in sign
- coefficient: the number multiplied onto a variable
The Elimination Method
The elimination method for solving systems of linear equations uses the addition property of equality: adding the same term to both sides of an equation results in an equivalent equation. This can be extended to: adding two equations together results in an equivalent equation. This is true because if [latex]A=B[/latex] and [latex]C=D[/latex], then adding [latex]C[/latex] to both sides of the first equation is equivalent to adding [latex]D[/latex] to both sides of the first equation, since [latex]C=D[/latex]. That means that [latex]A+C=B+C[/latex] and [latex]A+D=B+D[/latex]. Consequently, [latex]A+C=B+D[/latex] is also an equivalent equation since [latex]C=D[/latex].
For example, if [latex]x+y=3\\x-y=5[/latex] then adding the equations together gives [latex]2x=8[/latex], which is an equivalent equation to both [latex]x+y=3[/latex] and [latex]x-y=5[/latex]. In addition, adding the two equations together eliminated the [latex]y[/latex]-terms since they were opposites, and their sum is zero. This results in a linear equation in one variable [latex]2x=8[/latex], which can be solved to [latex]x=4[/latex]. Now that we know the value of the [latex]x[/latex]-variable, we can substitute its value into either of the original equations to find [latex]y[/latex]: [latex]x+y=3\\4+y=3\\y=-1[/latex] Consequently the solution of the system is [latex](4,-1)[/latex].
This solution must also satisfy the other equation [latex]x-y=5[/latex], which it does: [latex]4-(-1)=5[/latex].
In order for the elimination method to work, one of the variables must be eliminated when the equations are added together. This requires that the coefficients of one of the variables are opposites.
Example
Use elimination to solve the system.
[latex]\begin{equation}\begin{aligned}x–y & =−6\\x+y & =8\end{aligned}\end{equation}[/latex]
Solution
The [latex]y[/latex] terms have opposite coefficients of [latex]-1[/latex] and [latex]1[/latex], which add to zero.
Add the equations:
[latex]\begin{equation}\begin{aligned}x-y &=-6\\ x+y & =8\\ \\2x +0y& =2\end{aligned}\end{equation}[/latex]
Solve for [latex]x[/latex]:
[latex]\begin{equation}\begin{aligned}2x &=2\\x &=1\end{aligned}\end{equation}[/latex]
Substitute [latex]x=1[/latex] into one of the original equations and solve for [latex]y[/latex]:
[latex]\begin{equation}\begin{aligned}x+y &=8\\1+y &=8\\y&=8–1\\y&=7\end{aligned}\end{equation}[/latex]
Since we used the second equation to find [latex]x[/latex], we can check the solution in the first equation:
[latex]\begin{equation}\begin{aligned}x–y&=−6\\1–7&=−6\\−6&=−6\end{aligned}\end{equation}[/latex]
The answer checks.
Answer
The solution to this system is [latex]\left(1,7\right)[/latex].
Example
Solve the given system of equations by elimination.
Solution
The [latex]x[/latex] terms have opposite coefficients of [latex]1[/latex] and [latex]-1[/latex], which add to zero.
Add the two equations together to eliminate [latex]x[/latex]:
Now that we have eliminated [latex]x[/latex], we can solve the resulting equation for [latex]y[/latex]:
[latex]\begin{equation}\begin{aligned}3y&=2 \\ y&=\frac{2}{3}\end{aligned}\end{equation}[/latex]
Then, we substitute this value for [latex]y[/latex] into one of the original equations and solve for [latex]x[/latex]:
[latex]\begin{equation}\begin{aligned}-x+y&=3 \\ -x+\frac{2}{3}&=3\\-x&=3-\dfrac{2}{3} \\ -x&=\frac{7}{3} \\ x&=-\frac{7}{3}\end{aligned}\end{equation}[/latex]
The solution to this system is [latex]\left(-\dfrac{7}{3},\dfrac{2}{3}\right)[/latex].
Since we used the second equation to find [latex]x[/latex], we can check the solution in the first equation:
[latex]\begin{equation}\begin{aligned}x+2y&=-1\\ \left(-\dfrac{7}{3}\right)+2\left(\dfrac{2}{3}\right)&=-1 \\ -\dfrac{7}{3}+\dfrac{4}{3}&=-1\\ \text{ }-\dfrac{3}{3}&=-1\\ -1&=-1\;\;\;\;\; \text{True}\end{aligned}\end{equation}[/latex]
Answer
The solution to this system is [latex]\left(-\dfrac{7}{3},\dfrac{2}{3}\right)[/latex].
We gain an important perspective on systems of equations by looking at the graphical representation. In the graph below, we see that the equations intersect at the solution. We do not need to ask whether there may be a second solution, because observing the graph confirms that the system has exactly one solution.
Try It
Solve the given system of equations by elimination.
Try It
Solve the given system of equations by elimination.
Unfortunately not all systems work out this nicely. How about a system like [latex]2x+y=12[/latex] and [latex]−3x+y=2[/latex]. If we add these two equations together, no variables are eliminated: [latex]-x+2y=14[/latex]
But we need to eliminate a variable for this method to work. Each equation has an [/latex]x[/latex] variable with a coefficient of [latex]1[/latex], so if we were to subtract the equations[/latex]x[/latex] would be eliminated. Subtraction is equivalent to adding the opposite, so we add the opposite of one of the equations to the other equation. This means multiplying every term in one of the equations by [latex]-1[/latex]: [latex]-1\left(-3x+y\right )=2(-1)[/latex] becomes [latex]3x-y=-2[/latex].
[latex]\begin{equation}\begin{aligned}2x+y&=12\\3x-y&=-2\\ 5x\;\;\;\;\;&=10\\x&=2\end{aligned}\end{equation}[/latex]
We eliminated the [latex]y[/latex] variable, and solved for [latex]x[/latex]. We can now substitute [latex]x[/latex] into either of the original equations to solve for [latex]y[/latex]:
[latex]\begin{equation}\begin{aligned}2x+y&=12\\2(2)+y&=12\\4+y&=12\\y&=8\end{aligned}\end{equation}[/latex]
Consequently, the solution is [latex](2, 8)[/latex], which we can check by substituting it into the second equation:
[latex]\begin{equation}\begin{aligned}-3x+y&=2\\2(2)+8&=12\\4+8&=12\\12&=12\;\;\;\text{True}\end{aligned}\end{equation}[/latex]
As long as the coefficients are opposites we can eliminate the variable through addition. The following video describes a similar problem where one variable is eliminated by adding the two equations together.
Caution! When we need to add the opposite of one entire equation to another, make sure to change the sign of EVERY term on both sides of the equation. This is a very common mistake to make.
Example
Solve the system of equations.
[latex]\begin{array}{r}2x+y=12\\−3x+y=2\,\,\,\end{array}[/latex]
Solution
We can eliminate the [latex]y[/latex]-variable if we add the opposite of one of the equations to the other equation.
[latex]\begin{array}{r}2x+y=12\\−3x+y=2\,\,\,\end{array}[/latex]
Rewrite the second equation as its opposite: [latex]3x-y=-2[/latex]. Remember each term in the equation changes sign.
Add and solve for [latex]x[/latex]:
[latex]\begin{array}{r}2x+y=12\,\\3x–y=−2\\5x=10\,\\x=2\,\,\,\,\end{array}[/latex]
Substitute [latex]y=2[/latex] into one of the original equations and solve for [latex]y[/latex]:
[latex]\begin{array}{r}2x+y=12\\2\left(2\right)+y=12\\4+y=12\\\;y=8\,\,\,\end{array}[/latex]
Be sure to check your answer in the other equation:
[latex]\begin{array}{r}−3x+y=2\\−3\left(2\right)+8=2\\−6+8=2\\2=2\\\text{TRUE}\end{array}[/latex]
The answers check.
Answer
The solution is [latex](2, 8)[/latex].
Try It
The following are two more examples showing how to solve linear systems of equations using elimination.
Example
Use elimination to solve the system.
[latex]\begin{array}{r}−2x+3y=−1\\2x+5y=\,25\end{array}[/latex]
Solution
Notice the coefficients of each variable in each equation. If we add these two equations, the [latex]x[/latex] term will be eliminated since [latex]−2x+2x=0[/latex]:
[latex]\begin{array}{r}−2x+3y=−1\\2x+5y=\,25\end{array}[/latex]
Add and solve for [latex]y[/latex]:
[latex]\begin{array}{r}−2x+3y=−1\\2x+5y=25\,\\8y=24\,\\y=3\,\,\,\,\end{array}[/latex]
Substitute [latex]y=3[/latex] into one of the original equations:
[latex]\begin{array}{r}2x+5y=25\\2x+5\left(3\right)=25\\2x+15=25\\2x=10\\x=5\,\,\,\end{array}[/latex]
Check the solution in the other equation:
[latex]\begin{array}{r}−2x+3y=−1\\−2\left(5\right)+3\left(3\right)=−1\\−10+9=−1\\−1=−1\\\text{TRUE}\end{array}[/latex]
The answer checks.
Answer
The solution is [latex](5, 3)[/latex].
Example
Use elimination to solve for [latex]x[/latex] and [latex]y[/latex].
[latex]\begin{array}{r}4x+2y=14\\5x+2y=16\end{array}[/latex]
Solution
Notice the coefficients of each variable in each equation. We need to add the opposite of one of the equations to eliminate the variable [latex]y[/latex], as [latex]2y+2y=4y[/latex], but [latex]2y+\left(−2y\right)=0[/latex].
[latex]\begin{array}{r}4x+2y=14\\5x+2y=16\end{array}[/latex]
Change one of the equations to its opposite, add and solve for [latex]x[/latex]:
[latex]\begin{array}{r}4x+2y=14\,\,\,\,\\−5x–2y=−16\\−x=−2\,\,\,\\x=2\,\,\,\,\,\,\,\end{array}[/latex]
Substitute [latex]x=2[/latex] into one of the original equations and solve for [latex]y[/latex]:
[latex]\begin{array}{r}4x+2y=14\\4\left(2\right)+2y=14\\8+2y=14\\2y=6\,\,\,\\y=3\,\,\,\end{array}[/latex]
Check the solution in the other equation:
[latex]\begin{equation}\begin{aligned}5x+2y&=16\\5(2)+2(3)&=16\\10+6&=16\;\;\;\text{True}\end{aligned}\end{equation}[/latex]
Answer
The solution is [latex](2, 3)[/latex].
Try It
Solve the system of equations:
[latex]\begin{equation}\begin{aligned}x+2y&=10\\x-2y&=-6\end{aligned}\end{equation}[/latex]
Recognize systems that have no solution or an infinite number of solutions
Just as with the substitution method, the elimination method will sometimes eliminate both variables, and we end up with either a true statement or a false statement. Recall that a false statement implies an inconsistent system, which means that there is no solution. On the other hand, a true statement implies a dependent system, which means there are infinite solutions represented by either equation.
Let’s look at an example.
Example
Solve for the system of equations:
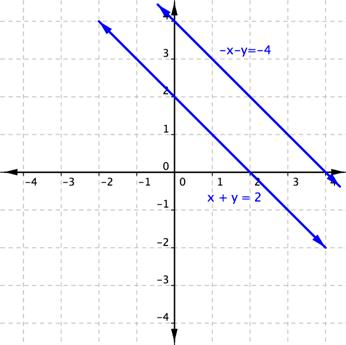
[latex]\begin{array}{r}-x–y=-4\\x+y=2\,\,\,\,\end{array}[/latex]
Solution
Add the equations to eliminate the [latex]x[/latex]-term:
[latex]\begin{array}{r}-x–y=-4\\\underline{x+y=2\,\,\,}\\0=−2\end{array}[/latex]
Both terms are eliminated and we get a contradiction.
Answer
There is no solution.
Graphing these lines shows that they are parallel lines and as such do not share any point in common, verifying that there is no solution.
Try It
If both variables are eliminated and we are left with a true statement, this indicates that there are an infinite number of ordered pairs that satisfy both of the equations. In fact, the equations are the same line.
Example
Solve for [latex]x[/latex] and [latex]y[/latex].
[latex]\begin{array}{r}x+y=2\,\,\,\,\\-x−y=-2\end{array}[/latex]
Solution
Add the equations to eliminate the [latex]x[/latex]-term:
[latex]\begin{array}{r}x+y=2\,\,\,\,\\\underline{-x−y=-2}\\0=0\,\,\,\,\,\end{array}[/latex]
The result is an identity, which is always true.
Answer
There are an infinite number of solutions represented by the equation [latex]x+y=2[/latex]. This can also be written as: [latex]\{\; (x,y) \;\large |\;\normalsize \; x+y=2\;\}[/latex]; read “the set of all ordered pairs [latex](x,y)[/latex] such that [latex]x+y=2[/latex].”
Graphing these two equations helps illustrate what is happening.
The two lines representing the equations lie on top of one another, so all solutions lie on this line.
Try It
Solve the system of equations:
[latex]\begin{equation}\begin{aligned}-2x+3y&=6\\2x-3y&=-6\end{aligned}\end{equation}[/latex]
In the following video, a system of equations which has no solutions is solved using the elimination method.
Summary
Combining equations is a powerful tool for solving a system of equations. Adding two equations in order to eliminate a common variable is called the elimination (or addition) method. Once one variable is eliminated, it becomes possible to solve for the other one.
