Learning Outcomes
- Graph a stretched or compressed exponential function.
- Graph a reflected exponential function.
- Write the equation of an exponential function that has been transformed.
While horizontal and vertical shifts involve adding constants to the input or to the function itself, a stretch or compression occurs when we multiply the parent function [latex]f\left(x\right)={b}^{x}[/latex] by a constant [latex]|a|>0[/latex]. For example, if we begin by graphing the parent function [latex]f\left(x\right)={2}^{x}[/latex], we can then graph the stretch, using [latex]a=3[/latex], to get [latex]g\left(x\right)=3{\left(2\right)}^{x}[/latex] and the compression, using [latex]a=\frac{1}{3}[/latex], to get [latex]h\left(x\right)=\frac{1}{3}{\left(2\right)}^{x}[/latex].
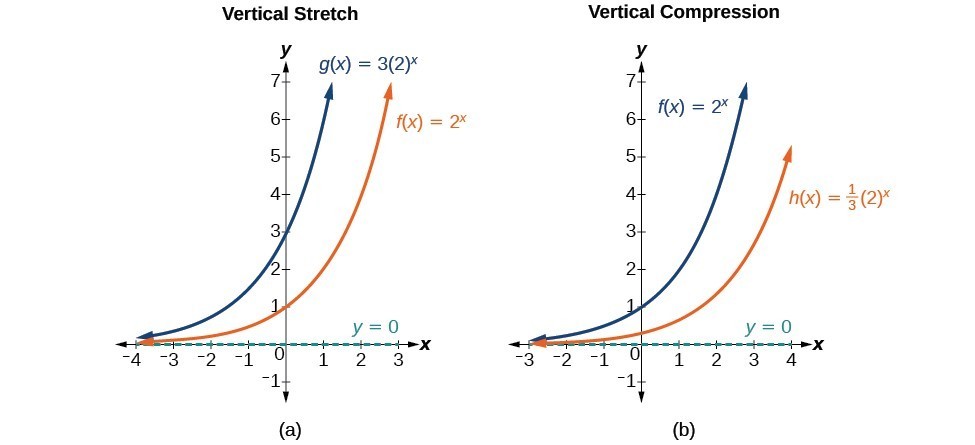
(a) [latex]g\left(x\right)=3{\left(2\right)}^{x}[/latex] stretches the graph of [latex]f\left(x\right)={2}^{x}[/latex] vertically by a factor of 3. (b) [latex]h\left(x\right)=\frac{1}{3}{\left(2\right)}^{x}[/latex] compresses the graph of [latex]f\left(x\right)={2}^{x}[/latex] vertically by a factor of [latex]\frac{1}{3}[/latex].
A General Note: Stretches and Compressions of the Parent Function [latex]f\left(x\right)={b}^{x}[/latex]
The function [latex]f\left(x\right)=a{b}^{x}[/latex]
- is stretched vertically by a factor of a if [latex]|a|>1[/latex].
- is compressed vertically by a factor of a if [latex]|a|<1[/latex].
- has a y-intercept is [latex]\left(0,a\right)[/latex].
- has a horizontal asymptote of [latex]y=0[/latex], range of [latex]\left(0,\infty \right)[/latex], and domain of [latex]\left(-\infty ,\infty \right)[/latex] which are all unchanged from the parent function.
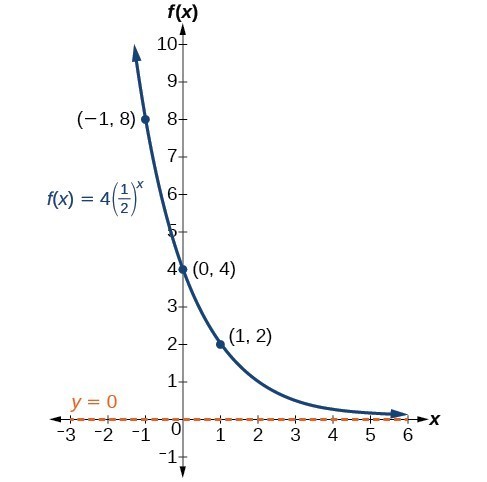
Example: Graphing the Stretch of an Exponential Function
Sketch a graph of [latex]f\left(x\right)=4{\left(\frac{1}{2}\right)}^{x}[/latex]. State the domain, range, and asymptote.
Try It
Graphing Reflections
In addition to shifting, compressing, and stretching a graph, we can also reflect it about the x-axis or the y-axis. When we multiply the parent function [latex]f\left(x\right)={b}^{x}[/latex] by –1, we get a reflection about the x-axis. When we multiply the input by –1, we get a reflection about the y-axis. For example, if we begin by graphing the parent function [latex]f\left(x\right)={2}^{x}[/latex], we can then graph the two reflections alongside it. The reflection about the x-axis, [latex]g\left(x\right)={-2}^{x}[/latex], and the reflection about the y-axis, [latex]h\left(x\right)={2}^{-x}[/latex], are both shown below.
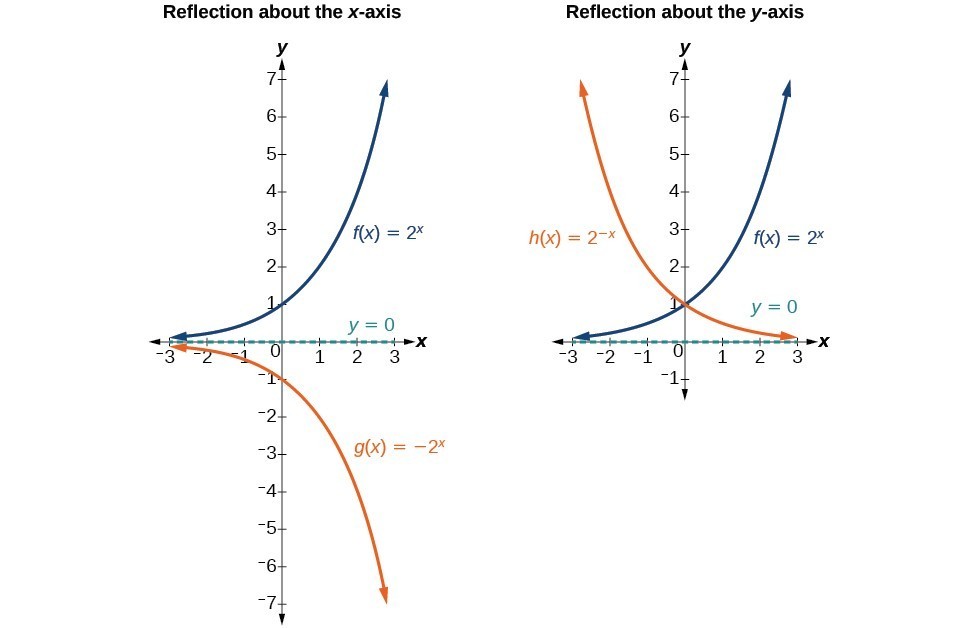
(a) [latex]g\left(x\right)=-{2}^{x}[/latex] reflects the graph of [latex]f\left(x\right)={2}^{x}[/latex] about the x-axis. (b) [latex]h\left(x\right)={2}^{-x}[/latex] reflects the graph of [latex]f\left(x\right)={2}^{x}[/latex] about the y-axis.
A General Note: Reflecting the Parent Function [latex]f\left(x\right)={b}^{x}[/latex]
The function [latex]f\left(x\right)=-{b}^{x}[/latex]
- reflects the parent function [latex]f\left(x\right)={b}^{x}[/latex] about the x-axis.
- has a y-intercept of [latex]\left(0,-1\right)[/latex].
- has a range of [latex]\left(-\infty ,0\right)[/latex].
- has a horizontal asymptote of [latex]y=0[/latex] and domain of [latex]\left(-\infty ,\infty \right)[/latex] which are unchanged from the parent function.
The function [latex]f\left(x\right)={b}^{-x}[/latex]
- reflects the parent function [latex]f\left(x\right)={b}^{x}[/latex] about the y-axis.
- has a y-intercept of [latex]\left(0,1\right)[/latex], a horizontal asymptote at [latex]y=0[/latex], a range of [latex]\left(0,\infty \right)[/latex], and a domain of [latex]\left(-\infty ,\infty \right)[/latex] which are unchanged from the parent function.
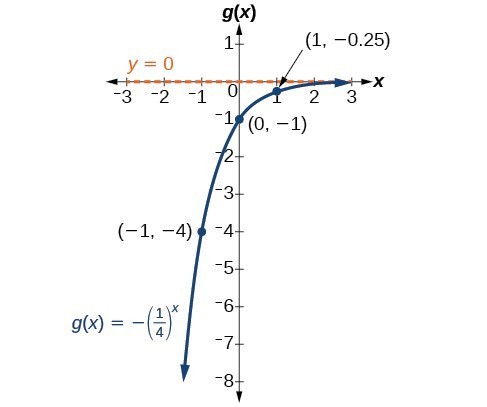
Example: Writing and Graphing the Reflection of an Exponential Function
Find and graph the equation for a function, [latex]g\left(x\right)[/latex], that reflects [latex]f\left(x\right)={\left(\frac{1}{4}\right)}^{x}[/latex] about the x-axis. State its domain, range, and asymptote.
Summarizing Transformations of the Exponential Function
Now that we have worked with each type of translation for the exponential function, we can summarize them to arrive at the general equation for transforming exponential functions.
| Transformations of the Parent Function [latex]f\left(x\right)={b}^{x}[/latex] | |
|---|---|
| Translation | Form |
Shift
|
[latex]f\left(x\right)={b}^{x+c}+d[/latex] |
Stretch and Compress
|
[latex]f\left(x\right)=a{b}^{x}[/latex] |
| Reflect about the x-axis | [latex]f\left(x\right)=-{b}^{x}[/latex] |
| Reflect about the y-axis | [latex]f\left(x\right)={b}^{-x}={\left(\frac{1}{b}\right)}^{x}[/latex] |
| General equation for all transformations | [latex]f\left(x\right)=a{b}^{x+c}+d[/latex] |
A General Note: Transformations of Exponential Functions
A transformation of an exponential function has the form
[latex]f\left(x\right)=a{b}^{x+c}+d[/latex], where the parent function, [latex]y={b}^{x}[/latex], [latex]b>1[/latex], is
- shifted horizontally c units to the left.
- stretched vertically by a factor of |a| if |a| > 0.
- compressed vertically by a factor of |a| if 0 < |a| < 1.
- shifted vertically d units.
- reflected about the x-axis when a < 0.
Note the order of the shifts, transformations, and reflections follow the order of operations.
Example: Writing a Function from a Description
Write the equation for the function described below. Give the horizontal asymptote, domain, and range.
- [latex]f\left(x\right)={e}^{x}[/latex] is vertically stretched by a factor of 2, reflected across the y-axis, and then shifted up 4 units.
Try It
Write the equation for the function described below. Give the horizontal asymptote, the domain, and the range.
- [latex]f\left(x\right)={e}^{x}[/latex] is compressed vertically by a factor of [latex]\frac{1}{3}[/latex], reflected across the x-axis, and then shifted down 2 units.
Contribute!
Candela Citations
- Revision and Adaptation. Provided by: Lumen Learning. License: CC BY: Attribution
- Question ID 129498. Authored by: Day, Alyson. License: CC BY: Attribution. License Terms: IMathAS Community LicenseCC-BY + GPL
- College Algebra. Authored by: Abramson, Jay et al.. Provided by: OpenStax. Located at: http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2. License: CC BY: Attribution. License Terms: Download for free at http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2