Learning Outcome
- Evaluate expressions and word problems involving percents
In this section we will solve percent questions by identifying the parts of the problem. We’ll look at a common application of percent—tips to a server at a restaurant—to see how to set up a basic percent application.
When Aolani and her friends ate dinner at a restaurant, the bill came to [latex]\text{\$80}[/latex]. They wanted to leave a [latex]20\%[/latex] tip. What amount would the tip be?
To solve this, we want to find what amount is [latex]20\%[/latex] of [latex]\$80[/latex]. The [latex]\$80[/latex] is called the base. The percent is the given [latex]20\%[/latex]. The amount of the tip would be [latex]0.20(80)[/latex], or [latex]\$16[/latex] — see the image below. To find the amount of the tip, we multiplied the percent by the base.
A [latex]20\%[/latex] tip for an [latex]\$80[/latex] restaurant bill comes out to [latex]\$16[/latex].
Pieces of a Percent Problem
Percent problems involve three quantities: the base amount (the whole), the percent, and the amount (a part of the whole or partial amount).
The amount is a percent of the base.
Let’s look at another example:
Jeff has a Guitar Strings coupon for [latex]15\%[/latex] off any purchase of [latex]$100[/latex] or more. He wants to buy a used guitar that has a price tag of [latex]$220[/latex] on it. Jeff wonders how much money the coupon will take off the original [latex]$220[/latex] price. Problems involving percents will have some combination of these three quantities to work with: the percent, the amount, and the base. The percent has the percent symbol (%) or the word percent. In the problem above, [latex]15\%[/latex] is the percent off the purchase price. The base is the whole amount or original amount. In the problem above, the “whole” price of the guitar is [latex]$220[/latex], which is the base. The amount is the unknown and what we will need to calculate.
There are thee cases: a missing amount, a missing percent or a missing base. Let’s take a look at each possibility.
Solving for the Amount
When solving for the amount in a percent problem, you will multiply the percent (as a decimal or fraction) by the base. Typically we choose the decimal value for percent.
[latex]\text{percent}\cdot{\text{base}}=\text{amount}[/latex]
Example
Find [latex]50\%[/latex] of [latex]20[/latex]
Solution:
First identify each piece of the problem:
percent: [latex]50\%[/latex] or [latex].5[/latex]
base: [latex]20[/latex]
amount: unknown
Now plug them into your equation [latex]\text{percent}\cdot{\text{base}}=\text{amount}[/latex]
[latex].5\cdot{20}= ?[/latex]
[latex].5\cdot{20}= 10[/latex]
Therefore, [latex]10[/latex] is the amount or part that is [latex]50\%[/latex] of [latex]20[/latex].
Example
What is [latex]25\%[/latex] of [latex]80[/latex]?
Try It
Solving for the Percent
When solving for the percent in a percent problem, you will divide the amount by the base. The equation above is rearranged and the percent will come back as a decimal of fraction you can report in the form asked of you.
[latex]\Large{\frac{\text{amount}}{\text{base}}}\normalsize=\text{percent}[/latex]
Example
What percent of [latex]320[/latex] is [latex]80[/latex]?
Solution:
First identify each piece of the problem:
percent: unknown
base: [latex]320[/latex]
amount: [latex]80[/latex]
Now plug the values into your equation [latex]\Large{\frac{\text{amount}}{\text{base}}}\normalsize=\text{percent}[/latex]
[latex]\large\frac{80}{320}\normalsize=?[/latex]
[latex]\large\frac{80}{320}\normalsize=.25[/latex]
Therefore, [latex]80[/latex] is [latex]25\%[/latex] of [latex]320[/latex].
TRY IT
Solving for the Base
When solving for the base in a percent problem, you will divide the amount by the percent (as a decimal or fraction). The equation above is rearranged and you will find the base after plugging in the values.
[latex]\Large{\frac{\text{amount}}{\text{percent}}}\normalsize=\text{base}[/latex]
EXample
[latex]60[/latex] is [latex]40\%[/latex] of what number?
Solution:
First identify each piece of the problem:
percent:[latex]40\%[/latex] or [latex].4[/latex]
base: unknown
amount: [latex]60[/latex]
Now plug the values into your equation [latex]\Large{\frac{\text{amount}}{\text{percent}}}\normalsize=\text{base}[/latex]
[latex](60)\div(.4)=?[/latex]
[latex](60)\div(.4)=150[/latex]
Therefore, [latex]60[/latex] is [latex]40\%[/latex] of [latex]150[/latex].
Example
An article says that [latex]15\%[/latex] of a non-profit’s donations, about [latex]$30,000[/latex] a year, comes from individual donors. What is the total amount of donations the non-profit receives?
TRY IT
Here are a few more percent problems for you to try.
try it
try it
try it
Many applications of percent occur in our daily lives, such as tips, sales tax, discount, and interest. To solve these applications we’ll translate to a basic percent equation, just like those we solved in the previous examples in this section. Once you translate the sentence into a percent equation, you know how to solve it.
example
Dezohn and his girlfriend enjoyed a dinner at a restaurant, and the bill was [latex]\text{\$68.50}[/latex]. They want to leave an [latex]\text{18%}[/latex] tip. If the tip will be [latex]\text{18%}[/latex] of the total bill, how much should the tip be?
Solution
| What are you asked to find? | the amount of the tip |
| What formula/equation should you use? | [latex]\text{percent}\cdot{\text{base}}=\text{amount}[/latex] |
| Substitute in the correct values. | [latex](.18)\cdot{68.50}[/latex] |
| Solve. | [latex](.18)\cdot{68.50}=12.33[/latex] |
| Write a complete sentence that answers the question. | The couple should leave a tip of [latex]\text{\$12.33}[/latex]. |
try it
In the next video we show another example of finding how much tip to give based on percent.
example
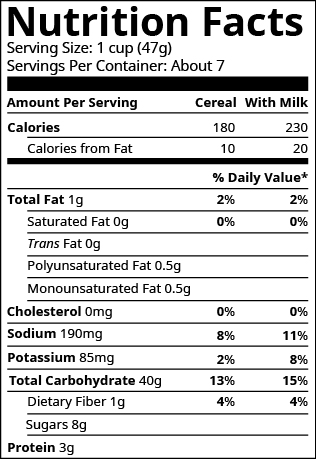
The label on Masao’s breakfast cereal said that one serving of cereal provides [latex]85[/latex] milligrams (mg) of potassium, which is [latex]\text{2%}[/latex] of the recommended daily amount. What is the total recommended daily amount of potassium?
