Introduction

Some important terminology before we begin:

• operations/operators: In mathematics we call things like multiplication, division, addition, and subtraction operations.  They are the verbs of the math world, doing work on numbers and variables. The symbols used to denote operations are called operators, such as [latex]+{, }-{, }\times{, }\div[/latex]. As you learn more math, you will learn more operators.
• term: Examples of terms would be [latex]2x[/latex] and [latex]-\frac{3}{2}[/latex] or [latex]a^3[/latex]. Even lone integers can be a term, like 0.
• expression: A mathematical expression is one that connects terms with mathematical operators. For example  [latex]\frac{1}{2}+\left(2^2\right)- 9\div\frac{6}{7}[/latex] is an expression.

Combining Like Terms

One way we can simplify expressions is to combine like terms. Like terms are terms where the variables match exactly (exponents included). Examples of like terms would be [latex]5xy[/latex] and [latex]-3xy[/latex] or [latex]8a^2b[/latex] and [latex]a^2b[/latex] or [latex]-3[/latex] and [latex]8[/latex].  If we have like terms we are allowed to add (or subtract) the numbers in front of the variables, then keep the variables the same. As we combine like terms we need to interpret subtraction signs as part of the following term. This means if we see a subtraction sign, we treat the following term like a negative term. The sign always stays with the term.

This is shown in the following examples:

In the following video you will be shown how to combine like terms using the idea of the distributive property.  Note that this is a different method than is shown in the written examples on this page, but it obtains the same result.

In the video that follows, you will be shown another example of combining like terms.  Pay attention to why you are not able to combine all three terms in the example.

Order of Operations

You may or may not recall the order of operations for applying several mathematical operations to one expression. Just as it is a social convention for us to drive on the right-hand side of the road, the order of operations is a set of conventions used to provide order when you are required to use several mathematical operations for one expression. The graphic below depicts the order in which mathematical operations are performed.

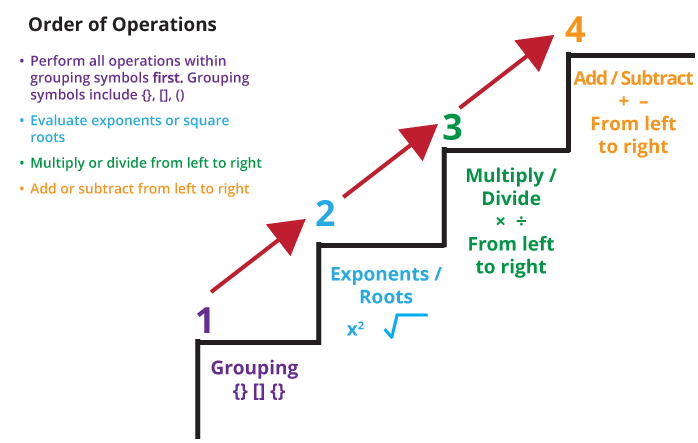
Order of Operations

In the following example, you will be shown how to simplify an expression that contains both multiplication and subtraction using the order of operations.

When you are applying the order of operations to expressions that contain fractions, decimals, and negative numbers, you will need to recall how to do these computations as well.

In the following video you are shown how to use the order of operations to simplify an expression that contains multiplication, division, and subtraction with terms that contain fractions.

Exponents

When you are evaluating expressions, you will sometimes see exponents used to represent repeated multiplication. Recall that an expression such as [latex]7^{2}[/latex] is exponential notation for [latex]7\cdot7[/latex]. (Exponential notation has two parts: the base and the exponent or the power. In [latex]7^{2}[/latex], 7 is the base and 2 is the exponent; the exponent determines how many times the base is multiplied by itself.)

Exponents are a way to represent repeated multiplication; the order of operations places it before any other multiplication, division, subtraction, and addition is performed.

In the video that follows, an expression with exponents on its terms is simplified using the order of operations.

Grouping Symbols

Grouping symbols such as parentheses ( ), brackets [ ], braces[latex]\displaystyle \left\{ {} \right\}[/latex], and fraction bars can be used to further control the order of the four arithmetic operations. The rules of the order of operations require computation within grouping symbols to be completed first, even if you are adding or subtracting within the grouping symbols and you have multiplication outside the grouping symbols. After computing within the grouping symbols, divide or multiply from left to right and then subtract or add from left to right. When there are grouping symbols within grouping symbols, calculate from the inside to the outside. That is, begin simplifying within the innermost grouping symbols first.

Remember that parentheses can also be used to show multiplication. In the example that follows, both uses of parentheses—as a way to represent a group, as well as a way to express multiplication—are shown.

In the following video, you are shown how to use the order of operations to simplify an expression with grouping symbols, exponents, multiplication, and addition.