Applications of Hyperbolic Functions

Learning Outcomes

  • Describe the common applied conditions of a catenary curve

One physical application of hyperbolic functions involves hanging cables. If a cable of uniform density is suspended between two supports without any load other than its own weight, the cable forms a curve called a catenary. High-voltage power lines, chains hanging between two posts, and strands of a spider’s web all form catenaries. The following figure shows chains hanging from a row of posts.

An image of chains hanging between posts that all take the shape of a catenary.

Figure 3. Chains between these posts take the shape of a catenary. (credit: modification of work by OKFoundryCompany, Flickr)

Hyperbolic functions can be used to model catenaries. Specifically, functions of the form [latex]y=a\text{cosh}\left(\frac{x}{a}\right)[/latex] are catenaries. Figure 4 shows the graph of [latex]y=2\text{cosh}\left(\frac{x}{2}\right).[/latex]

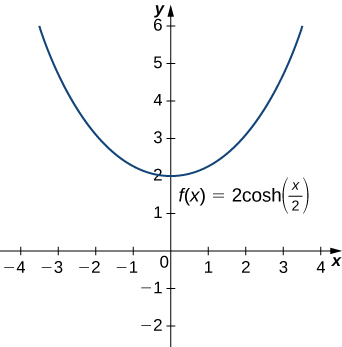
This figure is a graph. It is of the function f(x)=2cosh(x/2). The curve decreases in the second quadrant to the y-axis. It intersects the y-axis at y=2. Then the curve becomes increasing.

Figure 4. A hyperbolic cosine function forms the shape of a catenary.

example: Using a Catenary to Find the Length of a Cable

Assume a hanging cable has the shape [latex]10\text{cosh}\left(\frac{x}{10}\right)[/latex] for [latex]-15\le x\le 15,[/latex] where [latex]x[/latex] is measured in feet. Determine the length of the cable (in feet).

Watch the following video to see the worked solution to Example: Using a Catenary to Find the Length of a Cable.

Try It

Assume a hanging cable has the shape [latex]15\text{cosh}\left(\frac{x}{15}\right)[/latex] for [latex]-20\le x\le 20.[/latex] Determine the length of the cable (in feet).

Try It