Problem Set: Separable Equations

Solve the following initial-value problems with the initial condition ${y}_{0}=0$ and graph the solution.

1. $\frac{dy}{dt}=y+1$

Show Solution

$y={e}^{t}-1$

$\frac{dy}{dt}=y - 1$

3. $\frac{dy}{dt}=y+1$

Show Solution

$y=1-{e}^{\text{-}t}$

$\frac{dy}{dt}=\text{-}y - 1$

Find the general solution to the differential equation.

5. ${x}^{2}y^{\prime} =\left(x+1\right)y$

Show Solutoin

$y=Cx{e}^{\frac{-1}{x}}$

$y^{\prime} =\tan\left(y\right)x$

7. $y^{\prime} =2x{y}^{2}$

Show Solution

$y=\frac{1}{C-{x}^{2}}$

$\frac{dy}{dt}=y\cos\left(3t+2\right)$

9. $2x\frac{dy}{dx}={y}^{2}$

Show Solution

$y=-\frac{2}{C+\text{ln}x}$

10.

$y^{\prime} ={e}^{y}{x}^{2}$

11. $\left(1+x\right)y^{\prime} =\left(x+2\right)\left(y - 1\right)$

Show Solution

$y=C{e}^{x}\left(x+1\right)+1$

12.

$\frac{dx}{dt}=3{t}^{2}\left({x}^{2}+4\right)$

13. $t\frac{dy}{dt}=\sqrt{1-{y}^{2}}$

Show Solution

$y=\sin\left(\text{ln}t+C\right)$

14.

$y^{\prime} ={e}^{x}{e}^{y}$

Find the solution to the initial-value problem.

15. $y^{\prime} ={e}^{y-x},y\left(0\right)=0$

Show Solution

$y=\text{-}\text{ln}\left({e}^{\text{-}x}\right)$

16.

$y^{\prime} ={y}^{2}\left(x+1\right),y\left(0\right)=2$

17. $\frac{dy}{dx}={y}^{3}x{e}^{{x}^{2}},y\left(0\right)=1$

Show Solution

$y=\frac{1}{\sqrt{2-{e}^{{x}^{2}}}}$

18.

$\frac{dy}{dt}={y}^{2}{e}^{x}\sin\left(3x\right),y\left(0\right)=1$

19. $y^{\prime} =\frac{x}{{\text{sech}}^{2}y},y\left(0\right)=0$

Show Solution

$y={\text{tanh}}^{-1}\left(\frac{{x}^{2}}{2}\right)$

20.

$y^{\prime} =2xy\left(1+2y\right),y\left(0\right)=-1$

21. $\frac{dx}{dt}=\text{ln}\left(t\right)\sqrt{1-{x}^{2}},x\left(1\right)=0$

Show Solution

$x=\sin\left(1-t+t\text{ln}t\right)$

22.

$y^{\prime} =3{x}^{2}\left({y}^{2}+4\right),y\left(0\right)=0$

23. $y^{\prime} ={e}^{y}{5}^{x},y\left(0\right)=\text{ln}\left(\text{ln}\left(5\right)\right)$

Show Solution

$y=\text{ln}\left(\text{ln}\left(5\right)\right)-\text{ln}\left(2-{5}^{x}\right)$

24.

$y^{\prime} =-2x\tan\left(y\right),y\left(0\right)=\frac{\pi }{2}$

For the following problems, use a software program or your calculator to generate the directional fields. Solve explicitly and draw solution curves for several initial conditions. Are there some critical initial conditions that change the behavior of the solution?

25. [T] $y^{\prime} =1 - 2y$

Show Solution

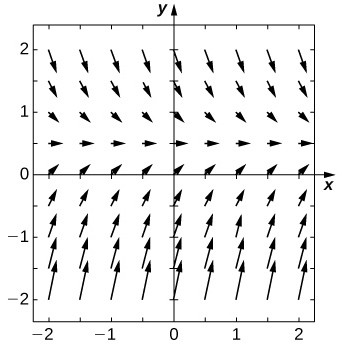
$y=C{e}^{-2x}+\frac{1}{2}$ A direction field with horizontal arrows pointing to the right at y = 0.5. Above 0.5, the arrows slope down and to the right and are increasingly vertical the further they are from y = 0.5 Below 0.5, the arrows slope up and to the right and are increasingly vertical the further they are from y = 0.5.

A direction field with horizontal arrows pointing to the right at y = 0.5. Above 0.5, the arrows slope down and to the right and are increasingly vertical the further they are from y = 0.5 Below 0.5, the arrows slope up and to the right and are increasingly vertical the further they are from y = 0.5.

26. [T]

$y^{\prime} ={y}^{2}{x}^{3}$

27. [T] $y^{\prime} ={y}^{3}{e}^{x}$

Show Solution

$y=\frac{1}{\sqrt{2}\sqrt{C-{e}^{x}}}$ A direction field with arrows pointing to the right. They are horizontal at the y axis. The further the arrows are from the axis, the more vertical they become. They point up above the x-axis and down below the x axis.

A direction field with arrows pointing to the right. They are horizontal at the y axis. The further the arrows are from the axis, the more vertical they become. They point up above the x-axis and down below the x axis.

28. [T]

$y^{\prime} ={e}^{y}$

29. [T] $y^{\prime} =y\text{ln}\left(x\right)$

Show Solution

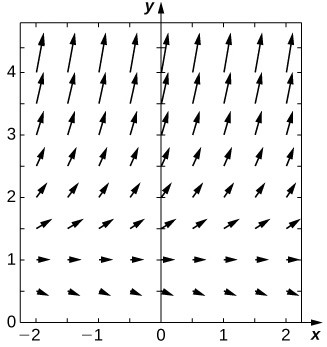
$y=C{e}^{\text{-}x}{x}^{x}$ A direction field with arrows pointing to the right. The arrows are flat on y = 1. The further the arrows are from that, the steeper they become. They point up above that line and down below that line.

A direction field with arrows pointing to the right. The arrows are flat on y = 1. The further the arrows are from that, the steeper they become. They point up above that line and down below that line.

30. Most drugs in the bloodstream decay according to the equation

$y^{\prime} =cy$ , where

$y$ is the concentration of the drug in the bloodstream. If the half-life of a drug is

$2$ hours, what fraction of the initial dose remains after

$6$ hours?

31. A drug is administered intravenously to a patient at a rate $r$ mg/h and is cleared from the body at a rate proportional to the amount of drug still present in the body, $d$ Set up and solve the differential equation, assuming there is no drug initially present in the body.

Show Solution

$y=\frac{r}{d}\left(1-{e}^{\text{-}dt}\right)$

32. [T] How often should a drug be taken if its dose is

$3$ mg, it is cleared at a rate

$c=0.1$ mg/h, and

$1$ mg is required to be in the bloodstream at all times?

33. A tank contains $1$ kilogram of salt dissolved in $100$ liters of water. A salt solution of $0.1$ kg salt/L is pumped into the tank at a rate of $2$ L/min and is drained at the same rate. Solve for the salt concentration at time $t$ . Assume the tank is well mixed.

Show Solution

$y\left(t\right)=10 - 9{e}^{\frac{\text{-}x}{50}}$

34. A tank containing

$10$ kilograms of salt dissolved in

$1000$ liters of water has two salt solutions pumped in. The first solution of

$0.2$ kg salt/L is pumped in at a rate of

$20$ L/min and the second solution of

$0.05$ kg salt/L is pumped in at a rate of

$5$ L/min. The tank drains at

$25$ L/min. Assume the tank is well mixed. Solve for the salt concentration at time

$t$ .

35. [T] For the preceding problem, find how much salt is in the tank $1$ hour after the process begins.

Show Solution

$134.3$ kilograms

36. Torricelli’s law states that for a water tank with a hole in the bottom that has a cross-section of

$A$ and with a height of water

$h$ above the bottom of the tank, the rate of change of volume of water flowing from the tank is proportional to the square root of the height of water, according to

$\frac{dV}{dt}=\text{-}A\sqrt{2gh}$ , where

$g$ is the acceleration due to gravity. Note that

$\frac{dV}{dt}=A\frac{dh}{dt}$ . Solve the resulting initial-value problem for the height of the water, assuming a tank with a hole of radius

$2$ ft. The initial height of water is

$100$ ft.

37. For the preceding problem, determine how long it takes the tank to drain.

Show Solution

$720$ seconds

For the following problems, use Newton’s law of cooling.

38. The liquid base of an ice cream has an initial temperature of

$200^\circ\text{F}$ before it is placed in a freezer with a constant temperature of

$0^\circ\text{F}\text{.}$ After

$1$ hour, the temperature of the ice-cream base has decreased to

$140^\circ\text{F}\text{.}$ Formulate and solve the initial-value problem to determine the temperature of the ice cream.

39. [T] The liquid base of an ice cream has an initial temperature of $210^\circ\text{F}$ before it is placed in a freezer with a constant temperature of $20^\circ\text{F}\text{.}$ After $2$ hours, the temperature of the ice-cream base has decreased to $170^\circ\text{F}\text{.}$ At what time will the ice cream be ready to eat? (Assume $30^\circ\text{F}$ is the optimal eating temperature.)

Show Solution

$24$ hours

$57$ minutes

40. [T] You are organizing an ice cream social. The outside temperature is

$80^\circ\text{F}$ and the ice cream is at

$10^\circ\text{F}\text{.}$ After

$10$ minutes, the ice cream temperature has risen by

$10^\circ\text{F}\text{.}$ How much longer can you wait before the ice cream melts at

$40^\circ\text{F?}$

41. You have a cup of coffee at temperature $70^\circ\text{C}$ and the ambient temperature in the room is $20^\circ\text{C}\text{.}$ Assuming a cooling rate $k\text{ of }0.125$ , write and solve the differential equation to describe the temperature of the coffee with respect to time.

Show Solution

$T\left(t\right)=20+50{e}^{-0.125t}$

42. [T] You have a cup of coffee at temperature

$70^\circ\text{C}$ that you put outside, where the ambient temperature is

$0^\circ\text{C}\text{.}$ After

$5$ minutes, how much colder is the coffee?

43. You have a cup of coffee at temperature $70^\circ\text{C}$ and you immediately pour in $1$ part milk to $5$ parts coffee. The milk is initially at temperature $1^\circ\text{C}\text{.}$ Write and solve the differential equation that governs the temperature of this coffee.

Show Solution

$T\left(t\right)=20+38.5{e}^{-0.125t}$

44. You have a cup of coffee at temperature

$70^\circ\text{C}$ , which you let cool

$10$ minutes before you pour in the same amount of milk at

$1^\circ\text{C}$ as in the preceding problem. How does the temperature compare to the previous cup after

$10$ minutes?

45. Solve the generic problem $y^{\prime} =ay+b$ with initial condition $y\left(0\right)=c$ .

Show Solution

$y=\left(c+\frac{b}{a}\right){e}^{ax}-\frac{b}{a}$

46. Prove the basic continual compounded interest equation. Assuming an initial deposit of

${P}_{0}$ and an interest rate of

$r$ , set up and solve an equation for continually compounded interest.

47. Assume an initial nutrient amount of $I$ kilograms in a tank with $L$ liters. Assume a concentration of $c$ kg/L being pumped in at a rate of $r$ L/min. The tank is well mixed and is drained at a rate of $r$ L/min. Find the equation describing the amount of nutrient in the tank.

Show Solution

$y\left(t\right)=cL+\left(I-cL\right){e}^{\frac{\text{-}rt}{L}}$

48. Leaves accumulate on the forest floor at a rate of

$2$ g/cm²/yr and also decompose at a rate of

$90\text{%}$ per year. Write a differential equation governing the number of grams of leaf litter per square centimeter of forest floor, assuming at time

$0$ there is no leaf litter on the ground. Does this amount approach a steady value? What is that value?

49. Leaves accumulate on the forest floor at a rate of $4$ g/cm²/yr. These leaves decompose at a rate of $10\text{%}$ per year. Write a differential equation governing the number of grams of leaf litter per square centimeter of forest floor. Does this amount approach a steady value? What is that value?

Show Solution

$y=40\left(1-{e}^{-0.1t}\right),40$ g/cm2

Differential Equations: Supplemental Content

Problem Set: Separable Equations

Candela Citations