Section 6.3: Properties of the Trigonometric Functions; Reference Angles

Learning Outcomes

  • Use reference angles to evaluate trigonometric functions.
  • Use even-odd properties to find the exact values of the trigonometric functions.
  • Use periodic properties to find the exact values of the trigonometric functions.

Reference Angles

An angle’s reference angle is the measure of the smallest, positive, acute angle [latex]t[/latex] formed by the terminal side of the angle [latex]t[/latex] and the horizontal axis. Thus positive reference angles have terminal sides that lie in the first quadrant and can be used as models for angles in other quadrants. See Figure 1 for examples of reference angles for angles in different quadrants. 

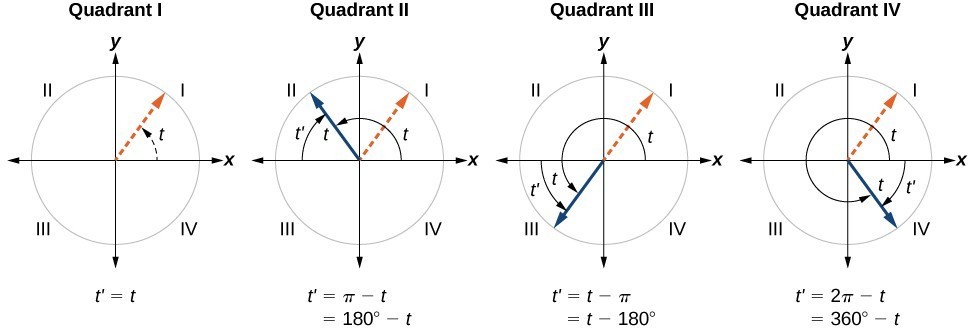
Four side by side graphs. First graph shows an angle of t in quadrant 1 in it's normal position. Second graph shows an angle of t in quadrant 2 due to a rotation of pi minus t. Third graph shows an angle of t in quadrant 3 due to a rotation of t minus pi. Fourth graph shows an angle of t in quadrant 4 due to a rotation of two pi minus t.

Figure 1

A GENERAL NOTE: REFERENCE ANGLES

An angle’s reference angle is the size of the smallest acute angle, [latex]{t}^{\prime }[/latex], formed by the terminal side of the angle [latex]t[/latex] and the horizontal axis.

How To: Given an angle between [latex]0[/latex] and [latex]2\pi [/latex], find its reference angle.

  1. An angle in the first quadrant is its own reference angle.
  2. For an angle in the second or third quadrant, the reference angle is [latex]|\pi -t|[/latex] or [latex]|180^\circ \mathrm{-t}|[/latex].
  3. For an angle in the fourth quadrant, the reference angle is [latex]2\pi -t[/latex] or [latex]360^\circ \mathrm{-t}[/latex].
  4. If an angle is less than [latex]0[/latex] or greater than [latex]2\pi [/latex], add or subtract [latex]2\pi [/latex] as many times as needed to find an equivalent angle between [latex]0[/latex] and [latex]2\pi [/latex].

Example 1: Finding a Reference Angle

Find the reference angle of [latex]225^\circ [/latex] as shown in Figure 2.

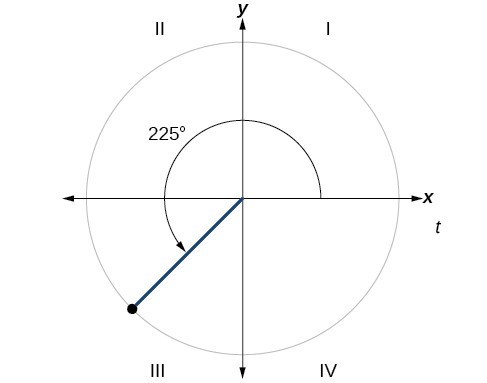
Graph of circle with 225 degree angle inscribed.

Figure 2

Try It

Find the reference angle of [latex]\frac{5\pi }{3}[/latex].

Using Reference Angles

Reference angles make it possible to evaluate trigonometric functions for angles outside the first quadrant. They can also be used to find [latex]\left(x,y\right)[/latex] coordinates for those angles. We will use the reference angle of the angle of rotation combined with the quadrant in which the terminal side of the angle lies.  We can find the exact trig value of any angle in any quadrant if we apply the trig function to the reference angle.  The sign depends on the quadrant of the original angle.

The trigonometric function values for the original angle will be the same as those for the reference angle, except for the positive or negative sign, which is determined by x– and y-values in the original quadrant. Figure 3 shows which functions are positive in which quadrant.

To help us remember which of the six trigonometric functions are positive in each quadrant, we can use the mnemonic phrase “All Students Take Calculus” Each of the four words in the phrase corresponds to one of the four quadrants, starting with quadrant I and rotating counterclockwise. In quadrant I, which is “A,” all of the six trigonometric functions are positive. In quadrant II, “Students,” only sine and its reciprocal function, cosecant, are positive. In quadrant III, “Take,” only tangent and its reciprocal function, cotangent, are positive. Finally, in quadrant IV, “Calculus” only cosine and its reciprocal function, secant, are positive.

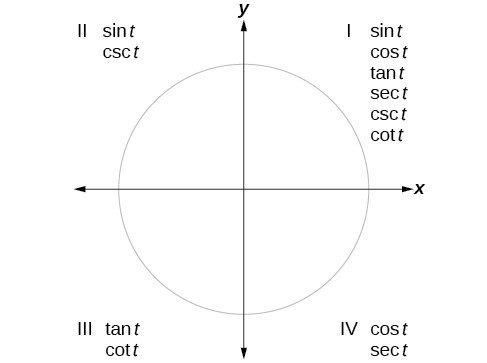
Graph of circle with each quadrant labeled. Under quadrant 1, labels fro sin t, cos t, tan t, sec t, csc t, and cot t. Under quadrant 2, labels for sin t and csc t. Under quadrant 3, labels for tan t and cot t. Under quadrant 4, labels for cos t, sec t.

Figure 3

How To: Find the trigonometric value for any angle

  1. Measure the angle between the terminal side of the given angle and the horizontal axis. This is the reference angle.
  2. Apply the trig function to the reference angle.
  3. Apply the appropriate sign using the table above.

Example 2: Using Reference Angles to Find Sine and Cosine

  1. Using a reference angle, find the exact value of [latex]\cos \left(150^\circ \right)[/latex] and [latex]\text{sin}\left(150^\circ \right)[/latex].
  2. Using the reference angle, find [latex]\cos \frac{5\pi }{4}[/latex] and [latex]\sin \frac{5\pi }{4}[/latex].

Example 3: Using Reference Angles to Find Tangent and Cotangent

  1. Using a reference angle, find the exact value of [latex]\tan \left(240^\circ \right)[/latex]
  2. Using the reference angle, find [latex]\cot \frac{7\pi }{4}[/latex]

Example 4: Using Reference Angles to Find Secant and Cosecant

  1. Using a reference angle, find the exact value of [latex]\sec \left(210^\circ \right)[/latex] and [latex]\csc\left(210^\circ \right)[/latex].
  2. Using the reference angle, find [latex]\sec \frac{3\pi }{4}[/latex] and [latex]\csc \frac{3\pi }{4}[/latex].

Try It

a. Use the reference angle of [latex]315^\circ [/latex] to find [latex]\cos \left(315^\circ \right)[/latex] and [latex]\tan \left(315^\circ \right)[/latex].

b. Use the reference angle of [latex]\frac{2\pi }{3}[/latex] to find [latex]\sec \left(\frac{2\pi }{3}\right)[/latex] and [latex]\cot \left(\frac{2\pi }{3}\right)[/latex].

Try It

Example 5: Using Reference Angles to Find Trigonometric Functions

Use reference angles to find all six trigonometric functions of [latex]-\frac{5\pi }{6}[/latex].

Try It

Use reference angles to find all six trigonometric functions of [latex]-\frac{7\pi }{4}[/latex].

Example 6: Using Reference Angles to Find Trigonometric Functions

Use reference angles to find all six trigonometric functions of [latex]-585^\circ[/latex].

Try It

Example 7: Using the Unit Circle to Find Coordinates

Find the coordinates of the point on the unit circle at an angle of [latex]\frac{7\pi }{6}[/latex].

Try It

Find the coordinates of the point on the unit circle at an angle of [latex]\frac{5\pi }{3}[/latex].

Example 8: Finding the Exact Value Involving Tangent

Given that [latex]\tan \theta =−\frac{3}{4}[/latex] and [latex]\theta [/latex] is in quadrant II, find the following:

  1. [latex]\sin \left(\theta \right)[/latex]
  2. [latex]\csc \left(\theta \right)[/latex]
  3. [latex]\cos \left(\theta \right)[/latex]
  4. [latex]\sec \left(\theta \right)[/latex]
  5. [latex]\cot \left(\theta \right)[/latex]

Try It

Given that [latex]\tan \theta =\frac{5}{12}[/latex] and [latex]\theta [/latex] is in quadrant III, find the following:

  1. [latex]\sin \left(\theta \right)[/latex]
  2. [latex]\csc \left(\theta \right)[/latex]
  3. [latex]\cos \left(\theta \right)[/latex]
  4. [latex]\sec \left(\theta \right)[/latex]
  5. [latex]\cot \left(\theta \right)[/latex]

Using Even and Odd Trigonometric Functions

To be able to use our six trigonometric functions freely with both positive and negative angle inputs, we should examine how each function treats a negative input. As it turns out, there is an important difference among the functions in this regard.

Consider the function [latex]f\left(x\right)={x}^{2}[/latex], shown in Figure 5. The graph of the function is symmetrical about the y-axis. All along the curve, any two points with opposite x-values have the same function value. This matches the result of calculation: [latex]{\left(4\right)}^{2}={\left(-4\right)}^{2}[/latex], [latex]{\left(-5\right)}^{2}={\left(5\right)}^{2}[/latex], and so on. So [latex]f\left(x\right)={x}^{2}[/latex] is an even function, a function such that two inputs that are opposites have the same output. That means [latex]f\left(-x\right)=f\left(x\right)[/latex].

Graph of parabola with points (-2, 4) and (2, 4) labeled.

Figure 5. The function [latex]f\left(x\right)={x}^{2}[/latex] is an even function.

Now consider the function [latex]f\left(x\right)={x}^{3}[/latex], shown in Figure 6. The graph is not symmetrical about the y-axis. All along the graph, any two points with opposite x-values also have opposite y-values. So [latex]f\left(x\right)={x}^{3}[/latex] is an odd function, one such that two inputs that are opposites have outputs that are also opposites. That means [latex]f\left(-x\right)=-f\left(x\right)[/latex].

Graph of function with labels for points (-1, -1) and (1, 1).

Figure 6. The function [latex]f\left(x\right)={x}^{3}[/latex] is an odd function.

We can test whether a trigonometric function is even or odd by drawing a unit circle with a positive and a negative angle, as in Figure 7. The sine of the positive angle is [latex]y[/latex]. The sine of the negative angle is −y. The sine function, then, is an odd function. We can test each of the six trigonometric functions in this fashion. The results are shown in in the table below.

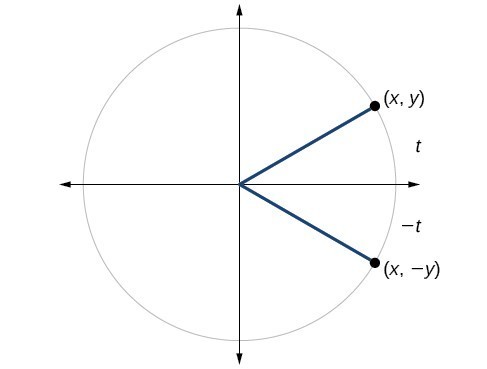
Graph of circle with angle of t and -t inscribed. Point of (x, y) is at intersection of terminal side of angle t and edge of circle. Point of (x, -y) is at intersection of terminal side of angle -t and edge of circle.

Figure 7

[latex]\begin{array}{l}\sin t=y\hfill \\ \sin \left(-t\right)=-y\hfill \\ \sin t\ne \sin \left(-t\right)\hfill \end{array}[/latex] [latex]\begin{array}{l}\text{cos}t=x\hfill \\ \cos \left(-t\right)=x\hfill \\ \cos t=\cos \left(-t\right)\hfill \end{array}[/latex] [latex]\begin{array}{l}\text{tan}\left(t\right)=\frac{y}{x}\hfill \\ \tan \left(-t\right)=-\frac{y}{x}\hfill \\ \tan t\ne \tan \left(-t\right)\hfill \end{array}[/latex]
[latex]\begin{array}{l}\sec t=\frac{1}{x}\hfill \\ \sec \left(-t\right)=\frac{1}{x}\hfill \\ \sec t=\sec \left(-t\right)\hfill \end{array}[/latex] [latex]\begin{array}{l}\csc t=\frac{1}{y}\hfill \\ \csc \left(-t\right)=\frac{1}{-y}\hfill \\ \csc t\ne \csc \left(-t\right)\hfill \end{array}[/latex] [latex]\begin{array}{l}\cot t=\frac{x}{y}\hfill \\ \cot \left(-t\right)=\frac{x}{-y}\hfill \\ \cot t\ne cot\left(-t\right)\hfill \end{array}[/latex]

A General Note: Even and Odd Trigonometric Functions

An even function is one in which [latex]f\left(-x\right)=f\left(x\right)[/latex].

An odd function is one in which [latex]f\left(-x\right)=-f\left(x\right)[/latex].

Cosine and secant are even:

[latex]\begin{gathered}\cos \left(-t\right)=\cos t \\ \sec \left(-t\right)=\sec t \end{gathered}[/latex]

Sine, tangent, cosecant, and cotangent are odd:

[latex]\begin{gathered}\sin \left(-t\right)=-\sin t \\ \tan \left(-t\right)=-\tan t \\ \csc \left(-t\right)=-\csc t \\ \cot \left(-t\right)=-\cot t \end{gathered}[/latex]

Example 7: Using Even and Odd Properties of Trigonometric Functions

If the [latex]\sec t=2[/latex], what is the [latex]\sec (-t)[/latex]?

Try It

If the [latex]\cot t=\sqrt{3}[/latex], what is [latex]\cot (-t)[/latex]?

Periodic Properties

If you add or subtract one revolution [latex]\left(360^\circ \text{ or }2\pi\right)[/latex] to an angle, the result will be the same because going around one full revolution will result in the same place on the unit circle. We will let [latex]k[/latex] be any integer, and this represents [latex]k[/latex] revolution in the equations below. These formulas are presented in radians, however they can also be expressed in degrees if we use [latex]360^\circ k[/latex].

A General Note: Periodic Properties

Let [latex]k[/latex] be an integer, and [latex]t[/latex] represent an angle.

[latex]\begin{gathered}\sin\left(t\pm 2\pi k\right)=\sin(t) \\ \cos\left(t\pm 2\pi k\right)=\cos(t) \\ \tan\left(t\pm 2\pi k\right)=\tan(t) \\ \csc\left(t\pm 2\pi k\right)=\csc(t) \\ \sec\left(t\pm 2\pi k\right)=\sec(t) \\ \cot\left(t\pm 2\pi k\right)=\cot(t)\end{gathered}[/latex]

Example 8: Simplifying using Even-Odd Properties and Periodic Properties

Use the Even-Odd Properties and Periodic Properties to simplify:
[latex]7\cos\left(-2t\right)+4\sin(-2t)-3\cos\left(2t-2\pi\right)[/latex]

Key Equations

Cosine [latex]\cos t=x[/latex]
Sine [latex]\sin t=y[/latex]
Pythagorean Identity [latex]{\cos }^{2}t+{\sin }^{2}t=1[/latex]

Key Concepts

  • The sine and cosine of an angle have the same absolute value as the sine and cosine of its reference angle.
  • The signs of the sine and cosine are determined from the x– and y-values in the quadrant of the original angle.
  • An angle’s reference angle is the size angle, [latex]t[/latex], formed by the terminal side of the angle [latex]t[/latex] and the horizontal axis.
  • Reference angles can be used to find the sine and cosine of the original angle.
  • Reference angles can also be used to find the coordinates of a point on a circle.

 

Section 6.3 Homework Exercises

1. Discuss the difference between a coterminal angle and a reference angle.

2. Explain how the cosine of an angle in the second quadrant differs from the cosine of its reference angle in the unit circle.

3. Explain how the sine of an angle in the second quadrant differs from the sine of its reference angle in the unit circle.

4. What is the purpose of a reference angle?

For the following exercises, state the reference angle for the given angle.

5. [latex]240^\circ [/latex]

6. [latex]-170^\circ [/latex]

7. [latex]460^\circ [/latex]

8. [latex]-675^\circ [/latex]

9. [latex]135^\circ [/latex]

10. [latex]\frac{5\pi }{4}[/latex]

11. [latex]\frac{2\pi }{3}[/latex]

12. [latex]\frac{17\pi }{6}[/latex]

13. [latex]-\frac{17\pi }{3}[/latex]

14. [latex]-\frac{7\pi }{4}[/latex]

15. [latex]-\frac{\pi }{8}[/latex]

For the following exercises, find the reference angle, the quadrant of the terminal side, and the sine, cosine of each angle.

16. [latex]225^\circ [/latex]

17. [latex]300^\circ [/latex]

18. [latex]315^\circ [/latex]

19. [latex]135^\circ [/latex]

20. [latex]570^\circ [/latex]

21. [latex]480^\circ [/latex]

22. [latex]-120^\circ [/latex]

23. [latex]-210^\circ [/latex]

24. [latex]\frac{5\pi }{4}[/latex]

25. [latex]\frac{7\pi }{6}[/latex]

26. [latex]\frac{5\pi }{3}[/latex]

27. [latex]\frac{3\pi }{4}[/latex]

28. [latex]\frac{4\pi }{3}[/latex]

29. [latex]\frac{2\pi }{3}[/latex]

30. [latex]\frac{-19\pi }{6}[/latex]

31. [latex]\frac{-9\pi }{4}[/latex]

For the following exercises, find the reference angle, the quadrant of the terminal side, and the exact value of the trigonometric function.

32. [latex]\tan \frac{5\pi }{6}[/latex]

33. [latex]\sec \frac{7\pi }{6}[/latex]

34. [latex]\csc \frac{11\pi }{6}[/latex]

35. [latex]\cot \frac{13\pi }{6}[/latex]

36. [latex]\tan \frac{15\pi }{4}[/latex]

37. [latex]\sec \frac{3\pi }{4}[/latex]

38. [latex]\csc \frac{5\pi }{4}[/latex]

39. [latex]\cot \frac{11\pi }{4}[/latex]

40. [latex]\tan \left(-\frac{4\pi }{3}\right)[/latex]

41. [latex]\sec \left(-\frac{2\pi }{3}\right)[/latex]

42. [latex]\csc \left(-\frac{10\pi }{3}\right)[/latex]

43. [latex]\cot \left(-\frac{7\pi }{3}\right)[/latex]

44. [latex]\tan 225^\circ [/latex]

45. [latex]\sec 300^\circ [/latex]

46. [latex]\csc 510^\circ [/latex]

47. [latex]\cot 600^\circ [/latex]

48. [latex]\tan \left(-30^\circ\right) [/latex]

49. [latex]\sec \left(-210^\circ\right) [/latex]

50. [latex]\csc \left(-510^\circ\right) [/latex]

51. [latex]\cot \left(-405^\circ\right) [/latex]

In the following exercises, use a right triangle to find the exact value.

52. If [latex]\text{sin}t=\frac{3}{4}[/latex], and [latex]t[/latex] is in quadrant II, find [latex]\cos t,\sec t,\csc t,\tan t,\cot t[/latex].

53. If [latex]\text{cos}t=-\frac{1}{3}[/latex], and [latex]t[/latex] is in quadrant III, find [latex]\sin t,\sec t,\csc t,\tan t,\cot t[/latex].

54. If [latex]\tan t=\frac{12}{5}[/latex], and [latex]0\le t<\frac{\pi }{2}[/latex], find [latex]\sin t,\cos t,\sec t,\csc t[/latex], and [latex]\cot t[/latex].

55. If [latex]\sin t=\frac{\sqrt{3}}{2}[/latex] and [latex]\cos t=\frac{1}{2}[/latex], find [latex]\sec t,\csc t,\tan t[/latex], and [latex]\cot t[/latex].

For the following exercises, find the exact value using reference angles.

56. [latex]\sin\left(\frac{11\pi}{3}\right)\cos\left(\frac{-5\pi}{6}\right)[/latex]

57. [latex]\sin\left(\frac{3\pi}{4}\right)\cos\left(\frac{5\pi}{3}\right)[/latex]

58. [latex]\sin\left(\frac{-4\pi}{3}\right)\cos\left(\frac{\pi}{2}\right)[/latex]

59. [latex]\sin\left(\frac{-9\pi}{4}\right)\cos\left(\frac{-\pi}{6}\right)[/latex]

60. [latex]\sin\left(\frac{\pi}{6}\right)\cos\left(\frac{-\pi}{3}\right)[/latex]

61. [latex]\sin\left(\frac{7\pi}{4}\right)\cos\left(\frac{-2\pi}{3}\right)[/latex]

62. [latex]\cos\left(\frac{5\pi}{6}\right)\cos\left(\frac{2\pi}{3}\right)[/latex]

63. [latex]\cos\left(\frac{-\pi}{3}\right)\cos\left(\frac{\pi}{4}\right)[/latex]

64. [latex]\sin\left(\frac{-5\pi}{4}\right)\sin\left(\frac{11\pi}{6}\right)[/latex]

65. [latex]\sin\left(\pi\right)\sin\left(\frac{\pi}{6}\right)[/latex]