1.6 Section Exercises

1.6 Section Exercises

Verbal

1. When examining the formula of a function that is the result of multiple transformations, how can you tell a horizontal shift from a vertical shift?

2. When examining the formula of a function that is the result of multiple transformations, how can you tell a reflection with respect to the x-axis from a reflection with respect to the y-axis?

3. How can you determine whether a function is odd or even from the formula of the function?

Algebraic

4. Write a formula for the function obtained when the graph of[latex]\text{ }f\left(x\right)=\sqrt[\leftroot{1}\uproot{2} ]{x}\text{ }[/latex]is shifted up 1 unit and to the left 2 units.

5. Write a formula for the function obtained when the graph of[latex]\text{ }f\left(x\right)=|x|\text{ }[/latex] is shifted down 3 units and to the right 1 unit.

6. Write a formula for the function obtained when the graph of[latex]\text{ }f\left(x\right)=\frac{1}{x}\text{ }[/latex]is shifted down 4 units and to the right 3 units.

7. Write a formula for the function obtained when the graph of[latex]\text{ }f\left(x\right)=\frac{1}{{x}^{2}}\text{ }[/latex]is shifted up 2 units and to the left 4 units.

For the following exercises, describe how the graph of the function is a transformation of the graph of the original function[latex]\text{ }f.[/latex]

8. [latex]y=f\left(x-49\right)[/latex]

9. [latex]y=f\left(x+43\right)[/latex]

10. [latex]y=f\left(x+3\right)[/latex]

11. [latex]y=f\left(x-4\right)[/latex]

12. [latex]y=f\left(x\right)+5[/latex]

13. [latex]y=f\left(x\right)+8[/latex]

14. [latex]y=f\left(x\right)-2[/latex]

15.[latex]y=f\left(x\right)-7[/latex]

16. [latex]y=f\left(x-2\right)+3[/latex]

17. [latex]y=f\left(x+4\right)-1[/latex]

For the following exercises, determine the interval(s) on which the function is increasing and decreasing.

18. [latex]f\left(x\right)=4{\left(x+1\right)}^{2}-5[/latex]

19. [latex]g\left(x\right)=5{\left(x+3\right)}^{2}-2[/latex]

20. [latex]a\left(x\right)=\sqrt[\leftroot{1}\uproot{2} ]{-x+4}[/latex]

21. [latex]k\left(x\right)=-3\sqrt[\leftroot{1}\uproot{2} ]{x}-1[/latex]

Graphical

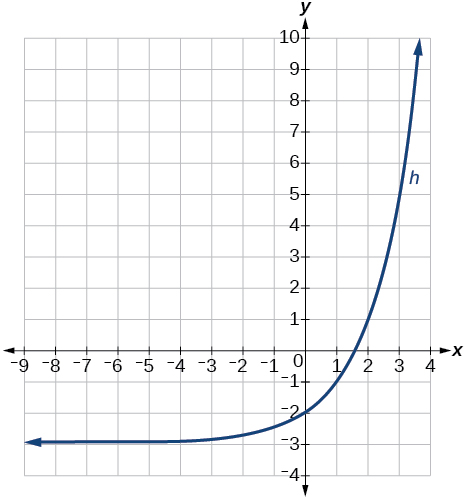
For the following exercises, use the graph of[latex]\text{ }f\left(x\right)={2}^{x}\text{ }[/latex]shown in (Figure) to sketch a graph of each transformation of[latex]\text{ }f\left(x\right).[/latex]

Graph of f(x).

Figure 33.

22. [latex]g\left(x\right)={2}^{x}+1[/latex]

23. [latex]h\left(x\right)={2}^{x}-3[/latex]

24. [latex]w\left(x\right)={2}^{x-1}[/latex]

For the following exercises, sketch a graph of the function as a transformation of the graph of one of the toolkit functions.

25. [latex]f\left(t\right)={\left(t+1\right)}^{2}-3[/latex]

26. [latex]h\left(x\right)=|x-1|+4[/latex]

27. [latex]k\left(x\right)={\left(x-2\right)}^{3}-1[/latex]

28. [latex]m\left(t\right)=3+\sqrt[\leftroot{1}\uproot{2} ]{t+2}[/latex]

Numeric

29. Tabular representations for the functions[latex]\text{ }f,\text{ }g,\text{ }[/latex]and[latex]\text{ }h\text{ }[/latex]are given below. Write[latex]\text{ }g\left(x\right)\text{ }[/latex]and[latex]\text{ }h\left(x\right)\text{ }[/latex]as transformations of[latex]\text{ }f\left(x\right).[/latex]

[latex]x[/latex] −2 −1 0 1 2
[latex]f\left(x\right)[/latex] −2 −1 −3 1 2
[latex]x[/latex] −1 0 1 2 3
[latex]g\left(x\right)[/latex] −2 −1 −3 1 2
[latex]x[/latex] −2 −1 0 1 2
[latex]h\left(x\right)[/latex] −1 0 −2 2 3

30. Tabular representations for the functions[latex]\text{ }f,\text{ }g,\text{ }[/latex]and[latex]\text{ }h\text{ }[/latex]are given below. Write[latex]\text{ }g\left(x\right)\text{ }[/latex]and[latex]\text{ }h\left(x\right)\text{ }[/latex]as transformations of[latex]\text{ }f\left(x\right).[/latex]

[latex]x[/latex] −2 −1 0 1 2
[latex]f\left(x\right)[/latex] −1 −3 4 2 1
[latex]x[/latex] −3 −2 −1 0 1
[latex]g\left(x\right)[/latex] −1 −3 4 2 1
[latex]x[/latex] −2 −1 0 1 2
[latex]h\left(x\right)[/latex] −2 −4 3 1 0

For the following exercises, write an equation for each graphed function by using transformations of the graphs of one of the toolkit functions.

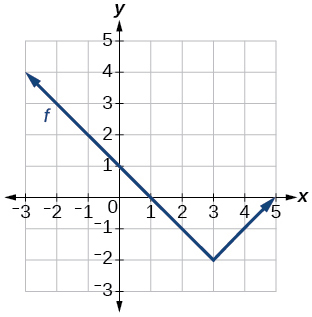
31. Graph of an absolute function.

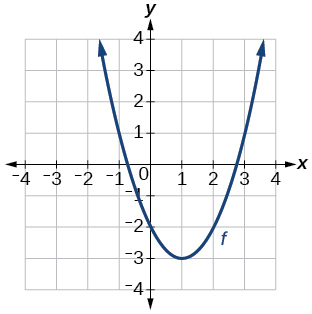
32. Graph of a parabola.
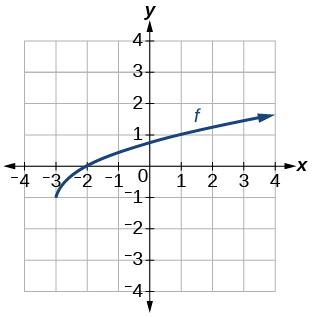
33. Graph of a square root function.

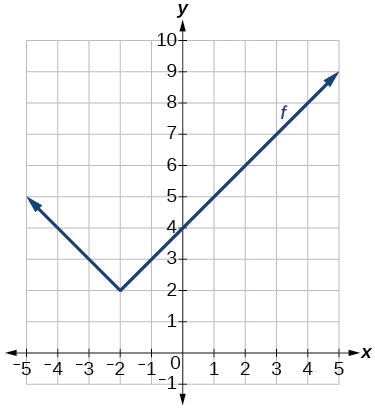
34. Graph of an absolute function.
35. Graph of a parabola

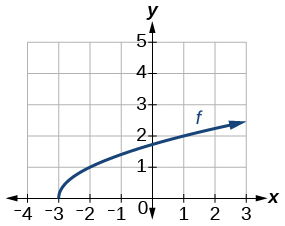
36. Graph of a square root function.
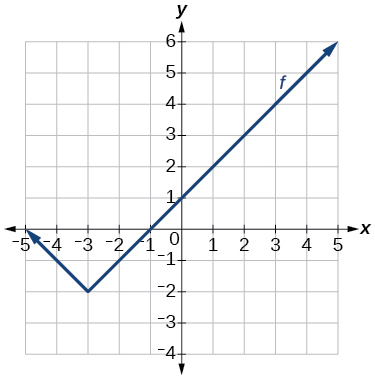
37. Graph of an absolute function.

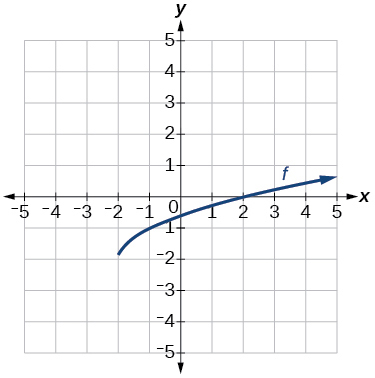
38. Graph of a square root function.

For the following exercises, use the graphs of transformations of the square root function to find a formula for each of the functions.

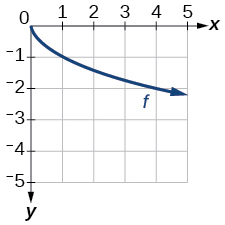
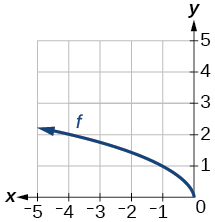
39. Graph of a square root function.

40. Graph of a square root function.

For the following exercises, use the graphs of the transformed toolkit functions to write a formula for each of the resulting functions.

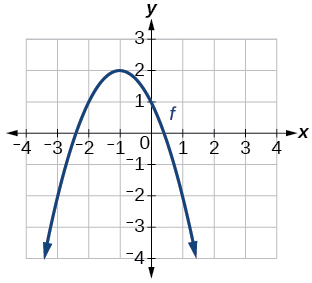
41. Graph of a parabola.

42. Graph of a cubic function.
43. Graph of a square root function.

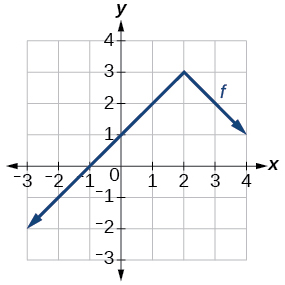
44. Graph of an absolute function.

For the following exercises, determine whether the function is odd, even, or neither.

45. [latex]f\left(x\right)=3{x}^{4}[/latex]

46. [latex]g\left(x\right)=\sqrt[\leftroot{1}\uproot{2} ]{x}[/latex]

47. [latex]h\left(x\right)=\frac{1}{x}+3x[/latex]

48. [latex]f\left(x\right)={\left(x-2\right)}^{2}[/latex]

49. [latex]g\left(x\right)=2{x}^{4}[/latex]

50. [latex]h\left(x\right)=2x-{x}^{3}[/latex]

For the following exercises, describe how the graph of each function is a transformation of the graph of the original function[latex]\text{ }f.[/latex]

53. [latex]g\left(x\right)=-f\left(x\right)[/latex]

54. [latex]g\left(x\right)=f\left(-x\right)[/latex]

55. [latex]g\left(x\right)=-f\left(-x\right)[/latex]

For the following exercises, use the graph in (Figure) to sketch the given transformations.

Graph of a polynomial.

Figure 34.

56. [latex]g\left(x\right)=f\left(x\right)-2[/latex]

57. [latex]g\left(x\right)=-f\left(x\right)[/latex]

58. [latex]g\left(x\right)=f\left(x+1\right)[/latex]

59. [latex]g\left(x\right)=f\left(x-2\right)[/latex]