Learning Outcomes
- Find the slope of a line from its graph
- Find the slope of horizontal and vertical lines
Key words
- Slope: the steepness and direction of a line
- Positive Slope: the line moves upwards from left to right
- Negative Slope: the line moves downwards from left to right
- Zero Slope: the line is horizontal
- Undefined Slope: the line is vertical
Slope
In English slope is defined as a surface that lies at an angle to the horizontal. A ski slope is a hill that skiers come down. The steeper the slope, the more difficult it is to ski. For a hiker going up a mountain, the steeper the slope, the more difficult it is to climb. Others words are also used in English to represent slope. For example, the steepness of a roof is called the pitch, while the steepness of a road is called the gradient. In math, slope is used to describe the steepness and direction of lines.
Using rubber bands on a geoboard gives a concrete way to model lines on a coordinate grid. By stretching a rubber band between two pegs on a geoboard, we can discover how to find the slope of a line. We’ll start by stretching a rubber band between two pegs to make a line as shown in the image below.

Does it look like a line?
Now we stretch one part of the rubber band straight up from the left peg and around a third peg to make the sides of a right triangle as shown in the image below. We carefully make a [latex]90^ \circ[/latex] angle around the third peg, so that one side is vertical and the other is horizontal.

To find the slope of the line, we measure the distance along the vertical and horizontal legs of the triangle. The vertical distance is called the rise and the horizontal distance is called the run, as shown below.

On our geoboard, the rise is [latex]2[/latex] units because the rubber band goes up [latex]2[/latex] spaces on the vertical leg. The rubber band goes across [latex]3[/latex] spaces on the horizontal leg, so the run is [latex]3[/latex] units.

The slope of a line is the ratio of the rise to the run. So the slope of our line is [latex]\frac{2}{3}[/latex]. In mathematics, the slope is always represented by the letter [latex]m[/latex].
Slope of a line
The slope of a line is [latex]m={\frac{\text{rise}}{\text{run}}}[/latex].
The rise measures the vertical change and the run measures the horizontal change.
example
What is the slope of the line on the geoboard shown?

Solution
Use the definition of slope.
[latex]m=\frac{\text{rise}}{\text{run}}[/latex]
Start at the left peg and make a right triangle by stretching the rubber band up and to the right to reach the second peg.
Count the rise and the run as shown.
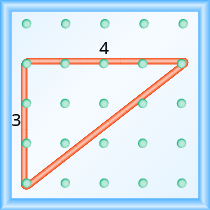
[latex]\begin{array}{cccc}\text{The rise is }3\text{ units}.\hfill & & & m={\Large\frac{3}{\text{run}}}\hfill \\ \text{The run is}4\text{ units}.\hfill & & & m={\Large\frac{3}{4}}\hfill \\ & & & \text{The slope is }{\Large\frac{3}{4}}\hfill \end{array}[/latex]
example
What is the slope of the line on the geoboard shown?

Solution
Use the definition of slope.
[latex]m=\frac{\text{rise}}{\text{run}}[/latex]
Start at the left peg and make a right triangle by stretching the rubber band to the peg on the right. This time we need to stretch the rubber band down to make the vertical leg, so the rise is negative.

[latex]\begin{array}{cccc}\text{The rise is }-1.\hfill & & & m={\Large\frac{-1}{\text{run}}}\hfill \\ \text{The run is}3.\hfill & & & m={\Large\frac{-1}{3}}\hfill \\ & & & m=-{\Large\frac{1}{3}}\hfill \\ & & & \text{The slope is }-{\Large\frac{1}{3}}\hfill \end{array}[/latex]
try it
Finding the Slope of a Line from its Graph
By just looking at the graph of a line, we can learn some things about its slope, especially relative to other lines graphed on the same coordinate plane. Consider the graphs of the three lines shown below:
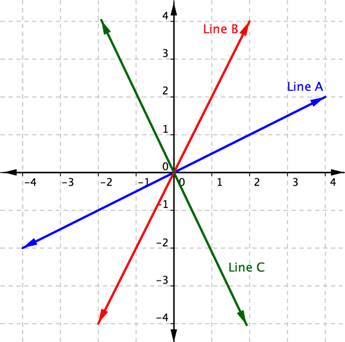
First, let’s look at lines A and B. Line B is steeper than line A, so Line B has a greater slope than line A.
Next, notice that lines A and B slant up as we move from left to right. We say these two lines have a positive slope. Line C slants down from left to right. Line C has a negative slope. Using two of the points on the line, we can find the slope of the line by finding the rise and the run. The vertical change between two points is called the rise, and the horizontal change is called the run. The slope equals the rise divided by the run: [latex]\displaystyle \text{Slope }=\frac{\text{rise}}{\text{run}}[/latex].
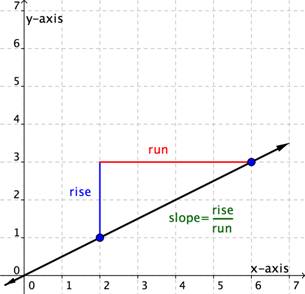
You can determine the slope of a line from its graph by looking at the rise and run. (Notice the similarity between the image above and the Geoboard examples we looked at earlier.) One characteristic of a line is that its slope is constant all the way along it. So, we can choose any 2 points along the graph of the line to figure out the slope. Let’s look at an example.
Example
Use the graph to find the slope of the line.
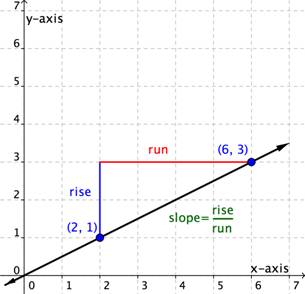
Solution
Start from a point on the line, such as [latex](2,1)[/latex] and move vertically until in line with another point on the line, such as [latex](6,3)[/latex]. The rise is 2 units. It is positive as you moved up.
| [latex]\text{rise}=2[/latex] | Start from a point on the line, such as [latex](2,1)[/latex] and move vertically until in line with another point on the line, such as [latex](6,3)[/latex]. The rise is 2 units. It is positive as you moved up. |
| [latex]\text{run}=4[/latex] | Next, move horizontally to the point [latex](6,3)[/latex]. Count the number of units. The run is 4 units. It is positive as you moved to the right. |
| [latex]\displaystyle \text{Slope}=\frac{2}{4}=\frac{1}{2}[/latex] | [latex]\displaystyle \text{Slope }=\frac{\text{rise}}{\text{run}}[/latex] |
Answer
[latex]m=\frac{1}{2}[/latex]
This line will have a slope of [latex]\displaystyle \frac{1}{2}[/latex] no matter which two points we pick on the line. Try measuring the slope from the origin, [latex](0,0)[/latex], to the point [latex](6,3)[/latex]. The [latex]\text{rise}=3[/latex] and the [latex]\text{run}=6[/latex]. The slope is [latex]\displaystyle \frac{\text{rise}}{\text{run}}=\frac{3}{6}=\frac{1}{2}[/latex]. It is the same!
Let’s look at another example.
Example
Use the graph to find the slope of the two lines.
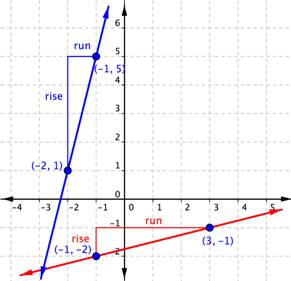
Solution
Notice that both of these lines have positive slopes, so we expect the answers to be positive.
| Blue line | |
|---|---|
| [latex]\text{rise}=4[/latex] | Start with the blue line, going from point [latex](-2,1)[/latex] to point [latex](-1,5)[/latex]. This line has a rise of 4 units up, so it is positive. |
| [latex]\text{run}=1[/latex] | Run is [latex]1[/latex] unit to the right, so it is positive. |
| [latex]\displaystyle \text{Slope }=\frac{4}{1}=4[/latex] | Substitute the values for the rise and run in the formula [latex]\displaystyle \text{Slope }\frac{\text{rise}}{\text{run}}[/latex]. |
| Red line | |
| [latex]\text{rise}=1[/latex] | The red line, going from point [latex](-1,-2)[/latex] to point [latex](3,-1)[/latex] has a rise of 1 unit. |
| [latex]\text{run}=4[/latex] | The red line has a run of 4 units. |
| [latex]\displaystyle \text{Slope }=\frac{1}{4}[/latex] | Substitute the values for the rise and run into the formula [latex]\displaystyle \text{Slope }\frac{\text{rise}}{\text{run}}[/latex]. |
Answer
The slope of the blue line is [latex]4[/latex] and the slope of the red line is [latex]\frac{1}{4}[/latex].
As we look at the two lines, we can see that the blue line is steeper than the red line. It makes sense the value of the slope of the blue line, [latex]4[/latex], is greater than the value of the slope of the red line, [latex]\displaystyle \frac{1}{4}[/latex]. The greater the slope, the steeper the line.
Watch the following video to see how to determine the slope of a line.
Positive and Negative Slopes
Direction is important when it comes to determining slope. It’s important to pay attention to whether we are moving up, down, left, or right; that is, if we are moving in a positive or negative direction. If we go up to get to our second point, the rise is positive. If we go down to get to our second point, the rise is negative. If we go right to get to our second point, the run is positive. If we go left to get to our second point, the run is negative.
Example
Find the slope of the line graphed below.
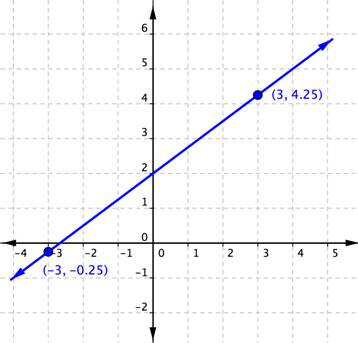
Solution
| [latex]\text{rise}=4.5[/latex] | Start at [latex](-3,-0.25)[/latex] and rise [latex]4.5[/latex]. This means moving 4.5 units in a positive direction. |
| [latex]\text{run}=6[/latex] | From there, run [latex]6[/latex] units in a positive direction to [latex](3,4.25)[/latex]. |
| [latex]\displaystyle \text{Slope}=\frac{4.5}{6}=0.75[/latex] | [latex]\displaystyle \text{Slope}=\frac{\text{rise}}{\text{run}}[/latex] |
Answer
The slope of the line is [latex]0.75[/latex].
The next example shows a line with a negative slope.
Example
Find the slope of the line graphed below.
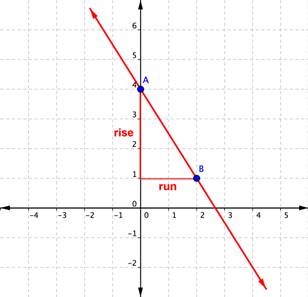
Solution
| [latex]\text{rise}=-3[/latex] | Start at Point A, [latex](0,4)[/latex] and rise [latex]−3[/latex]. This means movin g[latex]3[/latex] units in a negative direction. |
| [latex]\text{run}=2[/latex] | From there, run [latex]2[/latex] units in a positive direction to Point B [latex](2,1)[/latex]. |
| [latex]\displaystyle \text{Slope}=\frac{\text{rise}}{\text{run}}[/latex] | [latex]\displaystyle \text{Slope}=\frac{\text{rise}}{\text{run}}[/latex] |
Answer
The slope of the line is [latex]-\frac{3}{2}[/latex].
NOTE:
we could have found the slope by starting at point B, running [latex]{-2}[/latex], and then rising [latex]+3[/latex] to arrive at point A. The result is still a slope of [latex]\displaystyle\frac{\text{rise}}{\text{run}}=\frac{+3}{-2}=-\frac{3}{2}[/latex].
In all of the previous examples of finding the slope of a line, we were given two points. Now we will look at some examples where we are not automatically given two points on the line.
To find the slope, we must count out the rise and the run. But where do we start?
We locate any two points on the line. We try to choose points with coordinates that are integers to make our calculations easier. We then start with the point on the left and sketch a right triangle, so we can count the rise and run.
example
Find the slope of the line shown:
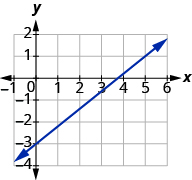
Solution
Locate two points on the graph, choosing points whose coordinates are integers. We will use [latex]\left(0,-3\right)[/latex] and [latex]\left(5,1\right)[/latex].
Starting with the point on the left, [latex]\left(0,-3\right)[/latex], sketch a right triangle, going from the first point to the second point, [latex]\left(5,1\right)[/latex].
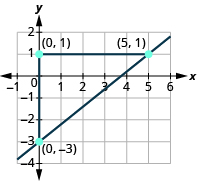 |
|
| Count the rise on the vertical leg of the triangle. | The rise is [latex]4[/latex] units. |
| Count the run on the horizontal leg. | The run is [latex]5[/latex] units. |
| Use the slope formula. | [latex]m={\Large\frac{\text{rise}}{\text{run}}}[/latex] |
| Substitute the values of the rise and run. | [latex]m={\Large\frac{4}{5}}[/latex] |
| The slope of the line is [latex]{\Large\frac{4}{5}}[/latex] . |
Notice that the slope is positive since the line slants upward from left to right.
try it
Find the slope OF A LINE from ITS graph
- Locate two points on the line.
- Starting with the point on the left, sketch a right triangle, going from the first point to the second point.
- Count the rise and the run on the legs of the triangle.
- Take the ratio of rise to run to find the slope. [latex]m={\Large\frac{\text{rise}}{\text{run}}}[/latex]
example
Find the slope of the line shown:

Solution
Locate two points on the graph. Look for points with coordinates that are integers. We can choose any points, but we will use [latex](0, 5)[/latex] and [latex](3, 3)[/latex]. Starting with the point on the left, sketch a right triangle, going from the first point to the second point.
 |
|
| Count the rise – it is negative. | The rise is [latex]−2[/latex]. |
| Count the run. | The run is [latex]3[/latex]. |
| Use the slope formula. | [latex]m=\Large\frac{\text{rise}}{\text{run}}[/latex] |
| Substitute the values of the rise and run. | [latex]m={\Large\frac{-2}{3}}[/latex] |
| Simplify. | [latex]m=-{\Large\frac{2}{3}}[/latex] |
| The slope of the line is [latex]-{\Large\frac{2}{3}}[/latex]. |
Notice that the slope is negative since the line slants downward from left to right.
What if we had chosen different points? Let’s find the slope of the line again, this time using different points. We will use the points [latex]\left(-3,7\right)[/latex] and [latex]\left(6,1\right)[/latex].

Starting at [latex]\left(-3,7\right)[/latex], sketch a right triangle to [latex]\left(6,1\right)[/latex].

| Count the rise. | The rise is [latex]−6[/latex]. |
| Count the run. | The run is [latex]9[/latex]. |
| Use the slope formula. | [latex]m=\frac{\text{rise}}{\text{run}}[/latex] |
| Substitute the values of the rise and run. | [latex]m={\Large\frac{-6}{9}}[/latex] |
| Simplify the fraction. | [latex]m=-{\Large\frac{2}{3}}[/latex] |
| The slope of the line is [latex]-{\Large\frac{2}{3}}[/latex]. |
It does not matter which points we use—the slope of the line is always the same. The slope of a line is constant!
try it
The lines in the previous examples had [latex]y[/latex] -intercepts with integer values, so it was convenient to use the [latex]y[/latex]-intercept as one of the points we used to find the slope. In the next example, the [latex]y[/latex]-intercept is a fraction. The calculations are easier if we use two points with integer coordinates.
example
Find the slope of the line shown:

Solution
| Locate two points on the graph whose coordinates are integers. | [latex]\left(2,3\right)[/latex] and [latex]\left(7,6\right)[/latex] |
| Which point is on the left? | [latex]\left(2,3\right)[/latex] |
| Starting at [latex]\left(2,3\right)[/latex] , sketch a right angle to [latex]\left(7,6\right)[/latex] as shown below. |
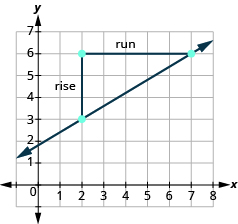 |
|
| Count the rise. | The rise is [latex]3[/latex]. |
| Count the run. | The run is [latex]5[/latex]. |
| Use the slope formula. | [latex]m=\frac{\text{rise}}{\text{run}}[/latex] |
| Substitute the values of the rise and run. | [latex]m={\frac{3}{5}}[/latex] |
| The slope of the line is [latex]{\Large\frac{3}{5}}[/latex]. |
try it
The following videos show examples of how to find the slope of a line given a graph.
Horizontal and Vertical Lines
Do you remember what was special about horizontal and vertical lines? Their equations had just one variable.
- horizontal line [latex]y=b[/latex]; all the [latex]y[/latex] -coordinates are the same.
- vertical line [latex]x=a[/latex]; all the [latex]x[/latex] -coordinates are the same.
So how do we find the slope of the horizontal line [latex]y=4?[/latex] One approach would be to graph the horizontal line, find two points on it, and count the rise and the run. Let’s see what happens. We’ll use the two points [latex]\left(0,4\right)[/latex] and [latex]\left(3,4\right)[/latex] to count the rise and run.
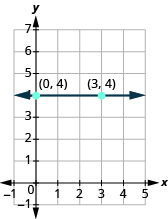
| What is the rise? | The rise is [latex]0[/latex]. |
| What is the run? | The run is [latex]3[/latex]. |
| What is the slope? | [latex]m=\frac{\text{rise}}{\text{run}}[/latex] |
| [latex]m={\Large\frac{0}{3}}[/latex] | |
| [latex]m=0[/latex] |
The slope of the horizontal line [latex]y=4[/latex] is [latex]0[/latex].
All horizontal lines have slope [latex]0[/latex] . When the [latex]y[/latex]-coordinates are the same, the rise is [latex]0[/latex] .
Slope of a Horizontal Line
The slope of a horizontal line, [latex]y=b[/latex], is [latex]0[/latex].
Now we’ll consider a vertical line, such as the line [latex]x=3[/latex], shown below. We’ll use the two points [latex]\left(3,0\right)[/latex] and [latex]\left(3,2\right)[/latex] to count the rise and run.
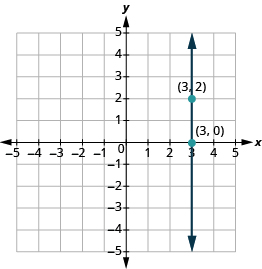
| What is the rise? | The rise is [latex]2[/latex]. |
| What is the run? | The run is [latex]0[/latex]. |
| What is the slope? | [latex]m=\frac{\text{rise}}{\text{run}}[/latex] |
| [latex]m={\Large\frac{2}{0}}[/latex] |
But we can’t divide by [latex]0[/latex]. Division by [latex]0[/latex] is undefined. So we say that the slope of the vertical line [latex]x=3[/latex] is undefined. The slope of all vertical lines is undefined, because the run is [latex]0[/latex].
Slope of a Vertical Line
The slope of a vertical line, [latex]x=a[/latex], is undefined.
example
Find the slope of each line:
1. [latex]x=8[/latex]
2. [latex]y=-5[/latex]
Solution
1. [latex]x=8[/latex]
This is a vertical line, so its slope is undefined.
2. [latex]y=-5[/latex]
This is a horizontal line, so its slope is [latex]0[/latex].
try it
Slopes of Lines
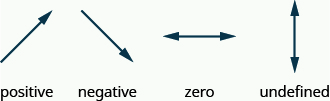
The following example shows how to determine the slope of horizontal and vertical lines.
Candela Citations
- Determine the Slope of a Line From a Graph (No Formula). Authored by: Mathispower4u. Located at: https://youtu.be/29BpBqsiE5w. License: CC BY: Attribution
