You learn as a child how to subtract numbers through everyday experiences. For example, if you have 10 animal cookies and eat 6 of them, you will have 4 animal cookies left.

Real-life experiences serve as models for subtracting positive numbers, and in some cases, such as temperature, for adding negative as well as positive numbers. But it is difficult to relate subtracting negative numbers to common life experiences. Most people do not have an intuitive understanding of subtraction when negative numbers are involved. Math teachers use several different models to explain subtracting negative numbers.

We will continue to use counters to model subtraction. Remember, the purple counters represent positive numbers and the orange counters represent negative numbers.

Perhaps when you were younger, you read [latex]5 - 3[/latex] as five take away three. When we use counters, we can think of subtraction the same way.

We will model four subtraction scenarios using the numbers [latex]5[/latex] and [latex]3[/latex].

• [latex]5 - 3[/latex]
• [latex]- 5-\left(-3\right)[/latex]
• [latex]-5 - 3[/latex]
• [latex]5-\left(-3\right)[/latex]

example

Model: [latex]5 - 3[/latex].

Solution:

Interpret the expression.	[latex]5 - 3[/latex] means [latex]5[/latex] take away [latex]3[/latex] .
Model the first number. Start with [latex]5[/latex] positives.
Take away the second number. So take away [latex]3[/latex] positives.
Find the counters that are left.
	[latex]5 - 3=2[/latex] The difference between [latex]5[/latex] and [latex]3[/latex] is [latex]2[/latex] .

Now you can try a similar problem.

try it

Model the expression:

[latex]6 - 4[/latex]

Show Solution

 

 

 

 

[latex]2[/latex]

 

Model the expression:

[latex]7 - 4[/latex]

Show Solution

 

 

 

 

[latex]3[/latex]

In the previous example we subtracted [latex]3[/latex] positives from positive [latex]5[/latex]. Now we will subtract [latex]3[/latex] negatives from negative [latex]5[/latex]. Compare the results of this example to the previous example after you read through it.

example

Model: [latex]-5-\left(-3\right)[/latex]

Show Solution

Solution:

Interpret the expression.	[latex]-5-\left(-3\right)[/latex] means [latex]-5[/latex] take away [latex]-3[/latex] .
Model the first number. Start with [latex]5[/latex] negatives.
Take away the second number. So take away [latex]3[/latex] negatives.
Find the number of counters that are left.
	[latex]-5-\left(-3\right)=-2[/latex] The difference between [latex]-5[/latex] and [latex]-3[/latex] is [latex]-2[/latex] .

You can try a similar problem.

try it

Model the expression:

[latex]-6-\left(-4\right)[/latex]

Show Solution

 

 

 

 

[latex]−2[/latex]

 

Model the expression:

[latex]-7-\left(-4\right)[/latex]

Show Solution

 

 

 

 

[latex]−3[/latex]

Notice that the previous two examples are very much alike.

• First, we subtracted [latex]3[/latex] positives from [latex]5[/latex] positives to get [latex]2[/latex] positives.
• Then we subtracted [latex]3[/latex] negatives from [latex]5[/latex] negatives to get [latex]2[/latex] negatives.

Each example used counters of only one color, and the “take away” model of subtraction was easy to apply.

Now let’s see what happens when we subtract one positive and one negative number. We will need to use both positive and negative counters and sometimes some neutral pairs, too. Adding a neutral pair does not change the value.

example

Model: [latex]-5 - 3[/latex]

Solution:

Interpret the expression.	[latex]-5 - 3[/latex] means [latex]-5[/latex] take away [latex]3[/latex] .
Model the first number. Start with [latex]5[/latex] negatives.
Take away the second number. So we need to take away [latex]3[/latex] positives.
But there are no positives to take away. Add neutral pairs until you have [latex]3[/latex] positives.
Now take away [latex]3[/latex] positives.
Count the number of counters that are left.
	[latex]-5 - 3=-8[/latex] The difference of [latex]-5[/latex] and [latex]3[/latex] is [latex]-8[/latex] .

 

Now you can try a similar problem.

try it

Model the expression:

[latex]-6 - 4[/latex]

Show Solution

 

 

 

 

 

 

[latex]−10[/latex]

 

Model the expression:

[latex]-7 - 4[/latex]

Show Solution

 

 

 

 

 

 

[latex]−11[/latex]

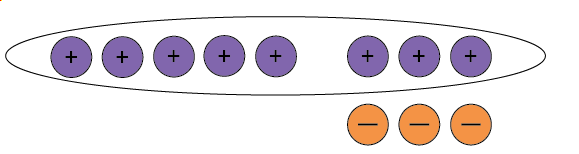
Now we will subtract a negative number from a positive number. Think of this as taking away the negative.

example

Model: [latex]5-\left(-3\right)[/latex]

Show Solution

Solution:

Interpret the expression.	[latex]5-\left(-3\right)[/latex] means [latex]5[/latex] take away [latex]-3[/latex] .
Model the first number. Start with [latex]5[/latex] positives.
Take away the second number, so take away [latex]3[/latex] negatives.
But there are no negatives to take away. Add neutral pairs until you have [latex]3[/latex] negatives.
Then take away [latex]3[/latex] negatives.
Count the number of counters that are left.
	The difference of [latex]5[/latex] and [latex]-3[/latex] is [latex]8[/latex] . [latex]5-\left(-3\right)=8[/latex]

Now you can try a similar problem.

try it

Model the expression:

[latex]6-\left(-4\right)[/latex]

Show Solution

[latex]10[/latex]

 

Model the expression:
[latex]7-\left(-4\right)[/latex]

Show Solution

[latex]11[/latex]

Now we will do an example that summarizes the situations above, with different numbers. Recall the different scenarios:

• subtracting a positive number from a positive number
• subtracting a positive number from a negative number
• subtracting a negative number from a positive number
• subtracting a negative number from a negative number

example

Model each subtraction.

1. [latex]8 − 2[/latex]
2. [latex]−5 − 4[/latex]
3. [latex]6 − (−6)[/latex]
4. [latex]−8 − (−3)[/latex]

Show Solution

1.
	[latex]8 - 2[/latex] This means [latex]8[/latex] take away [latex]2[/latex] .
Start with [latex]8[/latex] positives.
Take away [latex]2[/latex] positives.
How many are left?	[latex]6[/latex]
	[latex]8 - 2=6[/latex]

2.
	[latex]-5 - 4[/latex] This means [latex]-5[/latex] take away [latex]4[/latex] .
Start with [latex]5[/latex] negatives.
You need to take away [latex]4[/latex] positives. Add [latex]4[/latex] neutral pairs to get [latex]4[/latex] positives.
Take away [latex]4[/latex] positives.
How many are left?
	[latex]-9[/latex]
	[latex]-5 - 4=-9[/latex]

3.
	[latex]6-\left(-6\right)[/latex] This means [latex]6[/latex] take away [latex]-6[/latex] .
Start with [latex]6[/latex] positives.
Add [latex]6[/latex] neutrals to get [latex]6[/latex] negatives to take away.
Remove [latex]6[/latex] negatives.
How many are left?
	[latex]12[/latex]
	[latex]6-\left(-6\right)=12[/latex]

4.
	[latex]-8-\left(-3\right)[/latex] This means [latex]-8[/latex] take away [latex]-3[/latex] .
Start with [latex]8[/latex] negatives.
Take away [latex]3[/latex] negatives.
How many are left?
	[latex]-5[/latex]
	[latex]-8-\left(-3\right)=-5[/latex]

Now you can try a similar problem.

try it

Model each subtraction.

1. [latex]7 - (-8)[/latex]
2. [latex]-7 - (-2)[/latex]
3. [latex]4 - 1[/latex]
4. [latex]-6 - 8[/latex]

Show Solution

1. [latex]15[/latex]

2. [latex]-5[/latex]

 

 

 

 

3. [latex]3[/latex]

 

 

 

 

4. [latex]-14[/latex]

 

Model each subtraction.

1. [latex]4 - (-6)[/latex]
2. [latex]-8 - (-1)[/latex]
3. [latex]7 - 3[/latex]
4. [latex]-4 - 2[/latex]

Show Solution

1. [latex]10[/latex]

2. [latex]-7[/latex]

 

 

 

 

3. [latex]4[/latex]

 

 

 

 

 

4. [latex]-6[/latex]

 

 

 

 

 

Each of the examples so far have been carefully constructed so that the sign of the answer matched the sign of the first number in the expression.  For example, in  [latex]−5 − 4[/latex], the result is [latex]-9[/latex], which is the same sign as [latex]-5[/latex]. Now we will see subtraction where the sign of the result is different from the starting number.

example

Model each subtraction expression:

1. [latex]2 - 8[/latex]
2. [latex]-3-\left(-8\right)[/latex]

Show Solution

Solution

1. We start with [latex]2[/latex] positives.
We need to take away [latex]8[/latex] positives, but we have only [latex]2[/latex].
Add neutral pairs until there are [latex]8[/latex] positives to take away.
Then take away [latex]8[/latex] positives.
Find the number of counters that are left. There are [latex]6[/latex] negatives.
	[latex]2 - 8=-6[/latex]

2. We start with [latex]3[/latex] negatives.
We need to take away [latex]8[/latex] negatives, but we have only [latex]3[/latex].
Add neutral pairs until there are [latex]8[/latex] negatives to take away.
Then take away the [latex]8[/latex] negatives.
Find the number of counters that are left. There are [latex]5[/latex] positives.
	[latex]-3-\left(-8\right)=5[/latex]

Now you can try a similar problem.

try it

Model each subtraction expression.

1. [latex]7 - 9[/latex]
2. [latex]-5-9[/latex]

Show Solution

1.

 

 

 

 

 

[latex]−2[/latex]

2.

 

 

 

 

 

 

[latex]4[/latex]

 

Model each subtraction expression.

1. [latex]5 - 8[/latex]
2. [latex]-7-\left(-10\right)[/latex]

Show Solution

1.

 

 

 

 

 

 

[latex]−3[/latex]

2.

 

 

 

 

 

 

[latex]3[/latex]

When you subtract two integers, there are two possibilities, either the result will have a different sign from the starting number, or it will have the same sign.

Watch the video below to see more examples of modeling integer subtraction with color counters.