Why Illusions?

Psychologists have analyzed perceptual systems for more than a century. Vision and hearing have received the most attention by far, but other perceptual systems, like those for smell taste movement, balance, touch, and pain, have also been studied extensively. Perception scientists use a variety of approaches to study these systems—they design experiments, study neurological patients with damaged brain regions, and create perceptual illusions that toy with the brain’s efforts to interpret the sensory world.

Creation and testing of perceptual illusions has been a fruitful approach to the study of perception—particularly visual perception—since the early days of psychology. People often think that visual illusions are simply amusing tricks that provide us with entertainment. Many illusions are fun to experience, but perception scientists create illusions based on their understanding of the perceptual system. Once they have created a successful illusion, the scientist can explore what people experience, what parts of the brain are involved in interpretation of the illusion, and what variables increase or diminish the strength of the illusion. Scientists are not alone in this interest. Visual artists have discovered and used many illusion-producing principles for centuries, allowing them to create the experience of depth, movement, light and shadow, and relative size on two-dimensional canvases.

Depth Illusions

When we look at the world, we are not very good at detecting the absolute qualities of things—their exact size or color or shape. What we are very good at is judging objects in the context of other objects and conditions. Let’s take a look at a few illusions to see how they are based on insights about our perception. Look at Figure 2 below. Which of the two horizontal yellow lines looks wider, the top one or the bottom one?

Most people experience the top line as wider. They are both exactly the same length. This experience is called the Ponzo illusion. Even though you know that the lines are the same length, it is difficult to see them as identical. Our perceptual system takes the context into account, here using the converging “railroad tracks” to produce an experience of depth. Then, using some impressive mental geometry, our brain adjusts the experienced length of the top line to be consistent with the size it would have if it were that far away: if two lines are the same length on my retina, but different distances from me, the more distant line must be in reality longer. You experience a world that “makes sense” rather than a world that reflects the actual objects in front of you.

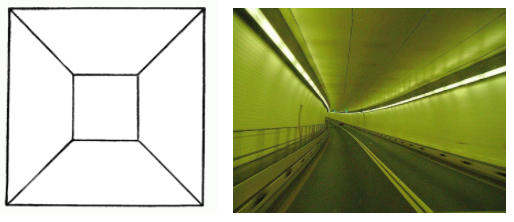
There are many depth illusions. It is difficult to see the drawing on the left below as a two-dimensional figure. The converging lines and smaller square at the center seem to coax our perceptual systems into seeing depth, even though we know that the drawing is flat. This urge to see depth is probably so strong because our ability to use two-dimensional information to infer a three dimensional world is essential for allowing us to operate in the world. The picture on the right below is a driving tunnel, something you would need to process at high speed if you were in a car going through it. Your quick and detailed use of converging lines and other cues allows you to make sense of this 3-D world.

Light and Size Illusions

Depth is not the only quality in the world that shows how we adjust what we experience to fit the surrounding world. Look at the two gray squares in the figure below. Which one looks darker?

Most people experience the square on the right as the darker of the two gray squares. You’ve probably already guessed that the squares are actually identical in shade, but the surrounding area—black on the left and white on the right—influence how our perceptual systems interpret the gray area. In this case, the greater difference in shading between the white surrounding area and the gray square on the right results in the experience of a darker square.

Here is another example below. The two triangular figures are identical in shade, but the triangle on the left looks lighter against the dark background of the cross when compared to the triangle in the white area on the right.

Our visual systems work with more than simple contrast. They also use our knowledge of how the world works to adjust our perceptual experience. Look at the checkerboard below. There are two squares with letters in them, one marked “A” and the other “B”. Which one of those two squares is darker?

This seems like an easy comparison, but the truth is that squares A and B are identical in shade. Our perceptual system adjusts our experience by taking some visual information into account. First, “A” is one of the “dark squares” and “B” is a “light square” if we take the checkerboard pattern into account. Perhaps even more impressive, our visual systems notice that “B” is in a shadow. Object in a shadow appear darker, so our experience is adjusted to take account of effect of the shadow, resulting in perceiving square B as being lighter than square A, which sits in the bright light. And if you really don’t believe your eyes, take a look at a video showing the same color tiles here.

One final illusion takes us back to adjustment for size. Look at the two sets of circles below. Your task is to adjust the center circle on the left so it is the same actual size as the center circle on the right. The surrounding circles will not change in size, though the right of circles will expand to accommodate the size of the center circle. Use the slide bar with the label “Size of left circle” to make your adjustments. When you are satisfied with your adjustment, check your accuracy by clicking on the “Verify Diameter” button. Click “Reset” to try again.

This illusion is called the Ebbinghaus illusion, created by Hermann Ebbinghaus, one of the early founders of experimental psychology. It is shown again below.

In this version of the illusion, most people see the circle on the right as larger than the one on the left. The two orange circles are exactly the same size. The Ebbinghaus illusion again illustrates the tendency of our perceptual systems to adjust our experience of the world to the surrounding context.

How do you think a psychologist might use the Ebbinghaus illusion to learn about mental processes or behavior (shown again below)? Read on to see an actual example from a psychologist at Colorado State University.

Ebbinghaus in the Real World

Imagine that you are in a golf competition in which you are putting against someone with the same experience and skill that you have. There is one problem: Your opponent gets to putt into a hole that is 10% larger than the hole you have to use. You’d probably think that the competition was unfairly biased against you.

Now imagine a somewhat different situation. You and your opponent are about equal in ability and the holes you are using are the same size, but the hole that your opponent is using looks 10% larger than the one you are using. Would your opponent have an unfair advantage now?

If you read the earlier section on the Ebbinghaus effect, you have an idea how psychologists could exploit your perceptual system (and your opponent’s) to test this very question. Psychologist Jessica Witt and her colleagues Sally Linkenauger and Dennis Proffitt recruited research participants with no unusual golf experience to participate in a putting task. They competed against themselves rather than against another person.

The experimenters made the task challenging by using a hole with a 2-inch diameter, which is about half the diameter of the hole you will find on a golf course. An overhead projector mounted on the ceiling of their lab allowed them to project Ebbinghaus’s circles around the putting hole. Some participants saw the putting hole surrounded by circles that were smaller than the hole in the center; the other half saw surrounding black circles that were larger.

Participants putted from about 11½ feet away.  They took 10 putts in one condition, and then 10 in the other condition. Half of the participants putted with the large surrounding circles first and half saw the small surrounding circles first. This procedure is called counterbalancing. If there is any advantage (e.g., getting better over time with practice) or disadvantage (e.g., getting tired of putting), counterbalancing assures that both conditions are equally exposed to the positive or negative effects of which task goes first or second. Failure to take account of this type of problem means that you may have a confounding variable—practice or fatigue—that influences performance. A confounding variable is something that could influence performance, but is not part of the study. We try to control (that is, neutralize) potentially confounding variables so they cannot be the cause of performance differences. So, for instance, if everyone did the large surrounding circles condition first and then the small surrounding circles, then differences in performance could be due to order of conditions (leading to practice or fatigue effects) rather than the size of the surrounding circles. By counterbalancing, we don’t get rid of the effects of practice or fatigue for any particular person, but—across all the participants—practice or fatigue should affect both conditions (both types of Ebbinghaus circles) equally.

The experimenters wanted to know two things. First, did they actually produce the Ebbinghaus illusion? Remember: there is no guarantee that people see or think the way your theory says they should. So just before the participant started putting in a particular condition, he or she drew a circle using a computerized drawing tool, attempting to match the exact size of the putting hole. This is better than simply asking, “do you see the illusion?” The drawing task attempts to directly measure what they perceive.

Second, the experimenters wanted to see if the perceived size of the hole influenced putting accuracy. They recorded the success or failure of each putt. Each participant could get a score of 0 to 10 successful putts in each condition.

Methods Summary

Recap the steps you’ve read about thus far:

1. The participant practices putting to get used to the task.
2. The participant completes the first condition (large surrounding circles for half of the participants and small surrounding circles for the other half).
   • The participant draws a circle corresponding to his or her estimation of the actual size of the putting hole. This allows the experimenters to determine if the Ebbinghaus effect actually occurred.
   • The participant putts 10 times in this condition.
3. Participant completes the second condition (whichever condition they have not yet done).
   • The participant draws a circle corresponding to his or her estimation of the actual size of the putting hole.
   • The participant putts 10 times in this condition.

Now see if you can guess the results of this study.

This is not the only experiment that has used a sports context to study the effects of illusions. Other experiments have shown that people hit softballs better when the balls are perceived as larger. People score higher in darts when the board appears larger. Athletes kick field goals and return tennis balls more successfully when the goal posts or tennis balls appear larger. In all of these studies, the balls or boards or goal posts were not actually larger, but they were perceived as larger because the experimenters created illusions. Skilled athletes often report that targets appear larger or time slows down when they are “in the zone”, as if practice and skill create their own perceptual illusions that increase confidence and make difficult challenges feel easier.

A Final Note: Science Doesn’t Always Produce Simple Results

Professor Witt’s study had interesting results; however, they weren’t quite as simple as we have made them seem. The researchers actually had two different hole sizes: 2 inches and 4 inches. The Ebbinghaus circles were adjusted to be relatively larger or smaller than the putting hole.

The Ebbinghaus illusion worked for the smaller (2 inch) putting holes, but not for the larger (4 inch) putting holes. In other words, when people drew the circles as they perceived them (the “manipulation check” dependent variable), they drew different sized circles for the 2 inch holes (the Ebbinghaus illusion), but the same size circles for the 4 inch holes (no Ebbinghaus illusion).

For the larger (4 inch) putting holes, putting accuracy was the same for the two different conditions. This didn’t bother the experimenters, because—as we have already noted—the participants did not experience the Ebbinghaus illusion with the larger holes. If the holes were perceived as the same, then self-confidence should not have been affected and, in turn, putting should not have been better in one condition than the other.

In the research paper, the experimenters suggest a few technical reasons that the larger hole might not have produced the Ebbinghaus illusion, but they admit that they have no definitive explanation. That’s okay. Science often yields messy results—and these can be the basis for new experiments and sometimes for really interesting discoveries. The world is not as simple as our theories try to make it seem. Happily, in science, as in many aspects of life, you learn more from your failures than your successes, so good scientists don’t try to hide from results they don’t expect.